The SECOND Most Important Equation in Quantum Mechanics: Eigenvalue Equation Explained for BEGINNERS
Summary
TLDRThis video explores the second most important equation in quantum mechanics, the eigenvalue equation. It starts by explaining the mathematical concept of eigenvectors and eigenvalues using matrices and vectors. The video then connects these concepts to quantum mechanics, showing how quantum states can be treated as vectors and how measurements act like matrices. The eigenvalue equation helps us understand possible measurement results, particularly for systems in an eigenstate. The video also touches on superposition, quantum measurements, and links to the Schrödinger equation, providing a clear and engaging explanation of key quantum principles.
Takeaways
- 🔬 The Schrödinger equation is considered the most important equation in quantum mechanics, governing the evolution of quantum states over time.
- 📊 The eigenvalue equation is regarded as the second most important equation in quantum mechanics, relating to how certain vectors are transformed by matrices.
- ➡️ Mathematically, vectors represent quantities with size and direction, while matrices can transform these vectors.
- 🔗 An eigenvector is a special vector that, when acted upon by a matrix, only changes in magnitude (stretched or shrunk), not direction. The eigenvalue represents this magnitude change.
- 🌀 In quantum mechanics, the state of a quantum system, such as an electron's spin, can be represented as a vector in an abstract mathematical space.
- 🔍 Measurement in quantum mechanics can be treated mathematically as a matrix applied to a quantum state, leading to possible eigenstates and eigenvalues as measurement results.
- 💡 When a quantum system is in an eigenstate, measuring it does not change the state, and the measurement result is the eigenvalue.
- 🎲 A quantum system can be in a superposition of multiple states, and measurements on non-eigenstates lead to a random collapse into one of the possible eigenstates.
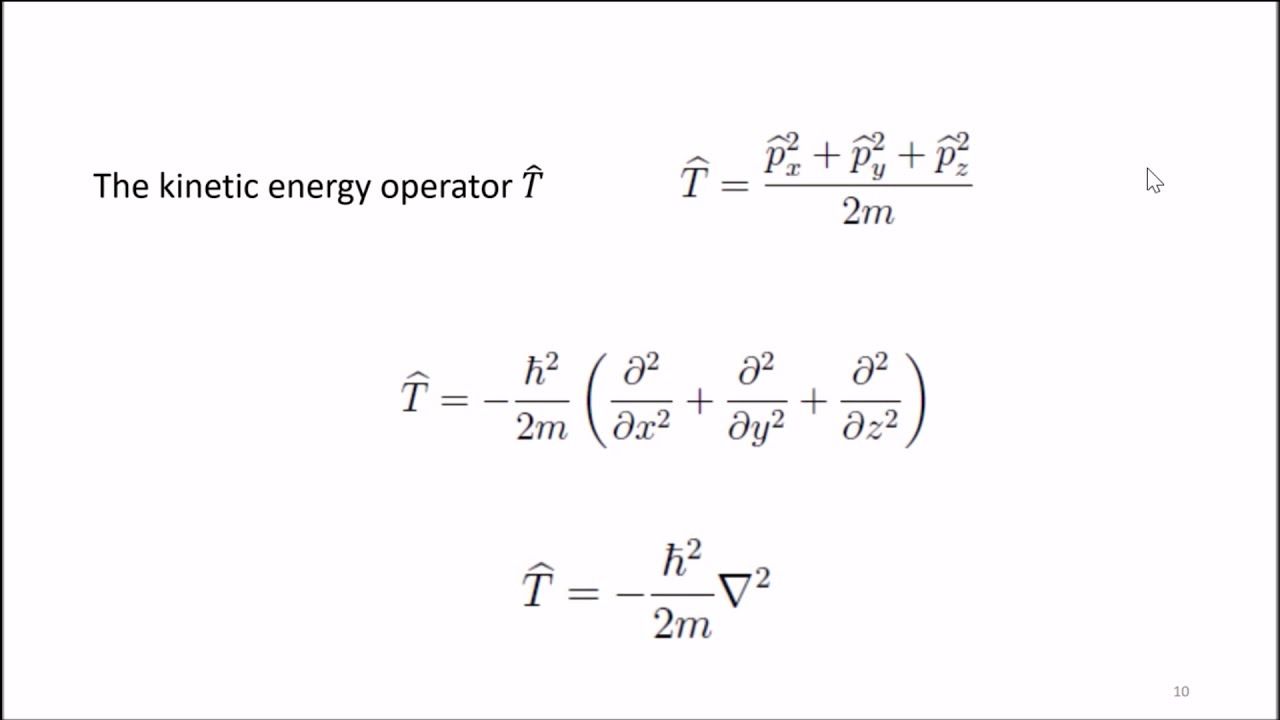
- 🌌 Eigenvalue equations also play a role in the Schrödinger equation, especially in the time-independent version, which relates to the system's energy.
- 🤔 The eigenvalue equation helps predict possible measurement outcomes in quantum systems, making it crucial for understanding real-world quantum phenomena.
Q & A
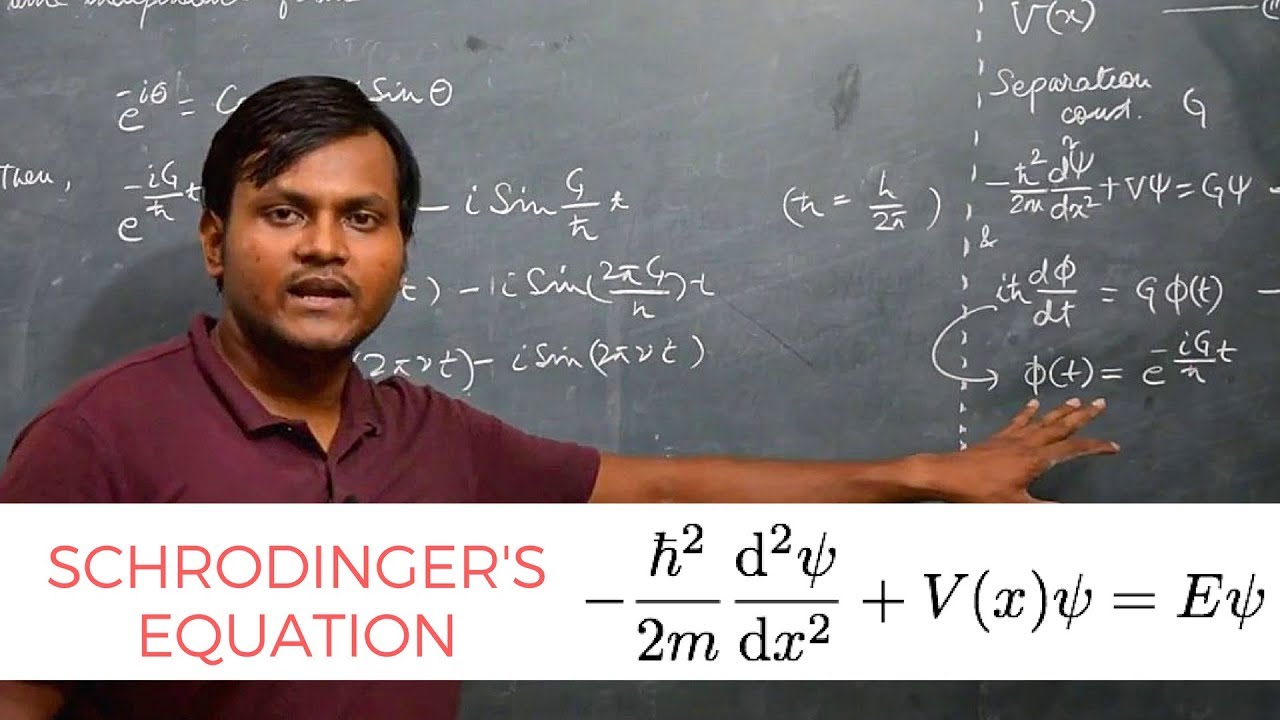
What is the Schrödinger equation, and why is it considered the most important in quantum mechanics?
-The Schrödinger equation governs how quantum states evolve over time, making it essential for understanding the dynamics of quantum systems. It is the foundation of quantum mechanics, describing the wave function and how it changes.
What is the second most important equation in quantum mechanics, according to the video?
-The second most important equation is the eigenvalue equation. This equation plays a key role in quantum mechanics by describing how certain measurements are related to specific quantum states.
How can the eigenvalue equation be represented mathematically?
-Mathematically, the eigenvalue equation can be written as a matrix applied to a vector. Some vectors, called eigenvectors, remain unchanged except for being stretched or shrunk by a scalar called the eigenvalue.
What are eigenvectors and eigenvalues in the context of quantum mechanics?
-In quantum mechanics, eigenvectors represent quantum states that do not change when a particular measurement is made. The eigenvalue is the measurement result associated with that eigenvector.
How does the concept of matrices relate to quantum mechanics?
-In quantum mechanics, matrices represent measurement operators. When applied to quantum states (which can be seen as vectors), these operators transform the states or yield eigenvalues, depending on whether the state is an eigenstate of the operator.
What is the significance of spin in quantum mechanics?
-Spin is a form of inbuilt angular momentum for particles like electrons. Even though electrons aren't physically spinning, their spin represents a fundamental property that affects measurements of their quantum state.
What happens when a measurement is applied to a quantum state that is not an eigenstate?
-If the quantum state is not an eigenstate of the measurement operator, the act of measurement causes the state to randomly collapse into one of the possible eigenstates. The probability of each outcome can be calculated using the square of certain coefficients.
What is the role of superposition in quantum measurements?
-Superposition allows a quantum system to exist in a combination of multiple states. For example, an electron can be in a blend of both clockwise and anticlockwise spin states until a measurement is made, which causes the system to collapse into one specific state.
How does the time-independent Schrödinger equation relate to the eigenvalue equation?
-The time-independent Schrödinger equation can be written as an eigenvalue equation where the operator measures the total energy of the system, and the eigenvalue is the energy level of that state.
Why is the eigenvalue equation important for quantum measurements?
-The eigenvalue equation is important because it helps determine the possible outcomes of measurements. Even if a system is not initially in an eigenstate, any quantum state can be written as a combination of eigenstates, making the equation useful in predicting results.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados
5.0 / 5 (0 votes)