What is The Schrödinger Equation, Exactly?
Summary
TLDRIn this educational video, Jade demystifies the complex Schrodinger equation, a fundamental concept in quantum mechanics. She likens it to the 'F=ma' of the quantum world, explaining how it reveals everything we can know about a quantum system. Jade breaks down the equation's components, including the wave function (denoted by the Greek letter psi), which describes the probability distribution of an electron's location, and the allowed energy levels. She also touches on the quantization of energy levels, a key feature of quantum mechanics, and the difference between potential and kinetic energy in this context. The video aims to provide viewers with a clearer understanding of the Schrodinger equation, enabling them to grasp quantum concepts better.
Takeaways
- 🧲 The Schrodinger equation is fundamental in quantum mechanics, often compared to F=ma in classical physics.
- 🌌 It encapsulates everything we can know about a quantum system, including energy levels and wave functions.
- 🎲 The wave function (represented by the Greek letter psi) provides probabilities of where a quantum object, like an electron, is likely to be found.
- 🚫 The Heisenberg Uncertainty Principle limits our ability to know both the exact position and momentum of quantum objects simultaneously.
- 🔮 The wave function describes a quantum object's probability distribution, which is spread out like a wave, unlike classical objects that occupy a single location.
- 📉 Energy in quantum systems is quantized, meaning particles can only have certain discrete energy levels, unlike the continuous range in classical physics.
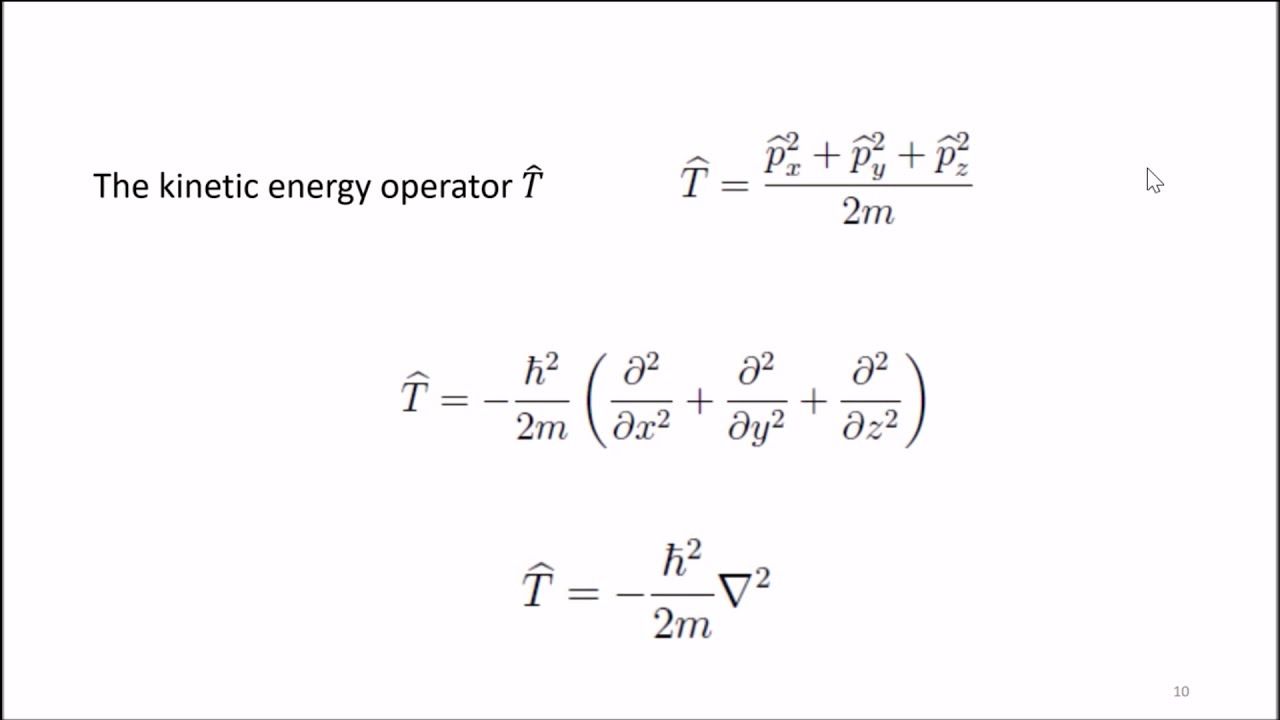
- ⚖️ The Schrodinger equation balances a quantum system's total energy as the sum of its kinetic and potential energies.
- 🔑 Solving the equation yields the energy levels and wave functions, which are key to understanding a quantum system's behavior.
- 🎓 The solutions to the Schrodinger equation involve constants and whole numbers, indicating that energy and probability distributions are quantized.
- 🔗 The script suggests using resources like Brilliant.org for a deeper understanding of quantum mechanics and the Schrodinger equation.
Q & A
What is the significance of the Schrödinger equation in quantum science?
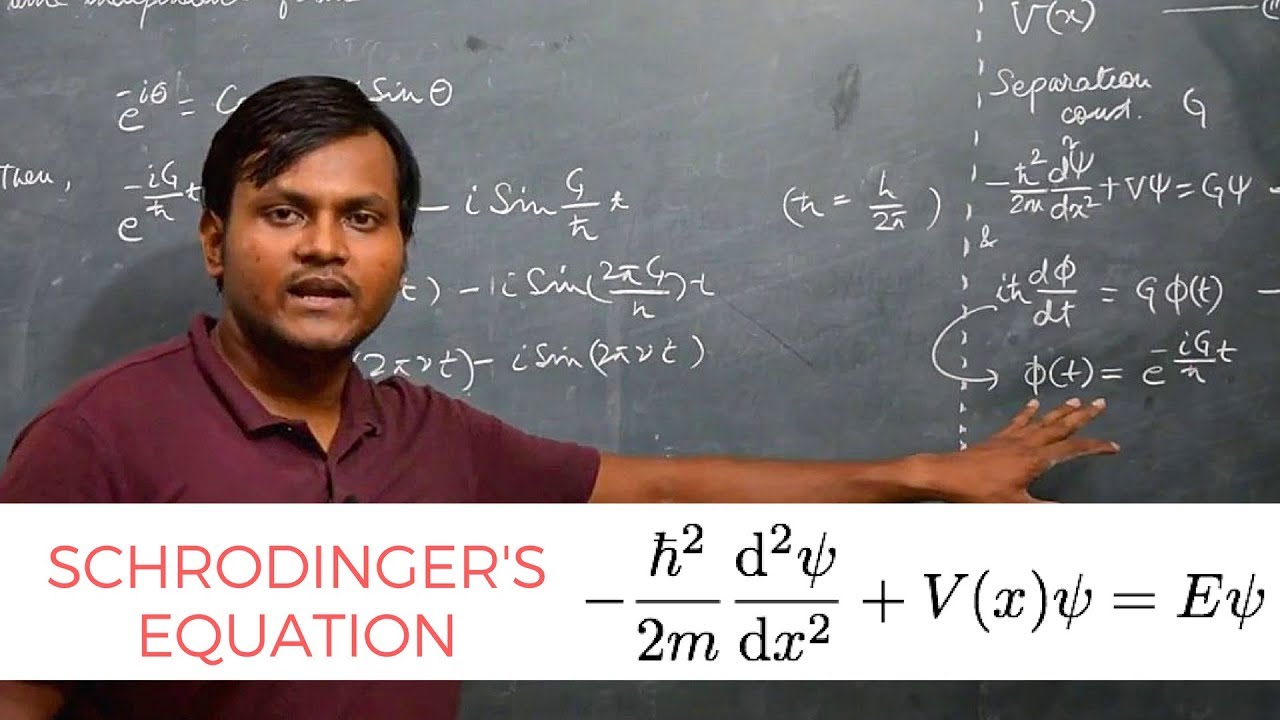
-The Schrödinger equation is fundamental in quantum science as it encapsulates everything we can know about a quantum system, much like F=ma in classical physics. It allows us to predict the energy levels and wave functions of quantum objects.
Why doesn't the classical equation F=ma apply to quantum particles?
-The classical equation F=ma does not apply to quantum particles because at the quantum level, the Heisenberg uncertainty principle prevents us from knowing both the exact position and momentum of particles simultaneously, necessitating a different approach like the Schrödinger equation.
What is the wave function (Ψ) in the context of the Schrödinger equation?
-The wave function (Ψ) represents the probability distribution of a quantum particle's location. It tells us where a particle is likely to be found but does not pinpoint an exact position until measured.
How does the Heisenberg uncertainty principle relate to the Schrödinger equation?
-The Heisenberg uncertainty principle, which states that the exact position and momentum of a quantum object cannot be simultaneously known, is inherent in the Schrödinger equation through its wave function, which only provides probabilities of location.
What does the energy term (E) in the Schrödinger equation signify?
-The energy term (E) in the Schrödinger equation signifies the allowed energy levels of a quantum system. These levels are quantized, meaning the particle can only have certain discrete energy values, reflecting the wave-like nature of quantum objects.
Why are energy levels quantized in quantum mechanics?
-Energy levels are quantized in quantum mechanics due to the wave-like nature of particles. The wave function must be zero at the boundaries (like the walls of a box), which restricts the allowable frequencies and, by Einstein's relation E=hf, the allowable energy levels.
What is the role of potential energy (V) in the Schrödinger equation?
-In the Schrödinger equation, potential energy (V) is a term that, along with kinetic energy, contributes to the total energy of the system. It represents the energy of the particle due to its position in a potential field.
How does the Schrödinger equation help in determining the behavior of an electron in a box?
-The Schrödinger equation helps determine the behavior of an electron in a box by providing solutions for the energy levels and wave functions. These solutions predict the probabilities of where the electron is likely to be and the allowed energy states it can occupy.
What is the significance of the quantized values in the solutions to the Schrödinger equation?
-The quantized values in the solutions to the Schrödinger equation are significant because they represent the discrete states that a quantum system can exist in. These values are whole numbers and constants, indicating that the system's properties are not continuous but occur in specific, quantized states.
How does the act of measurement affect the wave function of a quantum particle?
-The act of measurement causes the wave function of a quantum particle to collapse from a superposition of probabilities to a single outcome. This means the particle transitions from being in all possible states at once to being in a defined state upon observation.
What resources are available for someone looking to delve deeper into quantum mechanics and the Schrödinger equation?
-For those interested in further study, platforms like Brilliant.org offer courses on quantum mechanics, starting from foundational experiments to the derivation of the Schrödinger equation, complete with examples and problems to solve.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

What is Quantum Tunneling, Exactly?

The Quantum Barrier Potential Part 1: Quantum Tunneling
7 Postulates of Quantum Mechanics
Derive Time Independent SCHRODINGER's EQUATION from Time Dependent one
The SECOND Most Important Equation in Quantum Mechanics: Eigenvalue Equation Explained for BEGINNERS

Linearity and nonlinear theories. Schrödinger's equation
5.0 / 5 (0 votes)