Derive Time Independent SCHRODINGER's EQUATION from Time Dependent one

Summary

In this educational video, the presenter demonstrates how to transform the time-dependent Schrödinger equation into its time-independent form using the separation of variables method. This technique is crucial in quantum mechanics for analyzing the behavior of particles under potential fields that are time-independent. The video elucidates the process of reducing a complex partial differential equation to a simpler ordinary differential equation, which is essential for solving various quantum mechanical problems and understanding properties like energy levels and wavefunctions.

Takeaways

• 🔬 The video demonstrates the process of converting the time-dependent Schrödinger equation into its time-independent form using the separation of variables method.
• 🌌 In quantum mechanics, the Schrödinger equation is fundamental for studying the evolution of quantum systems, much like Newton's second law in classical mechanics.
• 📉 To use the separation of variables, the potential in the quantum system must be independent of time, allowing the wave function to be expressed as a product of spatial and temporal functions.
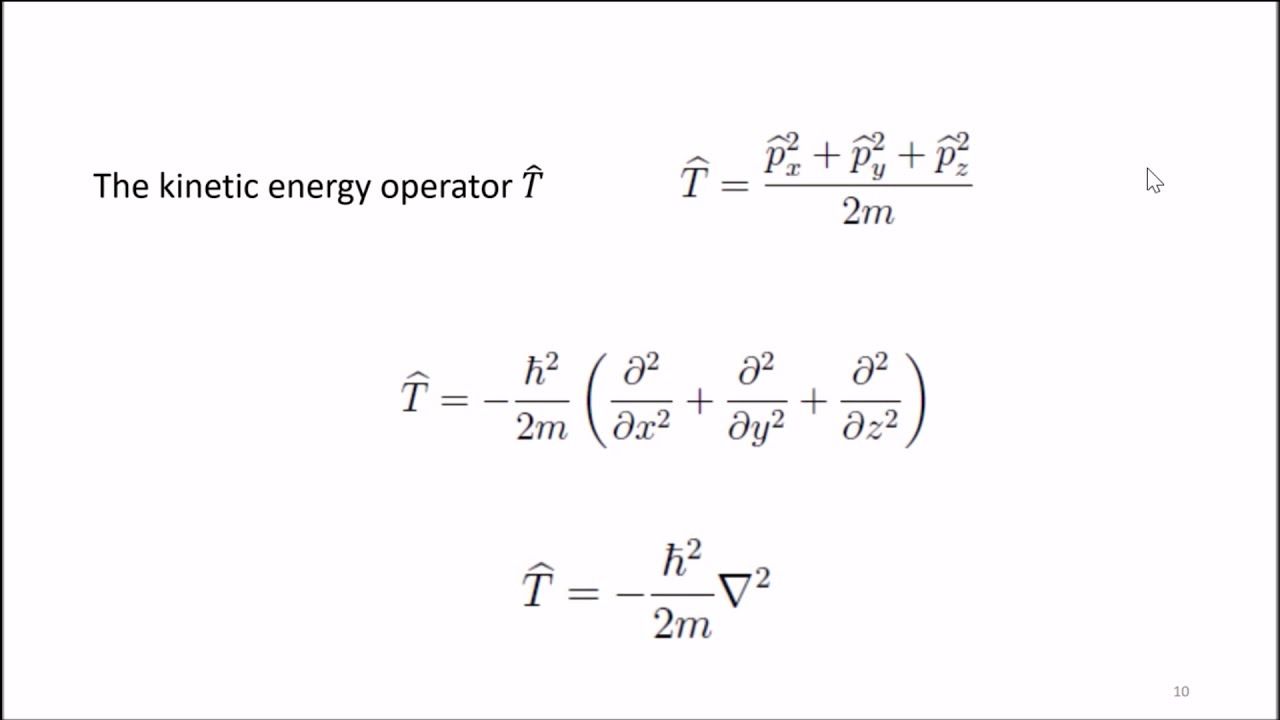
• ⏱️ The time-dependent Schrödinger equation is a partial differential equation involving both space and time derivatives, which can be simplified under the right conditions.
• 🧮 The reduction to an ordinary differential equation is achieved by assuming the wave function can be separated into functions of space and time, leading to two separate equations.
• 🔄 The separation constant, denoted as 'G', equates the spatial and temporal equations, suggesting that the energy of the system is related to the frequency of the time-dependent part of the wave function.
• 🌊 The time-independent part of the wave function is represented by a function of space only, which is crucial for solving the time-independent Schrödinger equation.
• 🌟 The solution to the time-independent equation provides eigenfunctions, which, when multiplied by the time-dependent part, give the complete wave function of the system.
• 📊 The probability density, derived from the wave function, remains constant over time for systems where the potential is time-independent, indicating stationary states.
• 🔍 The video concludes by emphasizing that the time-independent Schrödinger equation is essential for further analysis in quantum mechanical problems where the potential does not vary with time.

Q & A

What is the main topic of the video?

The main topic of the video is the reduction of the time-dependent Schrödinger equation to its time-independent form using the separation of variables method.

Why is it important to study the trajectory of a quantum mechanical particle?

Studying the trajectory of a quantum mechanical particle is important because it allows us to understand the evolution of the system, and from the solution of the Schrödinger equation, we can derive various physical quantities like position, momentum, velocity, and acceleration.

What is the fundamental equation in quantum mechanics that is discussed in the video?

The fundamental equation in quantum mechanics discussed in the video is the Schrödinger equation.

How does the separation of variables method simplify the Schrödinger equation?

The separation of variables method simplifies the Schrödinger equation by allowing the wave function to be expressed as a product of two separate functions, one dependent on space and the other on time, which reduces the partial differential equation to an ordinary differential equation.

Under what condition can the wave function be separated into space and time functions?

The wave function can be separated into space and time functions when the potential field experienced by the particle is independent of time and only depends on the spatial variable.

What is the significance of the separation constant (G) in the context of the Schrödinger equation?

The separation constant (G) in the Schrödinger equation is significant because it represents the energy of the particle in the quantum mechanical system, linking the frequency of the wave function to the energy of the particle through Planck's constant.

How is the time-independent Schrödinger equation derived from the time-dependent one?

The time-independent Schrödinger equation is derived by separating the wave function into space and time components and setting the separated spatial and temporal parts equal to a constant, which leads to two ordinary differential equations, one for the spatial part and one for the temporal part.

What is the physical interpretation of the solution to the time-independent Schrödinger equation?

The solution to the time-independent Schrödinger equation, often referred to as the eigenfunction, represents the spatial part of the wave function and is associated with the stationary states of the quantum system, where the probability density of the particle is constant over time.

Why are the solutions to the time-independent Schrödinger equation also called eigenfunctions?

The solutions to the time-independent Schrödinger equation are called eigenfunctions because they correspond to the eigenvalues of the Hamiltonian operator, which in this context represents the total energy of the system.

How does the video explain the relationship between the frequency of a particle's wave and its energy?

The video explains the relationship between the frequency of a particle's wave and its energy by referencing Planck's and Einstein's postulates, which state that the frequency (ν) is related to the energy (E) by the equation E = hν, where h is Planck's constant.

What is the final form of the wave function solution according to the video?

According to the video, the final form of the wave function solution is a product of the eigenfunction, which is a solution of the time-independent Schrödinger equation, and the time-dependent part, which is an exponential function representing the oscillatory behavior of the system.

Outlines

00:00

🔬 Introduction to Time-Dependent Schrödinger Equation

The video begins with an introduction to the process of simplifying the time-dependent Schrödinger equation into its time-independent form using the method of separation of variables. The presenter explains that in quantum mechanics, the Schrödinger equation is fundamental for studying the evolution of quantum systems. The trajectory of a quantum particle can be analyzed by solving this equation, which provides insights into various physical quantities like position, momentum, and velocity. The video aims to demonstrate how to reduce the complexity of the time-dependent equation by separating variables, a method applicable when the potential field is time-independent.

05:01

📐 Separation of Variables Method

This section delves into the mathematical process of using the separation of variables method to transform the time-dependent Schrödinger equation into an ordinary differential equation. The presenter explains that by assuming the wave function can be expressed as a product of two separate functions—one dependent on space and the other on time—the partial differential equation can be simplified. The conditions under which this method is applicable are discussed, specifically when the potential field does not vary with time. The video illustrates how to rearrange the equation and separate it into two ordinary differential equations, each dependent on a single variable.

10:01

🌌 Time-Independent Schrödinger Equation and Its Solutions

The presenter continues by solving the time-independent Schrödinger equation, which is derived from the separation of variables. The solution involves finding the eigenfunctions and eigenvalues, which are crucial for understanding the quantum states of a system. The video explains how the time-dependent part of the wave function can be represented as an exponential function of time, which includes an imaginary unit. The solutions are then decomposed into cosine and sine functions, revealing the oscillatory nature of the quantum states. The relationship between the frequency of these oscillations and the energy of the particle is discussed, linking to Planck's and Einstein's theories.

15:04

🌟 Conclusion: Stationary States and Probability Density

The final part of the video concludes with the derivation of the time-independent Schrödinger equation and its implications for quantum mechanical systems. The presenter demonstrates that the solution to the equation can be expressed as a product of spatial and temporal components, with the spatial part being the eigenfunction. It is explained that these solutions represent stationary states, where the probability density of the particle remains constant over time. The video wraps up by emphasizing the importance of solving the time-independent equation for various quantum mechanical problems and how it leads to a constant probability distribution, indicative of the system's stationary nature.

Keywords

💡Schrodinger Equation

The Schrodinger Equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes over time. In the video, it is mentioned as the starting point for studying the evolution of a quantum mechanical particle. The time-dependent form of the equation is reduced to a time-independent form using the separation of variables method, which is a key technique for solving quantum mechanical problems.

💡Separation of Variables

Separation of variables is a mathematical method used to simplify partial differential equations by breaking them down into ordinary differential equations. In the context of the video, this method is applied to the time-dependent Schrodinger Equation to reduce it to a time-independent form. This simplification allows for easier analysis and solution of quantum systems where the potential is time-independent.

💡Wave Function

The wave function, often denoted by the Greek letter psi (Ψ), is a mathematical description of the quantum state of a particle. In the video, the wave function is discussed in relation to its time-dependent and time-independent forms. The time-dependent wave function is a function of both space and time, while the time-independent wave function is represented as an eigenfunction, which is a solution to the time-independent Schrodinger Equation.

💡Eigenfunction

An eigenfunction is a function that, when used in an equation with a linear operator, returns the function itself multiplied by a scalar (the eigenvalue). In quantum mechanics, eigenfunctions represent the possible states of a quantum system. The video explains that the time-independent part of the wave function is an eigenfunction of the time-independent Schrodinger Equation.

💡Quantum Mechanical System

A quantum mechanical system refers to a physical system that is described by the principles of quantum mechanics. The video focuses on how to study such systems by solving the Schrodinger Equation to understand the behavior of particles within these systems, including their position, momentum, and energy.

💡Potential Field

A potential field is a scalar field whose negative gradient represents a force field. In the video, the potential field is mentioned as a condition for applying the separation of variables method. If the potential field is time-independent, the wave function can be expressed as a product of space and time functions, which simplifies the analysis.

💡Time-Independent Schrodinger Equation

The time-independent Schrodinger Equation is a form of the Schrodinger Equation that does not involve time derivatives, making it easier to solve for stationary states of a quantum system. The video demonstrates how to derive this equation from the time-dependent form, which is crucial for finding the eigenfunctions and eigenvalues that describe the system's energy levels.

💡Stationary States

Stationary states in quantum mechanics are states of a quantum system that do not change with time. The video explains that the solutions to the time-independent Schrodinger Equation represent these states, as their probability densities are constant over time. This is significant because it allows for the prediction of stable energy levels within a quantum system.

💡Probability Density

In quantum mechanics, probability density refers to the probability of finding a particle in a particular region of space. The video discusses how the time-independent solutions (eigenfunctions) result in a constant probability density, indicating that the likelihood of finding the particle in any given location does not change over time.

💡Planck's Constant

Planck's constant, denoted by the symbol ħ (h-bar), is a fundamental constant in quantum mechanics that relates the energy of a particle to its frequency. The video mentions Planck's constant in the context of the relationship between the energy of a particle and the frequency of its associated wave, which is crucial for understanding the time-dependent behavior of quantum systems.

Highlights

Introduction to reducing the time-dependent Schrödinger equation to its time-independent form using the separation of variables method.

Fundamental importance of Schrödinger's equation in quantum mechanics for studying the evolution of quantum mechanical particles.

The trajectory of a particle in classical mechanics is studied using Newton's second law, analogous to Schrödinger's equation in quantum mechanics.

The time-dependent Schrödinger equation is a partial differential equation involving space and time variables.

Condition for reducing the partial differential equation to an ordinary differential equation through the separation of variables.

The potential field must be independent of time for the separation of variables method to be applicable.

The wave function can be expressed as a product of space and time functions under certain conditions.

Derivation of the time-independent Schrödinger equation from the time-dependent form.

The separation constant 'G' is introduced, equating terms involving different variables.

Solving the time-independent Schrödinger equation provides the eigenfunction, a function of space only.

The time-dependent part of the wave function is solved as an exponential function of time.

The solution involves an imaginary number and Planck's constant, leading to an oscillatory function.

The frequency of the oscillatory function is related to the energy of the particle through Planck-Einstein relation.

The energy of the particle in a quantum mechanical system is represented by the separation constant 'G'.

The final wave function solution is a product of the eigenfunction and the time-dependent function.

Eigenfunctions represent stationary states where the probability density is constant with respect to time.

The probability density of the particle is independent of time, proving the stationary nature of the states.

Conclusion on how the time-dependent Schrödinger equation is reduced to a time-independent form using separation of variables.

Transcripts

so in today's video I want to show how

to reduce a time-dependent square inches

equation form into its type independent

form by using the separation of

variables method so in quantum mechanics

one of the most fundamental equations is

disk orange's equation now what happens

is that if you want to study some kind

of quantum mechanical system you want to

study the evolution of a quantum

mechanical particle you want to study

the trajectory of a particle the most

the first thing that you need to do is

solve this Cottagers equation and from

the solution you can study you can get

idea about many different physical

quantities like position momentum

velocity acceleration and all these

different things associated with that

particular system for example in

classical mechanics what you basically

do if you want to study the trajectory

of a particle is that you basically have

the Newton's second law you if you know

all the forces and you know the position

and the velocity of a particle at a

given instant in time they then you

solve the Newton's second law from that

you can find out the position with

respective time time derivative of the

position gives you an idea about the

velocity the time derivative of the

velocity gives you an idea about the

acceleration so in the same way in

quantum mechanics if you want to study a

particular system or a particle which is

bound by some kind of a force field or a

potential then you basically solve a

scourges equation and from in that

solution different kinds of information

is contained in it and how you proceed

from there on is a completely different

matter but in this video I what I want

to show is how to reduce the time

dependent Schrodinger equation to its

time independent form so the most basic

time dependence cod inches equation is

of this form

[Applause]

so as you can see in the time dependence

car in jersey collision the

wavefunctions solution sigh here is

actually a function of both space and

time looming and the potential itself is

also a function of X and T now this this

as you can see that this is a partial

differential equation which contains two

partials arrive at it so one is the

second order partial derivative of X and

the other is a first-order partial

derivative of time and I can reduce this

partial differential equation into an

ordinary differential equation if if

certain conditions are met so what

conditions on top keyboard so for

example if this particular wave function

which is both a function of X and T can

be written as product of two separate

functions one is a function of space and

the other is a function of time in that

case I can reduce this expression to an

ordinary differential equation now what

happens is that this kind of condition

is true only in those cases whenever the

particle is experiencing some kind of a

potential field where the potential

field is independent of time so

basically I have a potential field which

is independent of time and it is only

dependent on X here so for example

gravitation field electrostatic field so

a particle is experience it feels like

that which is independent of time in

those cases the wave function solution

is usually can be written as a product

of two separate functions both being

functions of independent separate

variables now if this this condition is

being met so let's say this is condition

number one and this is condition number

two so if I use so obviously these are

both related to each other so this is

known as separation of variables method

so separation of variables okay so if if

I have this particular condition I can

use this condition to reduce this

expression into a much simpler form

which can be solved for different kinds

of

quantum mechanical problems so now let's

replace equation number one in this

particular storages equation so if I do

that my equation becomes minus H cross

square by 2 m del square by Del X square

this this simple sy X plus Phi T plus V

X is equal to IH cross del Y del T off

[Applause]

now in the first term here now this is a

second order different partial

derivative of this particular product

but this product contains two separate

functions one is the function of space

there is a function of time domain since

X and T both are independent variables

so I can take this term outside the

derivative expression so this becomes

minus H cross square by 2 m Phi G del

square X by Del X square plus V X Phi X

Phi T is equal to IH cross similarly in

this term I can take the function of X

variable outside the partial derivative

of time in this case so IH cross Phi X X

my del T now one thing to notice is that

the moment I take these terms out side

the derivative expression this suddenly

becomes an ordinary derivative right

this becomes an ordinary gravity does

not remain a partial derivative anymore

because the function here is a function

of only one variable so it becomes the

ordinary derivative now to simplify it I

what I do is so this is Phi T I take

this out minus H cross square by 2 m

okay now taking these two expressions on

the other side interchanging them I get

so or so I get 1 by Phi X minus H cross

square by 2 m now as you can see this

differential equation has been reduced

to an ordinary differential equation in

which on the left hand side all these

terms all both of these two terms are

basically functions of X there is no

dependence on the variable of time T and

on the right hand side this term here is

as dependent is a function of time there

is no dependence of X now X M T these

are both independent variables and on

the left hand side if you have some kind

of a function of X and on the right hand

side if you have some kind of a function

of T and both these two expressions are

equal to each other since there is no

dependence of since both X and T are

independent of each other then the only

conclusion that you can derive from this

kind of an equation is that they are

both equal to some kind of a constant

and let's denote this kind of a constant

value to be let's say something like G

okay G is a separation constant so we

call G as the separation constant G okay

now if I if I if I do this then I can I

can separate both these two equations in

separate this entire equation do two

different equations so I can write that

minus H cross square by 2 m d square

side by DX square plus v sy is equal to

G I let's say this is point number three

and we also have IH cross D Phi by DT is

equal to G Phi T yes so now let's look

at the fourth equation let's look at the

fourth equation

in the fourth equation you have AI H

cross the Phi by DT is equal to G Phi or

D Phi by DT is equal to 1 by h plus G

Phi which can be written as if you take

the energy number I upwards this is

going to become minus I by H cross G Phi

or this is written as D Phi by DT is

equal to I by H cross hi gh plus fight

right so I is the imaginary number H

cross is Appliance constant divided by 2

pi G is the separation constant which is

equal to both these two different

expressions okay

now you solve this equation is very

simple so if you if you if you have some

equation of the form let's say dy by DT

is equal to let's say some kind of a

constant alpha multiplied by Y okay

because this entire equation is a this

form then the solution of this kind of

an equation is written as y is equal to

Y is equal to e to the power alpha T

this is a solution of an equation like

that y you can check that if I find the

first order derivative of D Y with

respect to time then dy by DT is equal

to so D by DT of e to the power alpha

which is basically the alpha is a

constant comes outside alpha e to the

power alpha T so either alpha is equal

to Y so this can be written as L so Y

alpha Y so dy by DT is equal to alpha y

is the different first order

differential equation whose solution is

y is equal to EI fod okay

so now I will use this conclusion here

that if we have this constant I G by H

cross is equal to some kind of a

constant alpha

IgH crosses some prolific constant he'll

find that case the solution Phi T I'm

sorry the - is here there's a minus sign

so - this thing okay so Phi T is equal

to Phi T is equal to e to the power

alpha T which is equal to e to the power

minus i g by h cross so this is the

solution of equation number four okay

this is the solution of equation number

four so equation number four has a

solution of Phi T is equal to e to the

power minus i g pi h cross t okay this

is a solution now let's look at this

particular solution the solution is

basically exponential to the power

something multiplied by time

now what and this sum this constant also

includes an Imagineer number now if you

have you have expressions of the form e

to the power i Tita then they are

basically written as cos theta plus I

sine theta right and if you have

expressions of re to power minus I theta

this can be written as cos theta minus I

theta so if I if I if I if I also

decomposed this particular expression in

the terms of cosines and sines then

basically I can write as e to the power

minus i g by H cross T is equal to

course cost

G by H cross t minus I sine G to the

power H cross okay where H cross is

nothing but H by 2 pi so this is

basically cause G by 2 pi G by h t minus

I sine 2 pi G bar H okay now as you can

see here

this is some kind of an oscillatory

function this is some kind of an

oscillator and the oscillator dependence

has a frequency of is equal to cos 2 pi

mu t minus I sine 2 pi right where the

frequency nu is is equal to G by H okay

because these are not just mathematical

functions they are supposed to represent

a some kind of particle which is

experience experiencing some kind of a

potential field now if if we have a time

dependence which is oscillatory in

nature then that oscillation of the that

time dependent function has an

expression which is equal to G by H but

we already know as given by as given by

Einstein and Planck's equation is that

the frequency of any kind of a wave

associated with some kind of a particle

has a relationship with Planck's

constant and energy which is expression

is given by e is equal to H nu so this

comes from Planck Einsteins postulate so

this expression was most initially given

by Max Planck when he was trying to

explain the phenomena of blackbody

radiation and later on it was also

followed by I'm trying to explain the

photoelectric effect so we already know

that whenever we have some kind of a

particle which has a wave associated

with it and the frequency of the wave is

related to its energy by this kind of an

expression so so by looking at this

similarity of this expression we can

conclude that this or G is equal to H u

in this case G is nothing but the energy

of that particle

stuck in that kanafeh given quantum

mechanical system so G is actually equal

to the energy by comparing the Max

Planck I'm Stein wash rate of relating

frequency with that of the energy and

the conclusion that we came up both from

here so G is nothing but the energy of

the particle stuck in that kind of a

quantum mechanical system so if G is

equal to e in that case so J is equal to

EE then equation number four equation

number four gives us the solution Phi T

is equal to e to the power minus i ii by

H cross P so this is the function which

shows you the dependence on time and

equation number three takes the form of

minus H cross square by 2m d square side

by DX square yes v sy is equal to e sy

so this here this equation here is the

time independent Schrodinger equation

this is the time independent scrod

inches equation which can be further

used in whatever problem we are studying

to to to know about the solution so

these the sy here is known as the

eigenfunction and sy is nothing but a

function of X now for all kinds of cases

of quantum mechanical systems where we

can use separation of variable methods

if the potential field is independent of

time in all of those cases the time

dependence can be written in this form

and then we have the space dependence

given by the time independent

Schrodinger equation so the next step is

to obviously solve this equation

depending upon what kind of problem we

have at hand so the final solution so

the final wave function solution

therefore can be represented as wave

function solution can be represented as

a product of this eigenfunction side and

T here so this can be written as

[Applause]

so the solution of the score dangers

equation has a wave function which can

be written in this form and this is the

eigen function which is a solution of

the time-independent orangist equation

now this is also known as eigen function

or sometimes also known as stationary

States because this is independent of

the time domain and if you solve this

time independent religious equation for

some particular quantum mechanical

problems then you will find that the

probability density of the particle for

those kind of cases are always constant

with respect to time so the there is the

the so if this is the case then the

probability density of the particle

which is a wave function solution that

looks like this is independent of

independent of time how how can you

prove that so for example if you have if

you want to find the probability

probability probability distribution of

a particle you basically do si star sy

DX right so if you do this so this this

exponential terms gets cancelled out and

you're left to it this particular term

only which is independent of x the

probability distribution is independent

of time and therefore this is also you

end up getting stationary States so this

is how you can reduce the time

dependents what is equation to a time

independent form by using the separation

of variables method thank you