Geometry 8-3: The Law of Sines
Summary
TLDRIn this lesson on the law of sines, we explore how it can be applied to solve problems involving triangles, both acute and obtuse. The video covers key concepts like using sine ratios to find side lengths and angles, particularly in 30-60-90 triangles. Step-by-step examples demonstrate the use of the law of sines to calculate missing side lengths and angles, showcasing real-world applications like navigation. The video also introduces the law of sines in non-right triangles, emphasizing its broad applicability beyond right triangles. Throughout, viewers are encouraged to use calculators for precise results and are guided through examples to solidify their understanding.
Takeaways
- 😀 The Law of Sines is used to solve problems in non-right triangles, such as acute and obtuse triangles.
- 😀 For a 30-60-90 triangle, the sine ratios of opposite sides over the hypotenuse are always consistent and equal.
- 😀 To calculate sine ratios correctly, always ensure parentheses are used properly in your calculator to avoid errors.
- 😀 The Law of Sines allows for the determination of side lengths and angle measures in triangles, even if they are not right triangles.
- 😀 The sine of an angle is equal to the opposite side divided by the hypotenuse in right triangles, which extends to all triangles through the Law of Sines.
- 😀 The Law of Sines applies universally to any triangle, not just right triangles. This property makes it powerful for solving triangles of any type.
- 😀 The Law of Sines formula states that sine of angle A over side a equals sine of angle B over side b, which equals sine of angle C over side c.
- 😀 By using the Law of Sines, we can find missing side lengths or angles, such as calculating the length of side yz by setting up proportions.
- 😀 In practice, solving for an unknown angle involves using the inverse sine function on a calculator to determine the angle measure.
- 😀 The Law of Sines is also useful in real-world applications, such as flight navigation, where distances are calculated around obstacles like thunderstorms.
Q & A
What is the Law of Sines, and how is it applied in solving triangles?
-The Law of Sines is a mathematical formula that relates the angles of a triangle to the lengths of its sides. It states that for any triangle ABC, the ratio of the sine of an angle to the length of the opposite side is constant, i.e., sine(A)/a = sine(B)/b = sine(C)/c. It is used to find unknown side lengths or angles in non-right triangles.
How can you determine if two ratios of sine and sides are equal using the Law of Sines?
-To determine if two ratios are equal, you can use the Law of Sines by setting up proportions based on the sine of angles and the lengths of the opposite sides. For example, if sine(60°)/5√3 = sine(30°)/5, then both ratios are equal, as shown in the video. This can be confirmed using a calculator.
Why do the sine ratios in a 30-60-90 triangle always have the same value?
-In a 30-60-90 triangle, the ratio of the sine of each angle to its opposite side remains constant because the side lengths follow a fixed ratio. Specifically, in such a triangle, if the hypotenuse is twice the length of the shorter leg, the ratios for sine remain consistent for these specific angles.
How does the Law of Sines apply to acute and obtuse triangles?
-The Law of Sines applies to both acute and obtuse triangles, as it is not limited to right triangles. It works for any triangle as long as the angles and opposite sides are known, enabling the determination of unknown angles or side lengths.
What is the importance of constructing altitudes when applying the Law of Sines?
-Constructing altitudes is important because it helps form right triangles within the original triangle. These right triangles allow for the use of sine ratios (opposite/hypotenuse) to express relationships between sides and angles, which is fundamental to deriving the Law of Sines.
How do you use the Law of Sines to find an unknown side length?
-To find an unknown side length using the Law of Sines, set up a proportion involving the sine of an angle and the lengths of the opposite sides. For example, if you know sine(51°)/x = sine(77°)/7, you can solve for x by rearranging and calculating the result using a calculator.
How do you calculate the angle of a triangle using the Law of Sines?
-To calculate an angle using the Law of Sines, set up a proportion involving the sine of the known angle and its opposite side, and then use the inverse sine function (shift or second sine) on your calculator to solve for the angle.
What does the Law of Sines tell us about the relationship between angles and sides in a triangle?
-The Law of Sines establishes that for any triangle, the sine of each angle is proportional to the length of its opposite side. This means that the angles and side lengths are related through their sine values, providing a method to find unknown values in a triangle.
Why do you need to use the inverse sine function when solving for an angle in a triangle?
-The inverse sine function is used to solve for an angle when the ratio of the opposite side to the hypotenuse (sine value) is known. It allows you to find the angle whose sine is equal to the given ratio.
What is the process to find the distance traveled in a real-life scenario, such as the flight route example in the video?
-To find the distance traveled in a real-life scenario like the flight route, apply the Law of Sines to the triangle formed by the flight path. By setting up proportions involving known side lengths and angles, you can determine the unknown distances. For example, using sine(113°)/471 = sine(22°)/x, you can find the distance from the turning point to Omaha, and then apply similar steps for the remaining distance.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

LEI DOS SENOS | TEOREMA DOS SENOS

KELILING DAN LUAS SEGITIGA #MENEMUKAN RUMUS LUAS SEGITIGA#CONTOH SOAL#MATEMATIKA KELAS7
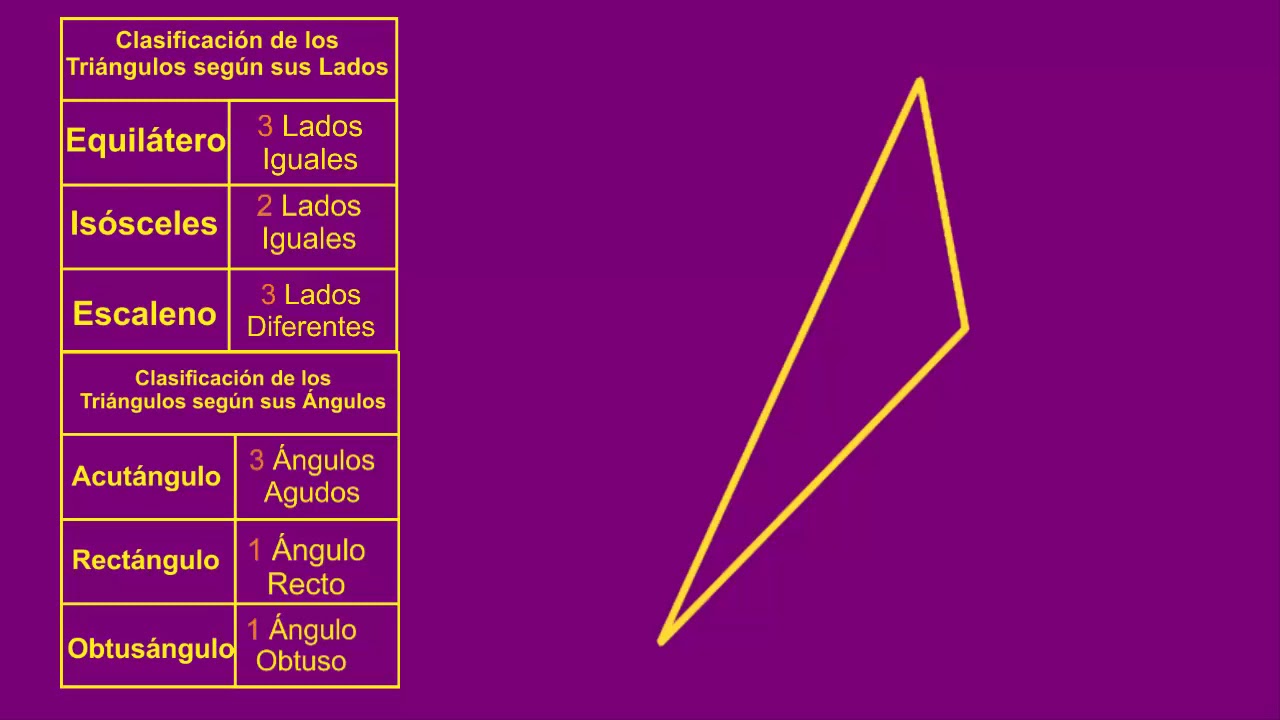
CLASSIFICATION OF TRIANGLES, according to their sides and according to their angles
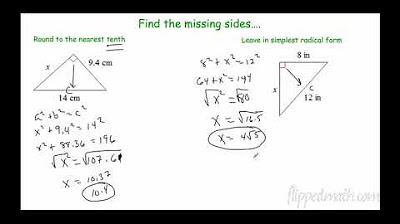
Geometry – 7.1 Pythagorean Theorem and Its Converse

tripel pythagoras kelas 8 part 2 (mudah) dan menentukan jenis segitiga - Abi Muis Math
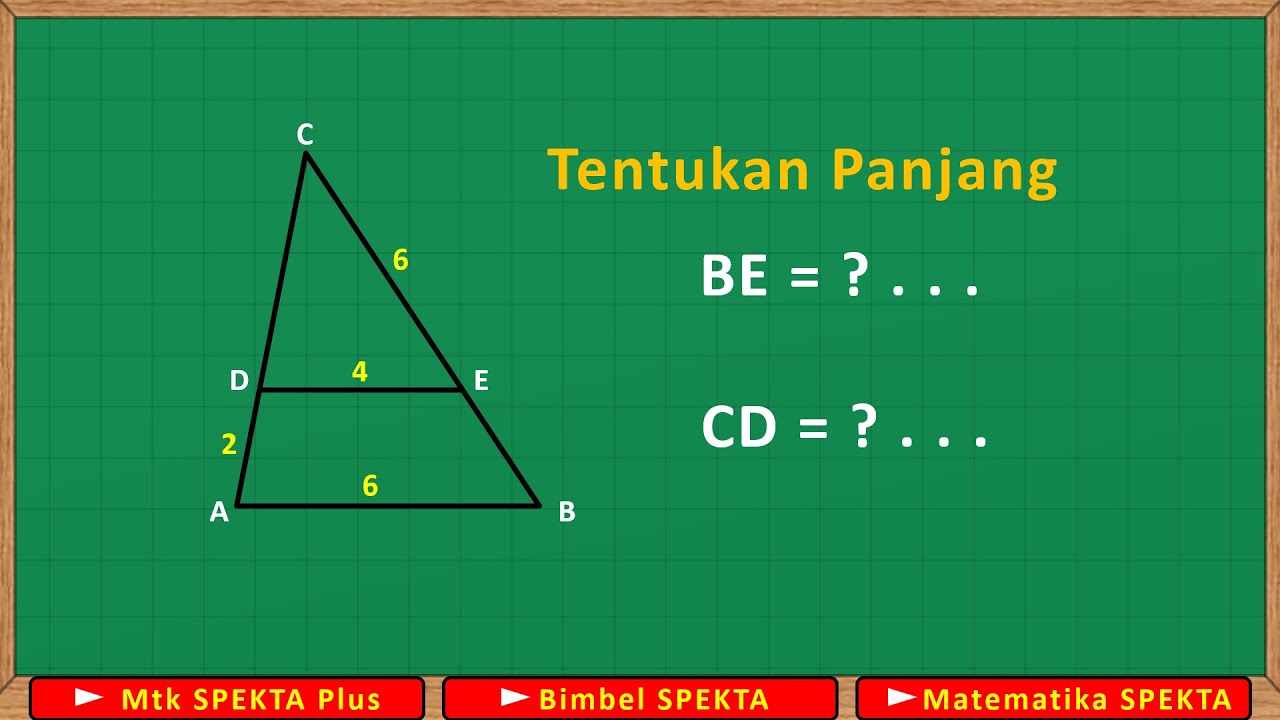
Kesebangunan Pada Segitiga. Part 3, Contoh Perhitungan
5.0 / 5 (0 votes)
