💥Razón ARITMÉTICA y razón GEOMÉTRICA (incluye ejemplos)
Summary
TLDRIn this educational video, viewers learn about arithmetic and geometric ratios. The presenter explains arithmetic ratios as the comparison of two quantities through subtraction, and geometric ratios through division. Examples are provided to illustrate how to solve problems involving these ratios, including finding the greater and lesser numbers based on given conditions. The video also demonstrates how to work with equations derived from the ratios, guiding viewers through step-by-step solutions to find the required values. The content is aimed at helping students understand and apply these concepts in practical problems.
Takeaways
- 😀 Arithmetic ratio is the comparison of two quantities through subtraction, where the first is the antecedent and the second is the consequent.
- 😀 The result of the arithmetic ratio is denoted by 'r', which is the result of the subtraction of the two quantities.
- 😀 Geometric ratio involves comparing two quantities through division, with the first being the antecedent, the second the consequent, and the result denoted by 'k'.
- 😀 An example problem is given where the arithmetic ratio between two numbers is 20, and the geometric ratio is 2, leading to solving for the larger number.
- 😀 In solving the example, it is crucial to express the geometric ratio as a fraction, where the geometric ratio of 2 can be written as 2/1.
- 😀 The next step is to replace constants in the geometric ratio and solve the resulting equations to find the value of the numbers.
- 😀 The arithmetic equation is manipulated by substituting the values for 'a' and 'b', leading to a solution where the larger number is identified as 40.
- 😀 Another example problem is given where the geometric ratio is 4/7 and the arithmetic ratio is 45, asking to find the smaller number.
- 😀 In this second example, the geometric ratio is expressed as a fraction, and the arithmetic ratio is solved through subtraction.
- 😀 The final answer to the second problem shows that the smaller number is 60, following the calculation and comparison of the results.
- 😀 Throughout the explanation, the importance of consistently applying the rules for both arithmetic and geometric ratios to solve problems effectively is emphasized.
Q & A
What is the arithmetic ratio?
-The arithmetic ratio is the comparison of two quantities through subtraction, where the first quantity is called the antecedent and the second is called the consequent.
What is the geometric ratio?
-The geometric ratio is the comparison of two quantities through division, where the first quantity is called the antecedent and the second is called the consequent.
In the example where the arithmetic ratio is 20 and the geometric ratio is 2, what is the greater number?
-The greater number is 40. This is determined by solving the equations derived from the arithmetic and geometric ratios.
How do we handle the geometric ratio when there is no fraction present?
-We assume that the denominator is 1 if no fraction is given, as in the example where the geometric ratio is written as 2/1, which simplifies to 2.
What is the role of the constant added to the fractions in these problems?
-The constant is added to both the numerator and denominator to help solve the equations, where the variables are represented by 'a' and 'b'.
How do we determine the greater or lesser number in a problem involving the arithmetic ratio?
-We determine the greater number by comparing the two numbers in the equation derived from the arithmetic ratio. The larger number is placed first when doing the subtraction.
In the example where the geometric ratio is 4/7 and the arithmetic ratio is 45, how do we solve for the smaller number?
-We solve for the smaller number by setting up an equation using the arithmetic ratio and then solving for 'a' and 'b', where the lesser number turns out to be 60.
What does the 'k' represent in the context of the geometric ratio?
-'k' represents the result of comparing the two quantities through division in the geometric ratio.
How is the arithmetic ratio equation formulated in the examples?
-The arithmetic ratio equation is formed by subtracting the variable 'b' (the consequent) from the variable 'a' (the antecedent), and setting the result equal to the given arithmetic ratio.
What is the method used to find the final values for 'a' and 'b' in the examples?
-The method involves setting up equations based on the given ratios, solving them algebraically, and then substituting the values back to find the final values of 'a' and 'b'.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
MATH 10 : DIFFERENTIATING GEOMETRIC SEQUENCE FROM AN ARITHMETIC SEQUENCE (Taglish)
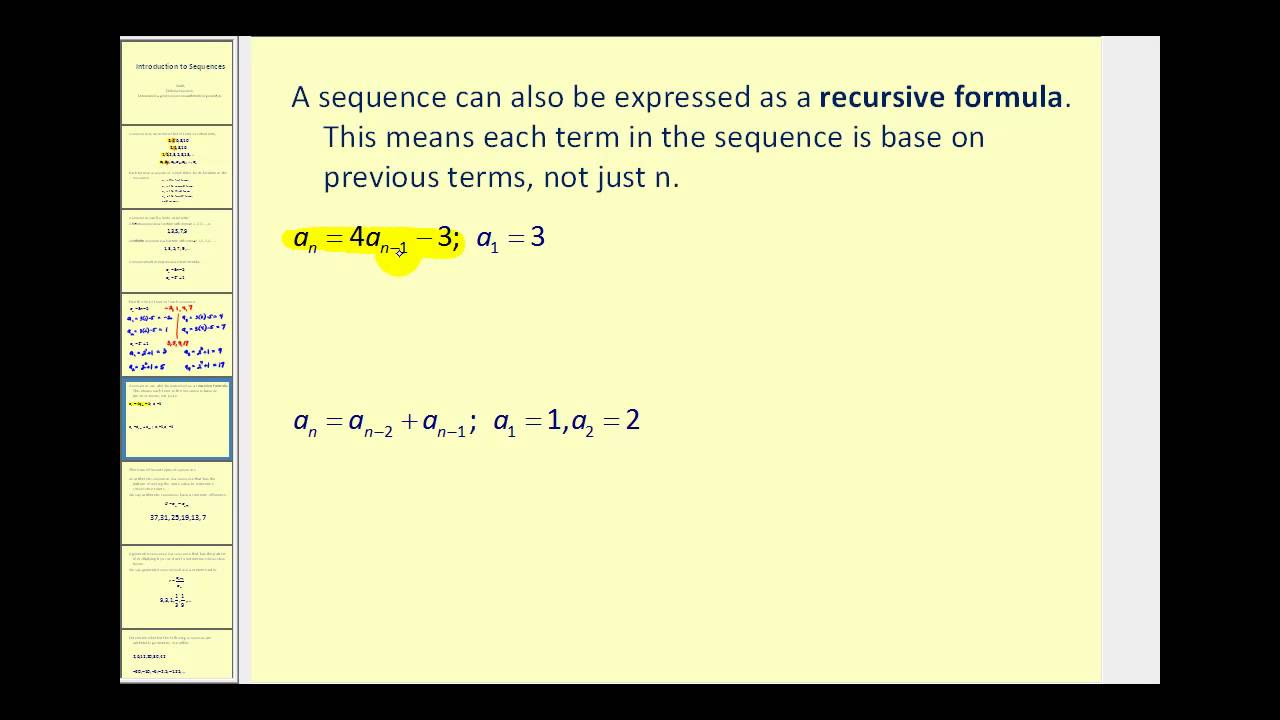
Introduction to Sequences

BARISAN ARITMATIKA DAN GEOMETRI (APERSEPSI)
Math Antics - Number Patterns

Grade 10 Math Q1 Ep6: Geometric Sequence VS Arithmetic Sequence

Pembahasan BARISAN DAN DERET (Aritmetika & Geometri) KELAS 11 | #MatematikAsik
5.0 / 5 (0 votes)
