Introduction to Sequences
Summary
TLDRThis video introduces sequences, explaining their types (finite and infinite) and how each term is identified by its position. It covers how sequences can be arithmetic (adding a common difference) or geometric (multiplying by a common ratio). The video explains sequences as functions, discusses recursive formulas where each term depends on the previous ones, and demonstrates how to calculate terms in both arithmetic and geometric sequences. It also shows how to identify patterns, determine common differences, and calculate ratios, preparing viewers for further exploration in upcoming videos.
Takeaways
- 📚 A sequence is an ordered list of elements where each term is identified by its position in the sequence.
- 🔢 Sequences can be finite, with a specific number of terms, or infinite, continuing indefinitely.
- 📊 Sequences can be considered functions, where the domain is the position of terms and the range is the terms themselves.
- 🧮 To find specific terms in a sequence, we use a formula where n represents the term's position.
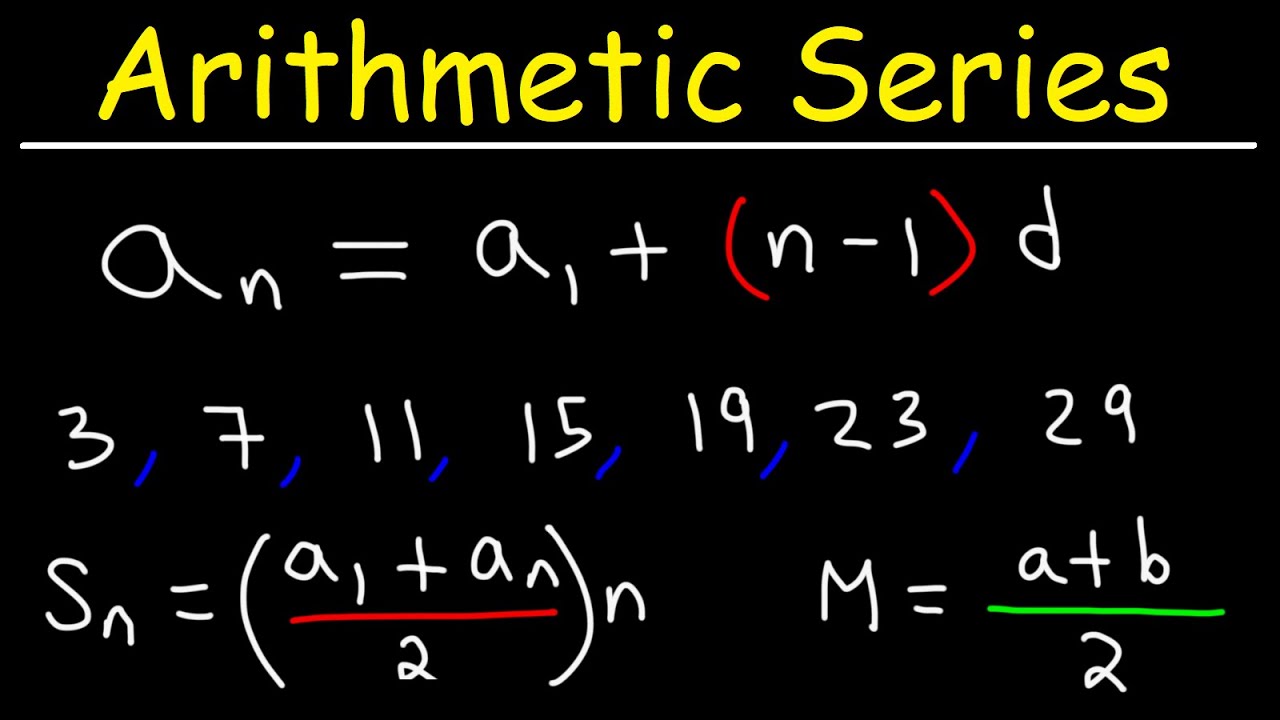
- ➗ Arithmetic sequences involve adding the same value (common difference) to each term.
- ✖️ Geometric sequences involve multiplying by the same value (common ratio) between terms.
- 🔄 Recursive formulas calculate each term based on previous terms, rather than directly using n.
- 🧑🏫 Arithmetic sequences have a common difference, which can be found by subtracting any term from the previous one.
- 📐 Geometric sequences have a common ratio, which can be determined by dividing any term by the previous one.
- 📖 Recursive sequences can also require two previous terms to generate the next term.
Q & A
What is a sequence?
-A sequence is an ordered list of terms or elements, where each term is identified by its position in the sequence. Terms are often referred to as a sub 1, a sub 2, a sub 3, etc., based on their location in the sequence.
What are the two types of sequences?
-There are two types of sequences: finite and infinite sequences. A finite sequence has a specific number of terms and then stops, while an infinite sequence continues indefinitely, often indicated by '...' at the end.
How can a sequence be considered a function?
-A sequence can be considered a function where the domain is 1, 2, 3, and so on (representing the term's position), and the range is the set of values that make up the sequence.
How can you find specific terms in a sequence using a formula?
-To find a term in a sequence, you can use the formula provided for the sequence. For example, to find the first term (a sub 1), substitute n = 1 into the formula. This process can be repeated for subsequent terms.
What is a recursive formula in a sequence?
-A recursive formula defines each term in a sequence based on previous terms, not just its position (n). For example, to find the third term (a sub 3), you would need the second term (a sub 2).
How does an arithmetic sequence differ from a geometric sequence?
-In an arithmetic sequence, a constant value (the common difference) is added to generate each successive term. In contrast, a geometric sequence involves multiplying by a constant (the common ratio) to generate successive terms.
What is the common difference in an arithmetic sequence?
-The common difference in an arithmetic sequence is the fixed value added to each term to generate the next one. It can be found by subtracting any term from the previous one.
How do you find the common ratio in a geometric sequence?
-The common ratio in a geometric sequence can be found by dividing any term by the previous term. This ratio remains constant throughout the sequence.
Can arithmetic sequences have negative common differences?
-Yes, an arithmetic sequence can have a negative common difference. In this case, you subtract a fixed value from each term to get the next one, as seen when the common difference is negative.
How can you identify a geometric sequence when examining terms?
-A geometric sequence is identified by a consistent multiplication pattern between terms. For instance, if the ratio between consecutive terms is constant (such as dividing by 3 or multiplying by 1/3), the sequence is geometric.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
5.0 / 5 (0 votes)