Arithmetic Sequences | HSF.LE.A.2 🖤
Summary
TLDRThis video lesson on arithmetic sequences guides viewers through identifying terms, finding common differences, and writing arithmetic sequences as functions. The instructor explains how sequences are ordered lists of numbers that follow a rule, with a focus on understanding consecutive terms and the common difference between them. Examples include adding both positive and negative differences. The lesson concludes with writing equations for arithmetic sequences and finding specific terms, such as the 35th or 45th, using these formulas. The session emphasizes how arithmetic sequences form discrete linear functions.
Takeaways
- 😀 Arithmetic sequences involve an ordered list of numbers where the difference between consecutive terms is constant.
- 📈 Each term in an arithmetic sequence has a position (e.g., the first term is a₁, the second term is a₂, etc.).
- ➕ The difference between consecutive terms in an arithmetic sequence is called the common difference, denoted as d.
- 🔢 To find the next terms in a sequence, add the common difference to the previous term.
- 🧮 An arithmetic sequence can be expressed as a function, where aₙ = a₁ + (n - 1) * d.
- ✏️ You can graph an arithmetic sequence, which forms a discrete linear function with a constant slope equal to the common difference.
- 📊 The slope of the graph of an arithmetic sequence represents the common difference, and the function is linear.
- 🔗 The equation for an arithmetic sequence helps calculate specific terms, including large ones like the 35th or 45th terms, by using the formula.
- 📉 The process for deriving the equation involves distributing and simplifying terms based on the first term and the common difference.
- 📐 Arithmetic sequences always have whole number inputs, making them discrete, not continuous functions.
Q & A
What is an arithmetic sequence?
-An arithmetic sequence is a sequence where the difference between each pair of consecutive terms is the same, known as the common difference.
How do you identify if a sequence is arithmetic?
-To identify if a sequence is arithmetic, check if the difference between consecutive terms is consistent. This difference must remain the same throughout the sequence.
What is the common difference, and how is it represented?
-The common difference is the consistent value that is added to each term in an arithmetic sequence to obtain the next term. It is represented by the variable 'd'.
How do you find the nth term of an arithmetic sequence?
-The nth term of an arithmetic sequence can be found using the formula: aₙ = a₁ + (n-1)d, where a₁ is the first term, n is the position of the term, and d is the common difference.
Why do we subtract 1 in the formula for finding the nth term?
-We subtract 1 in the formula (n-1) because the common difference is added to the first term after the first step. So, to find the nth term, we calculate the number of steps (n-1) between the first term and the nth term.
How would you extend the sequence: 3, 6, 9, 12?
-To extend the sequence, you would keep adding the common difference of 3 to each term. So the next three terms would be: 15, 18, and 21.
What is a discrete function in relation to an arithmetic sequence?
-A discrete function is a function where the input values are specific integers, such as term numbers in an arithmetic sequence. The values do not include fractions or decimals, so the sequence is made of distinct, separate points.
How do you graph an arithmetic sequence?
-To graph an arithmetic sequence, plot the term number (n) on the x-axis and the value of the term (aₙ) on the y-axis. The graph will form a series of points that align in a straight line if the sequence is linear.
What is the significance of the slope in an arithmetic sequence?
-In an arithmetic sequence, the slope of the graph represents the common difference. It shows how much the value of the terms increases or decreases for each step in the sequence.
How would you find the 35th term in an arithmetic sequence?
-To find the 35th term, use the formula: aₙ = a₁ + (n-1)d. Substitute a₁ (the first term), n=35, and the common difference d into the formula to calculate the value of the 35th term.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

Grade 10 Math Q1 Ep6: Geometric Sequence VS Arithmetic Sequence

Grade 10 Math Q1 Ep2: Generate Patterns From a Given Succession of Objects
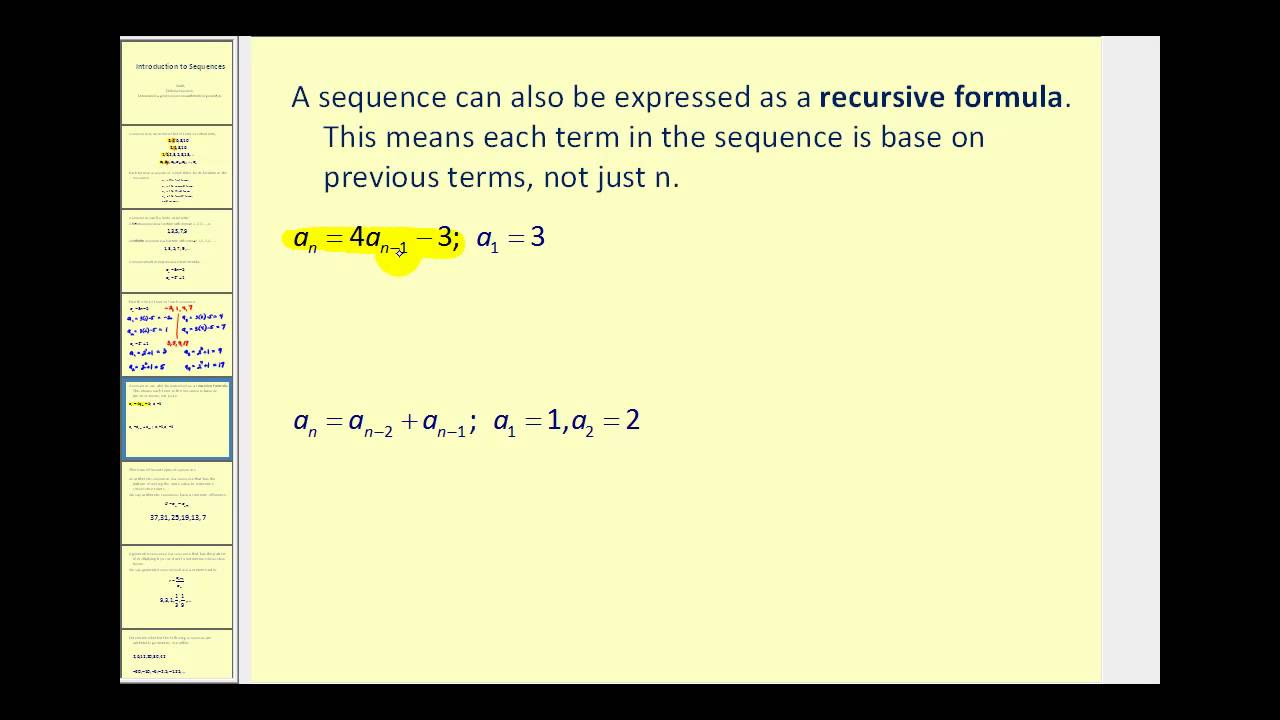
Introduction to Sequences

Arithmetic Progression Class 10

Grade 10 Math Q1 Ep4: Computing Arithmetic Means

Grade 10 MATH Q1 Ep3: Write and Use the Formula of the nth Term of an Arithmetic Sequences
5.0 / 5 (0 votes)
