UNDERSTAND, DESCRIBE, AND INTERPRET GRAPHS OF POLYNOMIAL FUNCTIONS | GRADE 10 MATH | TAGLISH
Summary
TLDRThe video lesson focuses on understanding, describing, and interpreting graphs of polynomial functions, beginning with a review of the Remainder and Factor Theorems. It uses examples to demonstrate synthetic substitution for evaluating polynomials and synthetic division for finding factors. The instructor explains how to determine x-intercepts and y-intercepts, emphasizing the importance of plotting points on a Cartesian plane. Additionally, the lesson covers leading coefficient tests to predict graph behaviors and the calculation of turning points. The engaging presentation aims to enhance students' comprehension of polynomial graphing techniques.
Takeaways
- 😀 Understanding graphs of polynomial functions involves interpreting their characteristics such as intercepts and end behavior.
- 📊 The remainder theorem helps evaluate polynomial functions using synthetic substitution.
- 🔍 The factor theorem identifies factors of polynomials by confirming if the remainder is zero.
- ✏️ To find x-intercepts, set y equal to zero and solve for x using the factor form of the polynomial.
- 📉 The y-intercept can be found by substituting x = 0 into the polynomial.
- 📈 Creating a table of values helps in plotting additional points on the graph of a polynomial.
- 🔑 The leading coefficient test determines the end behavior of polynomial graphs based on degree and coefficient sign.
- 💡 Polynomial functions can have multiple turning points, calculated using the formula n - 1, where n is the degree.
- 🔄 Recognizing the importance of synthetic division in polynomial factorization is crucial for simplifying complex expressions.
- 🎥 The video emphasizes the significance of practicing these concepts through example problems and visual aids.
Q & A
What is the main topic of the lesson discussed in the video?
-The main topic is understanding, describing, and interpreting graphs of polynomial functions.
What are the remainder and factor theorems?
-The remainder theorem states that the remainder of a polynomial division can be found by evaluating the polynomial at a specific value, while the factor theorem states that a polynomial has a factor (x - k) if the polynomial evaluated at k is zero.
How do you use synthetic substitution to evaluate a polynomial?
-Synthetic substitution involves writing the coefficients of the polynomial, using the value for x to perform calculations that yield the polynomial's value at that point.
What is the significance of x-intercepts and y-intercepts in polynomial graphs?
-X-intercepts are the points where the graph intersects the x-axis (where y = 0), while y-intercepts are where the graph intersects the y-axis (where x = 0). Both are crucial for sketching the graph.
How can you find the x-intercepts of the polynomial y = x^3 + 6x^2 + 3x - 10?
-To find the x-intercepts, set y to 0 and factor the polynomial, which yields the factors (x + 5)(x + 2)(x - 1). The x-intercepts are -5, -2, and 1.
What is the process for determining the y-intercept of a polynomial function?
-To find the y-intercept, set x to 0 and evaluate the polynomial. For the given polynomial, this results in a y-intercept of -10.
What is the leading coefficient test, and how does it help in graphing polynomials?
-The leading coefficient test helps determine the end behavior of a polynomial graph based on the sign and degree of the leading term. It indicates whether the graph rises or falls as x approaches positive or negative infinity.
How do you determine the number of turning points in a polynomial graph?
-The number of turning points can be determined using the formula n - 1, where n is the degree of the polynomial. For a cubic polynomial, the maximum number of turning points is 2.
What is the relationship between the degree of a polynomial and its end behavior?
-The degree and leading coefficient determine the end behavior: an odd degree with a positive leading coefficient will fall to the left and rise to the right, while an even degree with a negative leading coefficient will fall in both directions.
Why is it important to plot additional points when graphing a polynomial function?
-Plotting additional points helps provide a more accurate representation of the polynomial's behavior between the intercepts, ensuring a more precise sketch of the graph.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة

Polinomial (Bagian 4) - Teorema Sisa dan Teorema Faktor
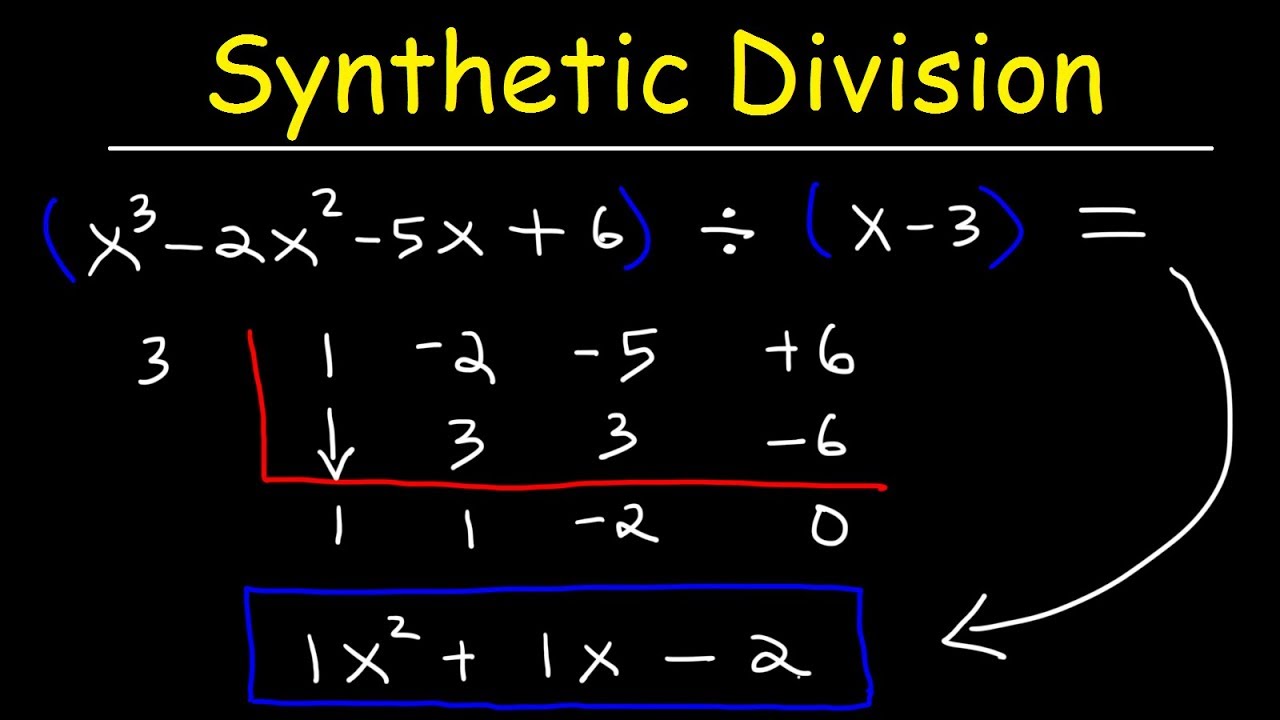
Synthetic Division of Polynomials

Suku Banyak (teorema sisa dan teorema faktor) #matematika #sma #polinom #sukubanyak #teoremasisa

Graphs of Polynomials: Identification and Characterization
Representations of Rational Function
FUNCTIONS | SHS GRADE 11 GENERAL MATHEMATICS QUARTER 1 MODULE 1 LESSON 1
5.0 / 5 (0 votes)
