Polinomial (Bagian 4) - Teorema Sisa dan Teorema Faktor
Summary
TLDRThe video script is a comprehensive tutorial on polynomial division and factor theorems. It introduces the Remainder Theorem and Factor Theorem, explaining how to find the remainder of a polynomial division without performing the actual division process. The script provides step-by-step examples, including substituting values to simplify calculations. It also covers how to determine factors of a polynomial when the remainder is zero upon division. The tutorial aims to make polynomial division more efficient and understandable for viewers.
Takeaways
- 📚 The video is part of a series discussing polynomials, specifically focusing on the fourth part which covers the Remainder Theorem and Factor Theorem.
- 🔍 The Remainder Theorem states that the remainder of the division of a polynomial f(x) by x - k is f(k), and the video explains how to find this remainder without full division.
- 📝 An example is provided to illustrate the Remainder Theorem, showing the process of substituting the value that makes the divisor zero into the polynomial to find the remainder.
- 📉 The video also explains the second form of the Remainder Theorem, which involves dividing by x + b and finding the remainder by substituting -b into the polynomial.
- 🔢 A detailed example is given for dividing a cubic polynomial by a linear binomial, demonstrating the step-by-step process of finding the remainder.
- 📈 The third form of the Remainder Theorem is introduced for dividing by a quadratic polynomial, resulting in a remainder that is a first-degree polynomial.
- 🔑 The Factor Theorem is discussed, which states that a polynomial f(x) is a factor of another polynomial if the remainder is zero when f(x) is divided by it.
- 🌰 An example polynomial is factored using the Factor Theorem, showing that x - 1 is a factor and then finding the other factor by dividing the polynomial by x - 1.
- 📚 The video concludes with a brief mention of the next topic, which will be polynomial equations, indicating a continuation of the series.
- 👋 The video ends with a sign-off greeting, wishing the viewers well in Arabic, which is a common practice in educational content to maintain cultural relevance.
Q & A
What is the main topic discussed in the fourth part of the polynomial series video?
-The main topic discussed in the fourth part of the polynomial series video is the Remainder Theorem and Factor Theorem.
What is the Remainder Theorem in the context of the video?
-The Remainder Theorem, as discussed in the video, is a method to find the remainder of a polynomial division without performing the actual division process.
How can one find the remainder of a polynomial division using the Remainder Theorem?
-To find the remainder of a polynomial division using the Remainder Theorem, one can substitute the value that makes the divisor zero into the polynomial and evaluate it.
What is an example of using the Remainder Theorem as shown in the video?
-An example given in the video is to find the remainder of the polynomial \( x^4 - 2x^3 + 4x^2 - 5 \) divided by \( x - 1 \). By substituting \( x = 1 \) into the polynomial, the remainder is found to be -2.
What is the second Remainder Theorem mentioned in the video?
-The second Remainder Theorem mentioned in the video states that the remainder of a polynomial \( f(x) \) divided by \( x + k \) is \( f(-k) \).
Can you provide an example of the second Remainder Theorem from the video?
-An example from the video is to find the remainder of the polynomial \( x^3 - 2x^2 + 3 \) divided by \( 2x + 3 \). By setting \( 2x + 3 = 0 \) and solving for \( x \), we get \( x = -\frac{3}{2} \). Substituting this value into the polynomial gives the remainder.
What is the third Remainder Theorem discussed in the video?
-The third Remainder Theorem discussed in the video is for dividing a polynomial by a quadratic polynomial, where the remainder is a linear polynomial \( S = P(x) + Q \) with \( Fa = p + q \) and \( Fb = pb \) plus a constant \( K \).
How does the Factor Theorem relate to the Remainder Theorem?
-The Factor Theorem is closely related to the Remainder Theorem. A polynomial \( f(x) \) is a factor of another polynomial if the remainder is zero when the latter is divided by the former.
What is an example of using the Factor Theorem from the video?
-An example from the video is the polynomial \( x^3 + x^2 + x - 3 \). By testing \( x = 1 \) and finding that the remainder is zero, it is concluded that \( x - 1 \) is a factor of the polynomial.
What is the significance of the Remainder Theorem and Factor Theorem in polynomial division?
-The Remainder Theorem and Factor Theorem are significant as they provide a quick way to determine the remainder of a polynomial division and to identify factors of a polynomial without performing long division.
What is the next topic to be covered after the Remainder and Factor Theorems in the polynomial series video?
-The next topic to be covered after the Remainder and Factor Theorems in the polynomial series video is polynomial equations.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
UNDERSTAND, DESCRIBE, AND INTERPRET GRAPHS OF POLYNOMIAL FUNCTIONS | GRADE 10 MATH | TAGLISH

Lesson 33 Long Division to Find Roots
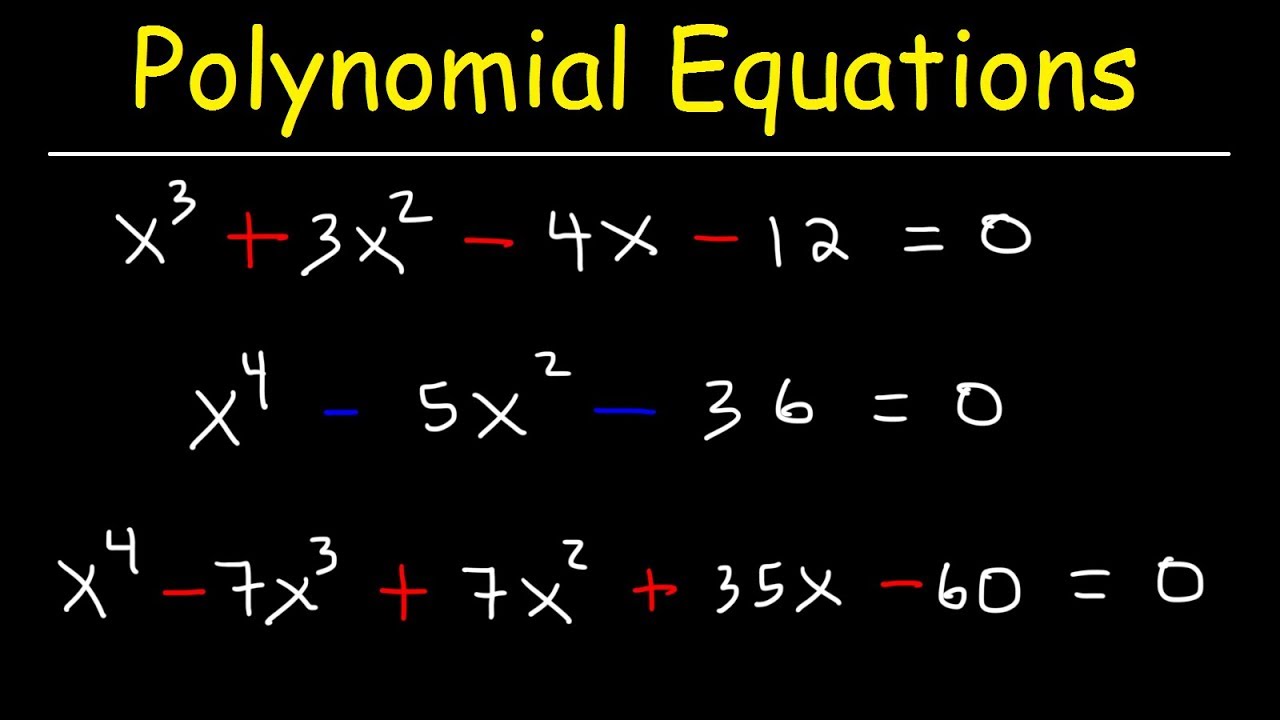
Solving Polynomial Equations By Factoring and Using Synthetic Division
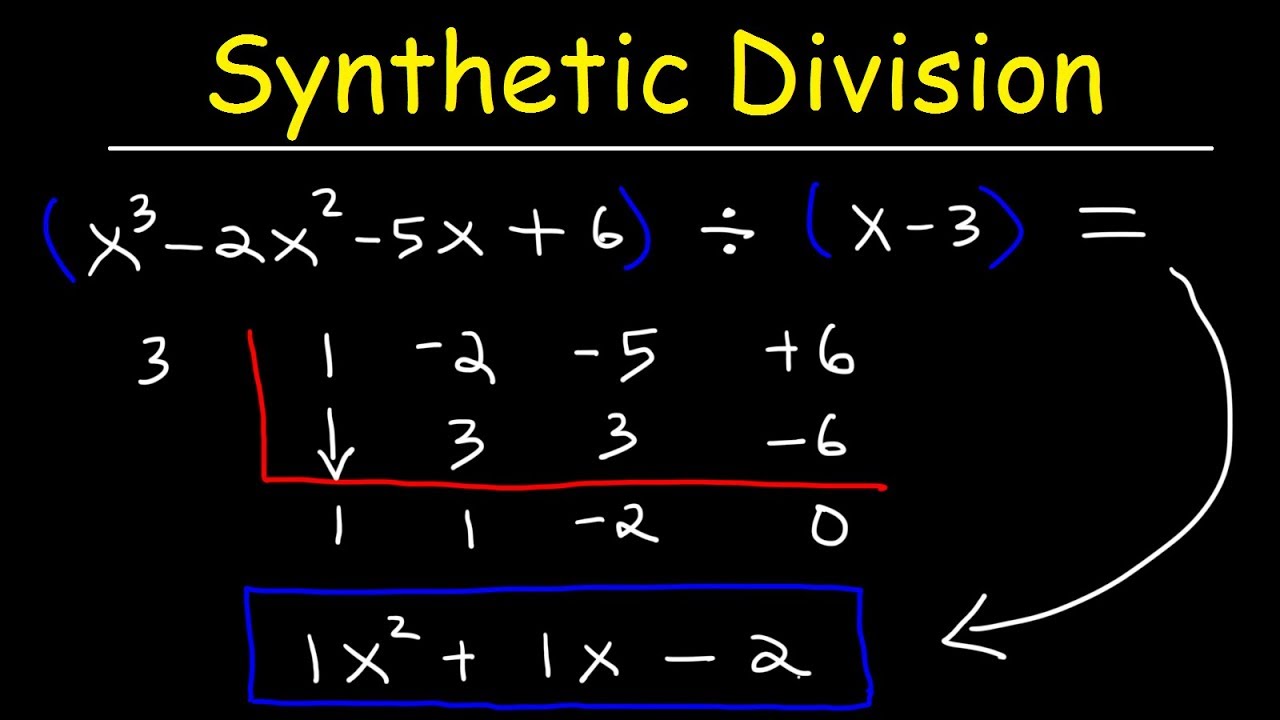
Synthetic Division of Polynomials

Latihan PAT Polinom/Suku Banyak ke (2) Matematika Peminatan XI MIPA Rosidawati Sinurat M.Pd

Polinomial (Bagian 3) - Pembagian Polinomial Cara Bersusun, Horner dan Horner - Kino
5.0 / 5 (0 votes)