BARISAN ARITMATIKA DAN GEOMETRI (APERSEPSI)
Summary
TLDRIn this educational video, Ibu Astrit explains the concept of arithmetic and geometric sequences through two examples. The first example discusses Tina's increasing savings each month, where the amount saved rises by Rp500 each month. The second example covers the growth in hotel visitors over the years, with the number of guests doubling each year. The video aims to help viewers understand the differences between arithmetic and geometric sequences, as well as how to calculate changes in each sequence.
Takeaways
- 😀 The video introduces the concept of arithmetic and geometric sequences.
- 😀 It differentiates between arithmetic and geometric sequences through examples.
- 😀 The first example involves Tina, who saves a specific amount of money each month, showing an arithmetic progression in her savings.
- 😀 Tina starts by saving Rp 2,000 in January and increases her savings by Rp 500 each month.
- 😀 The second example discusses the increasing number of hotel visitors each year, which follows a geometric progression.
- 😀 The hotel example highlights how the number of visitors increases by a consistent factor each year, starting with 42 visitors in 2015.
- 😀 The difference between arithmetic and geometric sequences is emphasized through these two examples.
- 😀 Arithmetic sequences involve a constant addition or subtraction of terms, while geometric sequences involve multiplication or division by a constant factor.
- 😀 The video encourages viewers to identify patterns in real-life situations to better understand these mathematical concepts.
- 😀 Viewers are challenged to predict future values in both Tina’s savings and the hotel visitor numbers based on the patterns provided.
- 😀 The video concludes with a reminder for viewers to analyze the patterns to understand whether changes are due to addition/subtraction (arithmetic) or multiplication/division (geometric).
Q & A
What is an arithmetic sequence?
-An arithmetic sequence is a sequence of numbers in which the difference between consecutive terms is constant.
What is a geometric sequence?
-A geometric sequence is a sequence where each term after the first is found by multiplying the previous term by a constant, known as the common ratio.
How does Tina’s savings illustrate an arithmetic sequence?
-Tina's savings demonstrate an arithmetic sequence because the amount she saves each month increases by a constant amount. For example, she saves Rp2,000 in January, Rp2,500 in February, and Rp3,000 in March, showing a consistent increase of Rp500 each month.
How does the increase in hotel guests demonstrate a geometric sequence?
-The increase in hotel guests demonstrates a geometric sequence because the number of guests added each year increases by a constant multiple. For instance, the increase from 2015 (42 guests) to 2016 (84 guests) is doubled, and from 2016 to 2017 (168 guests), it also doubles.
What is the common difference in Tina's savings pattern?
-The common difference in Tina's savings pattern is Rp Rp500, as she saves an additional Rp500 every month.
What is the common ratio in the hotel guests increase?
-The common ratio in the increase of hotel guests is 2, as the number of guests added each year doubles (42 guests, 84 guests, 168 guests).
How would you calculate Tina's total savings at the end of the year (December)?
-To calculate Tina's total savings at the end of the year, you would sum up the savings each month. Since this is an arithmetic sequence, the total can be calculated using the formula: Total = n n/2 * (first term + last term), where n is the number of terms.
If the hotel’s guest increase pattern continues, how many new guests would arrive in 2018?
-If the pattern continues, the increase in hotel guests in 2018 would be double the number from 2017, which is 168 guests. So, the increase would be 336 guests in 2018.
What is the main difference between an arithmetic sequence and a geometric sequence?
-The main difference between an arithmetic and geometric sequence is that in an arithmetic sequence, the terms increase or decrease by a constant difference, while in a geometric sequence, each term is multiplied by a constant ratio to get the next term.
How would you describe the progression of Tina's savings in terms of sequence?
-Tina's savings follow an arithmetic progression, where each month's deposit increases by the same amount (Rp500). This shows a linear progression where the difference between consecutive terms remains constant.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
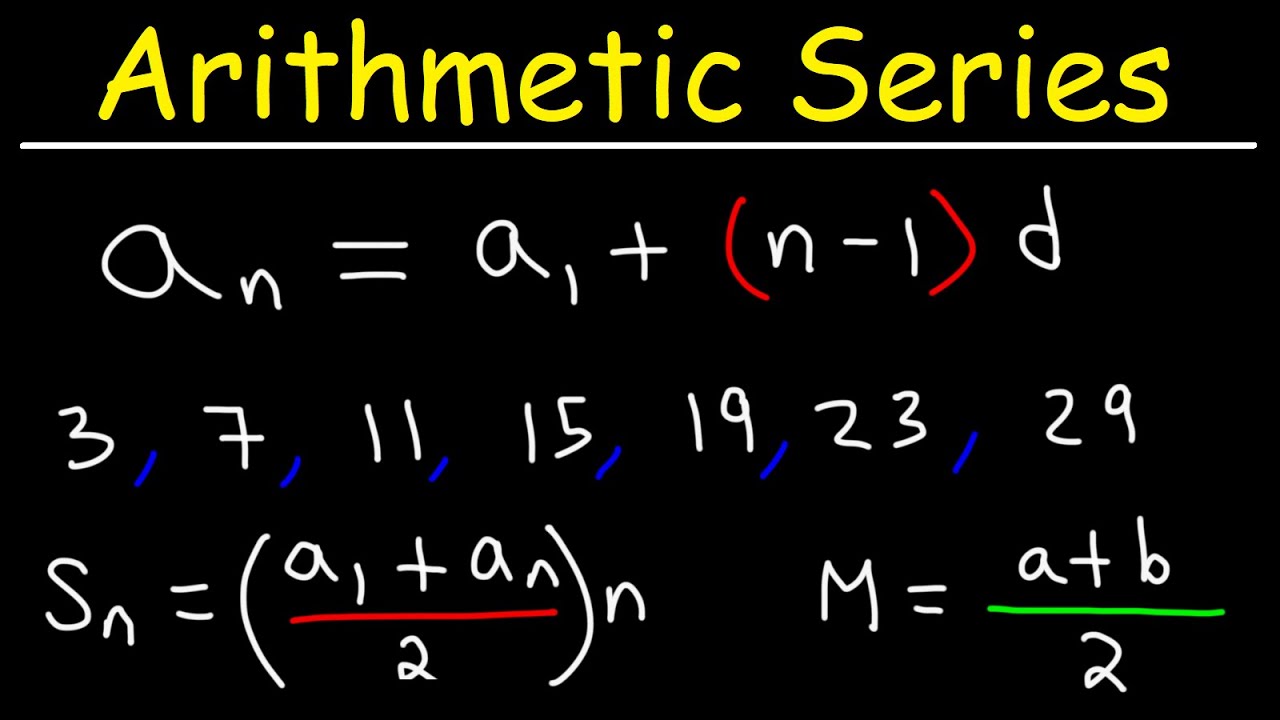
Arithmetic Sequences and Arithmetic Series - Basic Introduction

GCSE Maths - Types of Number Sequences - Arithmetic vs Geometric

Sequences and Series (Arithmetic & Geometric) Quick Review

Barisan dan deret Geometri kelas 10
MATH 10 : DIFFERENTIATING GEOMETRIC SEQUENCE FROM AN ARITHMETIC SEQUENCE (Taglish)

Pembahasan BARISAN DAN DERET (Aritmetika & Geometri) KELAS 11 | #MatematikAsik
5.0 / 5 (0 votes)
