Trigonometri : Ukuran Sudut (Derajat dan Radian) #fazanugas

Summary

In this educational video, Alaika Faza explains the concept of angle measurements, specifically focusing on degrees and radians. She covers the definitions and differences between the two units, highlighting that a full circle is 360° or 2π radians. The video also teaches how to convert between degrees and radians using simple formulas. With practical examples, Alaika demonstrates how to convert angle values from radians to degrees and vice versa, providing a clear understanding of angular measurements for viewers. The lesson is designed to help learners grasp these important concepts effectively.

Takeaways

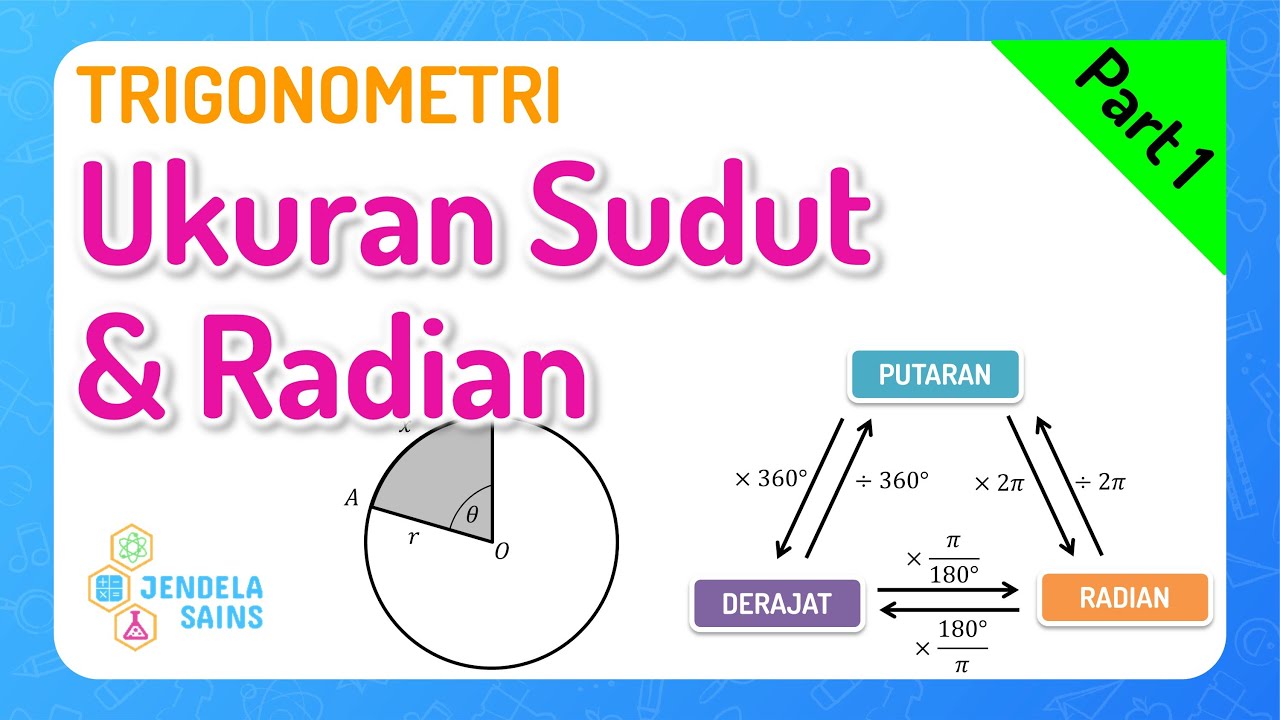
• 😀 One full rotation of a circle is 360°.
• 😀 1° is equivalent to 1/360 of a full circle rotation.
• 😀 Each hour on a clock forms an angle of 30° (360° ÷ 12).
• 😀 A radian is different from a degree; 180° equals π radians.
• 😀 A radian is the angle formed when the length of the arc of a circle is equal to the radius of the circle.
• 😀 The relationship between degrees and radians: 360° = 2π radians.
• 😀 One full rotation of a circle is 2π radians, which is equivalent to 360°.
• 😀 To convert radians to degrees, multiply by 180°/π.
• 😀 Example conversion: 2/3π radians equals 120°.
• 😀 To convert degrees to radians, divide by 180° and multiply by π.

Q & A

What is the definition of a full rotation in degrees and radians?

A full rotation is 360° or 2π radians. This means that a complete revolution around a circle corresponds to both 360 degrees and 2π radians.

How do you convert from radians to degrees?

To convert from radians to degrees, multiply the radian value by 180/π. For example, 2/3 π radians is equivalent to 120°.

What is the relationship between 1 degree and a full circle?

1 degree is equal to 1/360th of a full circle. Since a full circle is 360°, each degree represents a small fraction of that total.

What is the meaning of 1 radian in terms of arc length and radius?

1 radian is the angle formed when the length of the arc of a circle is equal to the radius of that circle. In other words, the angle is formed when the arc's length is the same as the radius.

Why is 1 radian approximately equal to 57.3°?

1 radian is roughly equal to 57.3° because it is the angle corresponding to an arc length that equals the radius of the circle. This can be derived from the formula for converting between radians and degrees.

How can you visualize angles using a clock as an example?

On a clock, the full circle is 360°. The 12-hour divisions on the clock represent 30° each. Therefore, each hour on the clock corresponds to a 30° angle.

How do you convert from degrees to radians?

To convert from degrees to radians, multiply the degree value by π/180. For example, 45° is equivalent to π/4 radians.

What is the formula for converting from 2/3 π radians to degrees?

To convert 2/3 π radians to degrees, multiply by 180/π. This results in 2/3 * 180 = 120°, as shown in the example in the video.

What does it mean when an angle is described as 'π radians'?

π radians represents half of a full rotation or 180°. In other words, an angle of π radians corresponds to a straight line or half of the full circle.

What is the advantage of using radians over degrees in certain calculations?

Radians are often more convenient in mathematical formulas, especially in calculus and trigonometry, because they are directly related to the arc length and radius of a circle. This avoids the need for conversion factors like those required in degrees.