Inverse trig functions: arctan | Trigonometry | Khan Academy
Summary
TLDRThis educational video script explains the concept of the inverse tangent function, also known as arctangent, in a clear and accessible manner. The presenter uses the analogy of a street conversation to introduce the topic and then proceeds to demonstrate how to find the angle whose tangent is a given number, specifically focusing on the example of arctangent of -1. The explanation includes a visual representation of the unit circle and the properties of a 45-45-90 triangle to derive the angle in both degrees and radians. The script also discusses the importance of restricting the range of the inverse tangent function to avoid multiple solutions and ensure a one-to-one mapping, concluding with a verification using a calculator.
Takeaways
- 😀 The script explains the concept of the arcsine and arctangent functions, emphasizing their equivalence to finding an angle where the sine or tangent of that angle equals a given value.
- 📚 The inverse sine function is introduced as a way to express the arcsine, highlighting that it's not a negative power but rather a way to denote the angle for which the sine equals a certain value.
- 🔍 The script uses the example of finding the arctangent of -1 to illustrate the process of determining the angle that corresponds to a given tangent value.
- 📈 The concept of the unit circle is reintroduced to help visualize the relationship between sine, cosine, and tangent in terms of the coordinates on the circle.
- 📐 The tangent function is defined as the ratio of the sine to the cosine, or the slope of a line drawn from the origin to a point on the unit circle.
- 📉 The script demonstrates how to find the angle with a tangent of -1 by drawing a 45-45-90 triangle on the unit circle and identifying the corresponding angle as -45 degrees.
- 🔢 The conversion from degrees to radians is explained, showing how -45 degrees is equivalent to -π/4 radians for the arctangent of -1.
- 🔁 The script discusses the periodic nature of the tangent function and the importance of restricting the range of the arctangent function to avoid multiple solutions.
- 🚫 It is emphasized that the range of the inverse tangent function is typically limited to the interval (-π/2, π/2) to ensure a one-to-one mapping and to exclude points where the tangent is undefined.
- 📊 The script concludes with a verification using a calculator to confirm that the arctangent of -1 is indeed -π/4, reinforcing the accuracy of the manual calculation.
Q & A
What is the arcsine of x?
-The arcsine of x, also known as the inverse sine function, represents the angle whose sine is x. It is denoted as sin^(-1)(x) or arcsin(x).
How is the inverse sine function written?
-The inverse sine function can be written in two ways: as sin^(-1)(x) or as arcsin(x), both meaning the angle whose sine is equal to x.
What is the relationship between the tangent function and the slope of a line?
-The tangent of an angle theta (tan(theta)) is equivalent to the slope of a line that passes through the origin and makes an angle theta with the positive x-axis.
What is the definition of the arctangent of x?
-The arctangent of x, or inverse tangent, is the angle whose tangent is equal to x. It is denoted as tan^(-1)(x) or arctan(x).
Why is it necessary to restrict the range of the inverse tangent function?
-The range of the inverse tangent function is restricted to avoid a one-to-many mapping, ensuring that each input x corresponds to a unique angle theta.
What is the relationship between the tangent function and the coordinates on the unit circle?
-The tangent function is related to the coordinates on the unit circle by the ratio of the y-coordinate (sine of theta) to the x-coordinate (cosine of theta).
What is the arctangent of -1?
-The arctangent of -1 is -45 degrees or -π/4 radians, which corresponds to an angle in the unit circle where the slope of the line from the origin to the point on the circle is -1.
How do you determine the angle for a given tangent value using the unit circle?
-To determine the angle for a given tangent value, draw the unit circle and consider the slope of the line from the origin to the point on the circle. The angle that gives the desired slope is the arctangent of the tangent value.
Why does the tangent function become undefined at certain angles?
-The tangent function becomes undefined at angles where the cosine of the angle is zero, such as π/2 and -π/2 radians, because division by zero is undefined.
What is the domain and range of the tangent function?
-The domain of the tangent function is all real numbers except for odd multiples of π/2 radians (or 90 degrees), while the range is all real numbers (minus infinity to positive infinity).
How can you verify the result of the arctangent function using a calculator?
-You can verify the result of the arctangent function using a calculator by inputting the value of x and checking if the calculator's output matches the calculated angle in radians or degrees.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
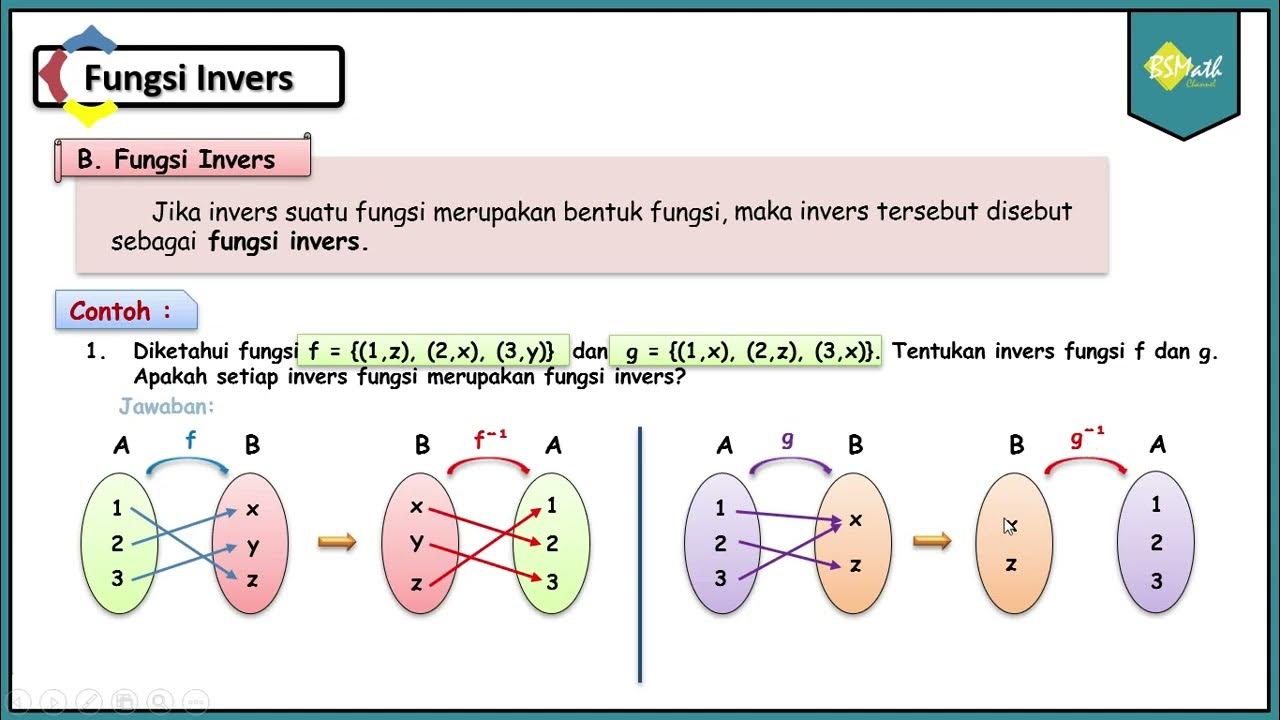
Fungsi Invers - Matematika SMA Kelas XI Kurikulum Merdeka

FUNÇÃO INVERSA | DEFINIÇÃO, LEI DE FORMAÇÃO, DIAGRAMA E EXERCÍCIOS | Ensino médio - Gis com Giz

Fungsi Invers Part 1 ||Materi, Soal & Pembahasan||

Apa itu Adverb of Manner? | Keterangan Cara dalam Grammar Bahasa Inggris

INVERSE OF ONE-TO-ONE FUNCTIONS || GRADE 11 GENERAL MATHEMATICS Q1
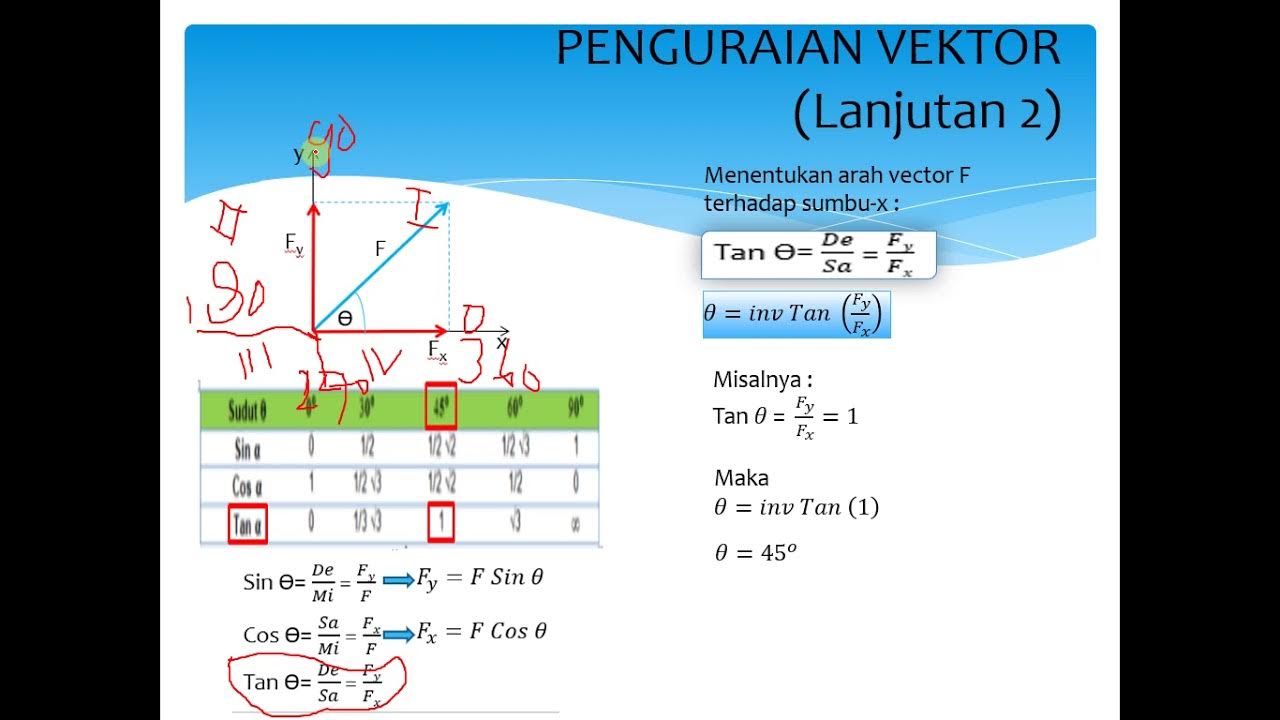
Penguraian Vektor (lanjutan)
5.0 / 5 (0 votes)