ST03: Rigid Body Equilibrium (1)
Summary
TLDRThis video script explains rigid body equilibrium, where the sum of forces and moments acting on a body must be zero for it to remain in a state of rest or constant velocity. The key concepts include understanding the forces in the X and Y directions, as well as calculating the moments that cause rotation. The script provides examples, such as an L-shaped bracket and a bar with unknown forces, to demonstrate how to apply the equilibrium equations. These principles are essential in mechanics and engineering for analyzing static structures and ensuring stability.
Takeaways
- 😀 A rigid body is a collection of connected particles, like a bar made up of many particles arranged in a specific manner.
- 😀 Static equilibrium in rigid bodies is achieved when the net force acting on it is zero, meaning the sum of forces in the X and Y directions must be zero.
- 😀 For static equilibrium to be maintained, a third condition involving moments (torques) must also be satisfied.
- 😀 Even if the net forces in both directions are zero, a rigid body can still rotate if moments are not balanced.
- 😀 A force acting at a distance from a particle causes a rotation, and this tendency is referred to as a moment.
- 😀 The magnitude of a moment is the product of the force (P) and the perpendicular distance (R) from the point of rotation: M = P * R.
- 😀 The direction of the moment is determined by the direction of rotation; counterclockwise moments are positive, and clockwise moments are negative.
- 😀 For a rigid body to be in equilibrium, the sum of the moments about any point must be zero.
- 😀 Equilibrium equations can be used to check if a body is in equilibrium or to determine unknown forces necessary to maintain equilibrium.
- 😀 In the example of the L-shaped bracket, the sum of forces in both X and Y directions was zero, and the moments about a chosen point also balanced, ensuring equilibrium.
- 😀 When solving for unknown forces in an equilibrium problem, a sign convention is essential for consistency, and solving the equilibrium equations allows determination of these unknown forces.
Q & A
What is a rigid body in the context of equilibrium?
-A rigid body is a collection of connected particles that maintain a fixed shape. For example, a bar can be viewed as a rigid body, where all the particles are arranged in a specific manner.
What are the conditions for a rigid body to be in static equilibrium?
-For a rigid body to be in static equilibrium, two conditions must be satisfied: the sum of the forces in both the X and Y directions must be zero, and the sum of the moments about any point must also be zero.
Why is it not enough for the forces in the X and Y directions to be zero for equilibrium?
-Because even if the forces in the X and Y directions are zero, the body can still rotate if there is an unbalanced moment. The third condition of equilibrium, which requires the sum of moments to be zero, is necessary to prevent rotation.
What is a moment, and how is it related to static equilibrium?
-A moment is the tendency of a force to cause rotation around a point. It is calculated as the product of the force and the perpendicular distance from the point to the line of action of the force. To maintain equilibrium, the net moment about any point must be zero.
How is the magnitude of a moment calculated?
-The magnitude of a moment is calculated using the formula M = P × R, where P is the force applied, and R is the perpendicular distance from the point of rotation to the line of action of the force.
Why does a force applied at a distance from a point cause a rotation in a rigid body?
-A force applied at a distance from a point causes a rotation because it creates a moment, which is the rotational effect produced by a force acting at a distance. The body rotates around the point where the moment is applied.
What is the direction of a moment when a force causes counterclockwise rotation?
-When a force causes counterclockwise rotation, the moment is considered to have a positive direction.
In the example of the L-shaped bracket, why is it important to calculate the moments about point B?
-It is important to calculate the moments about point B because the moments from the forces acting at a distance from point B help determine if the bracket is in equilibrium. By ensuring that the sum of the moments is zero, we confirm equilibrium.
What is a moment arm in the context of rigid body equilibrium?
-The moment arm is the perpendicular distance from the point of rotation to the line of action of the force. It is crucial in calculating the moment produced by a force.
In the example of the bar with unknown forces, how are the magnitudes of the unknown forces determined?
-The magnitudes of the unknown forces are determined by writing the equilibrium equations for the forces in the X and Y directions and the moments. By applying a sign convention and solving these equations, the unknown forces can be found.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
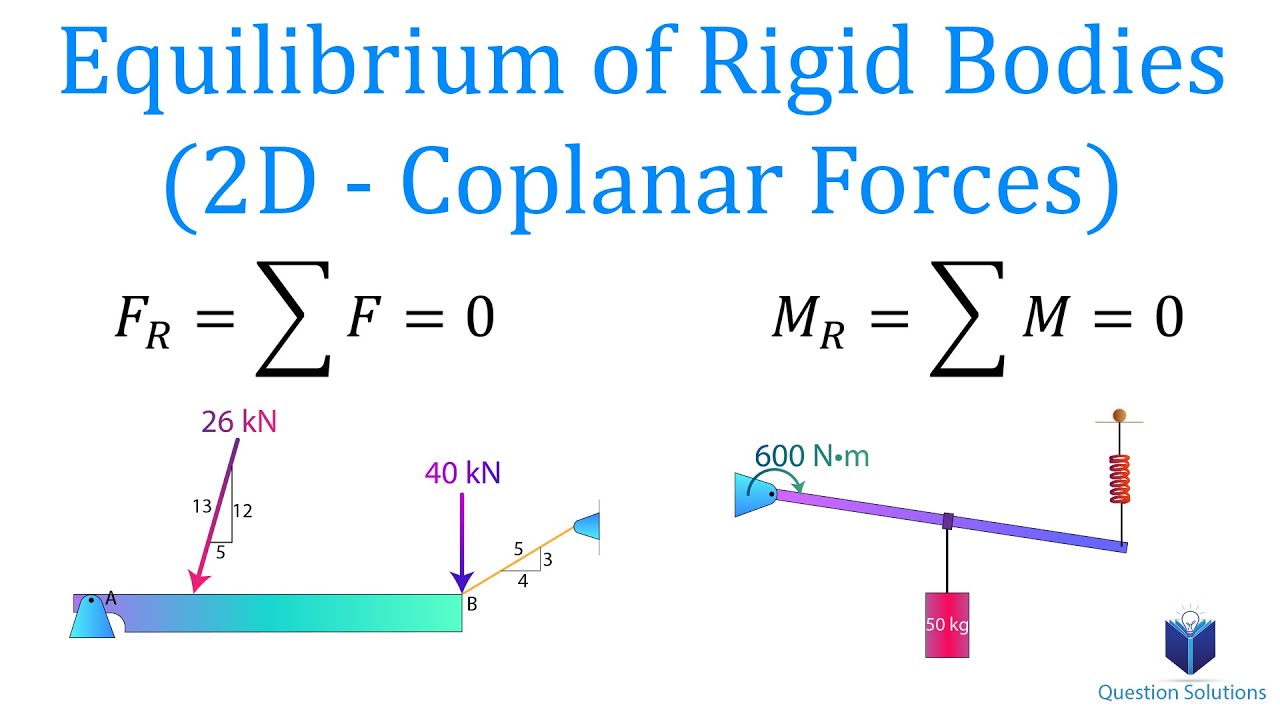
Equilibrium of Rigid Bodies (2D - Coplanar Forces) | Mechanics Statics | (Solved examples)

FISIKA KELAS XI || Kesetimbangan || DINAMIKA ROTASI & KESETIMBANGAN BENDA TEGAR

Video animasi hukum 1 newton
Balanced and Unbalanced Forces Science 8 Quarter 1 Week 1

Forces and Dynamics - free body diagrams - (IB Physics, AP, GCSE, A level)

Equilibrium of Rigid Bodies 3D force Systems | Mechanics Statics | (solved examples)
5.0 / 5 (0 votes)
