Konsep Variabel Acak Diskrit dan Kontinu
Summary
TLDRThis video explains the concept of random variables, distinguishing between discrete and continuous variables. It explores real-world examples such as counting the number of vehicles passing an intersection (discrete) and measuring the temperature of a patient (continuous). The script also covers how random variables are used in experiments, demonstrating their applications in various scenarios such as coin tosses, dice rolls, and statistical analysis. By breaking down complex ideas with clear examples, the video provides a solid foundation for understanding how different types of variables function in probability and statistics.
Takeaways
- 😀 Random variables are quantities that can change and take on different values, and are denoted using symbols like X, Y, Z.
- 😀 There are two types of random variables: discrete and continuous. Discrete variables take on specific values, while continuous variables can take on any value within an interval.
- 😀 Discrete variables are countable and typically represent things like the number of vehicles in a parking lot or the number of students in a class.
- 😀 Continuous variables represent measurements and can take any value within a range, such as weight, height, or temperature.
- 😀 Examples of discrete variables include the number of accidents per week, the number of children in a family, and the number of errors in a text.
- 😀 Continuous variables can represent things like temperature (which can have decimal values) or a person’s height (which can range between intervals like 160.1 to 160.2 cm).
- 😀 Discrete random variables typically have a finite or countable number of outcomes, like the results of rolling a die or flipping a coin.
- 😀 Continuous random variables have infinite possible values within an interval, such as the time taken to run a race or the weight of an object.
- 😀 The concept of random variables can be applied to various experiments, like flipping coins, rolling dice, or measuring people's heights.
- 😀 Random variables can be categorized as either discrete or continuous based on how their outcomes are measured—discrete for countable outcomes and continuous for measurable outcomes.
- 😀 Examples of calculating possible outcomes from random variables include summing the numbers on two dice, counting the number of heads in multiple coin flips, and determining the sum of values when rolling multiple dice.
Q & A
What is the definition of a variable in mathematics?
-A variable is a quantity that can change, meaning its value is not fixed and can take on multiple values, depending on the situation or conditions.
What is the difference between a discrete variable and a continuous variable?
-A discrete variable takes distinct, countable values (e.g., number of students in a class), while a continuous variable can take any value within a range and is typically measured (e.g., height or temperature).
Can you provide examples of discrete variables?
-Examples of discrete variables include the number of children in a family, the number of cars in a parking lot, or the number of accidents occurring in a week.
What are some examples of continuous variables?
-Examples of continuous variables include weight, height, temperature, and time, as these can take any value within a given range and are often measured rather than counted.
What is a random variable?
-A random variable is a variable whose value is determined by the outcome of a random event or experiment. It can be either discrete or continuous, depending on the nature of the experiment.
What distinguishes a discrete random variable from a continuous random variable?
-A discrete random variable has countable outcomes, such as the number of heads when flipping a coin multiple times, whereas a continuous random variable can take any value within a continuous range, such as the height of a person.
Can you give an example of a discrete random variable?
-An example of a discrete random variable is the number of heads when flipping two coins, as the possible outcomes are countable (0, 1, or 2 heads).
What would be an example of a continuous random variable?
-An example of a continuous random variable is the weight of an individual, as it can take any value within a range and be measured with precision (e.g., 65.5 kg, 70.2 kg).
Why are some variables considered continuous, even though they can be approximated by discrete values?
-Continuous variables are considered as such because their values can be measured with infinite precision within a given range, even if in practice, measurements are rounded or approximated. For example, temperature can be represented with decimal points, but it's theoretically continuous.
How can we determine whether a variable is discrete or continuous in a real-life scenario?
-To determine whether a variable is discrete or continuous, consider whether the values can be counted or measured. If the values are countable, like the number of people or events, it's discrete. If the values can vary smoothly over a range, like height or temperature, it’s continuous.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

Distribusi Normal • Part 1: Distribusi Peluang Variabel Acak Kontinu

VARIABEL ACAK DISKRIT: Pengertian dan Distribusi Peluang-nya!

Discrete and continuous random variables | Probability and Statistics | Khan Academy

Distribusi Binomial • Part 1: Variabel Acak
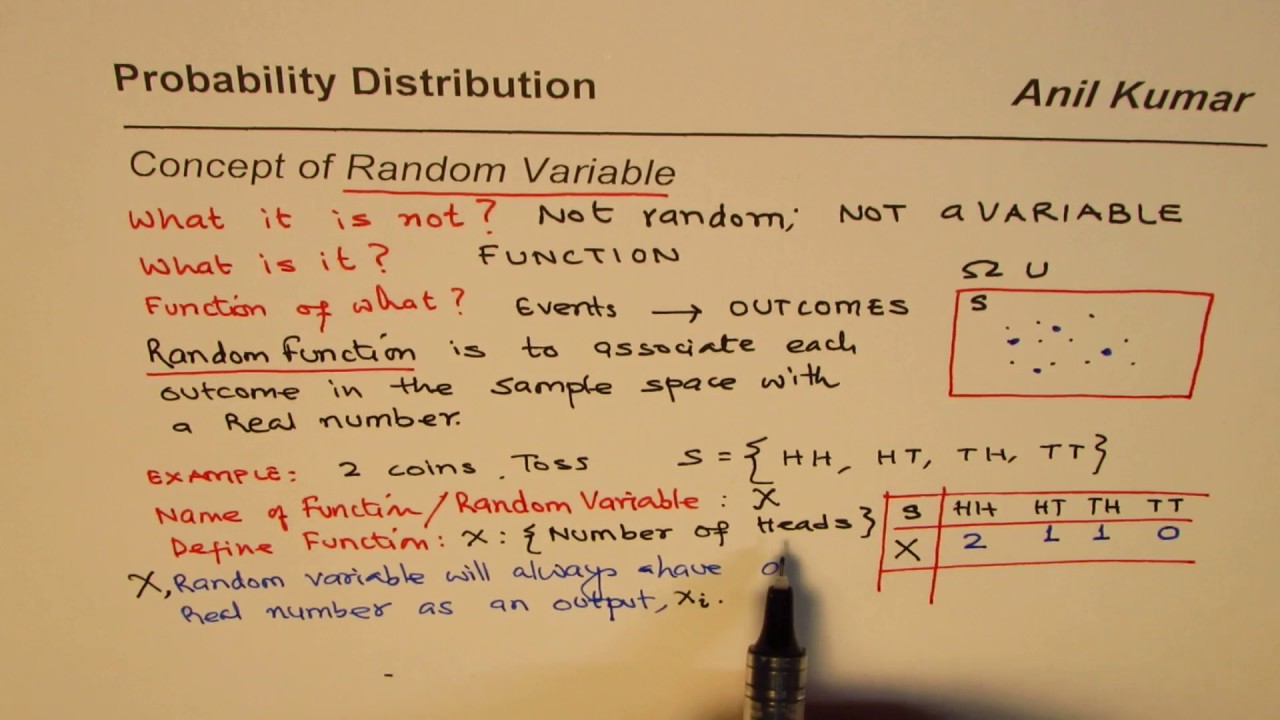
Introduction to Random Variables Probability Distribution
Random Variables - Grade 11 (Statistics and Probability) @MathTeacherGon
5.0 / 5 (0 votes)
