Random Variables - Grade 11 (Statistics and Probability) @MathTeacherGon
Summary
TLDRIn this Grade 11 statistics lesson, the teacher explains the concept of random variables, which are the outcomes of chance events that can be measured or counted. Using examples like tossing coins and rolling dice, the lesson demonstrates how to identify random variables and their possible values. Through two problems—one involving two coins and the other three—the teacher shows how to determine the values of random variables for the number of tails and heads, respectively. The video sets the stage for a future lesson on the difference between discrete and continuous random variables.
Takeaways
- 😀 A random variable is a result of a random event or experiment that can be measured or counted.
- 😀 Random variables are often represented using capital letters like X, Y, or T.
- 😀 Examples of experiments involving random variables include tossing coins, rolling dice, and drawing cards from a deck.
- 😀 The first step in analyzing a random variable is to determine the sample space, which is the set of all possible outcomes of an experiment.
- 😀 For the experiment of tossing two coins, the random variable T represents the number of tails that appear, and its possible values are 0, 1, and 2.
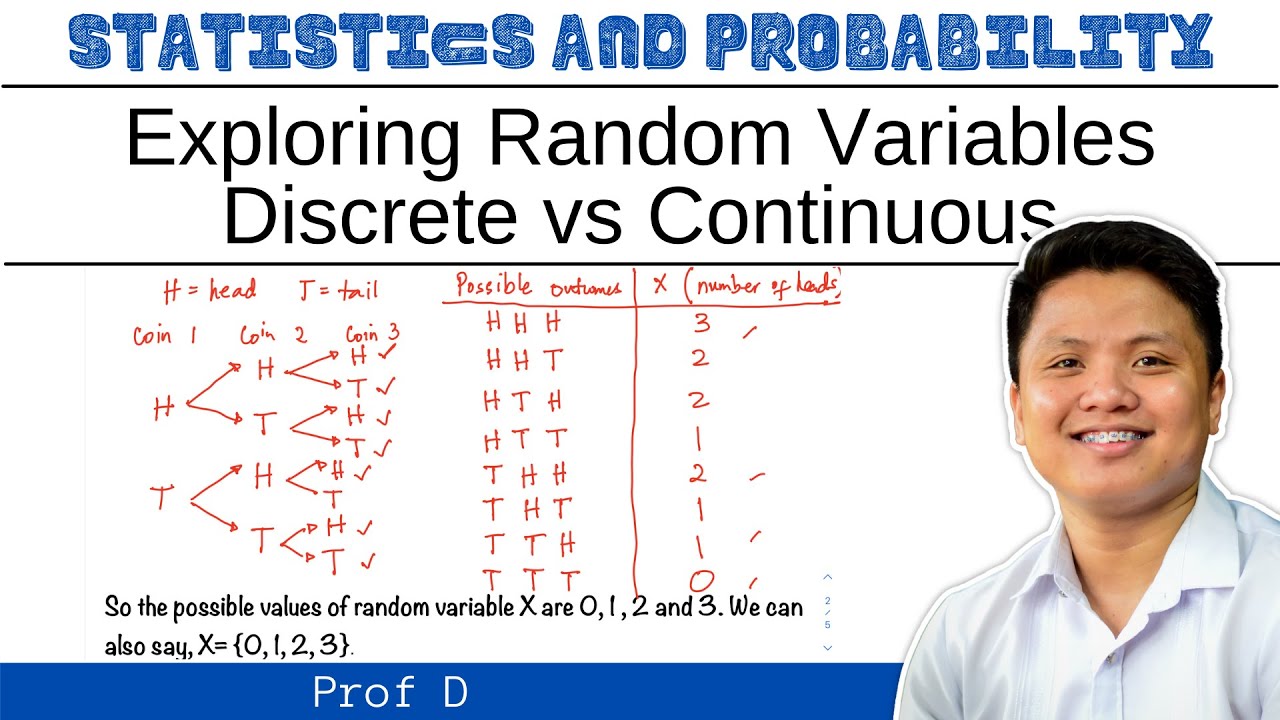
- 😀 When tossing three coins, the random variable Y represents the number of heads that appear, with possible values 0, 1, 2, and 3.
- 😀 The sample space for tossing two coins includes four possible outcomes: HH, HT, TH, and TT.
- 😀 In the two-coin example, the number of tails in the outcomes varies from 0 to 2.
- 😀 In the three-coin example, the number of heads in the outcomes can range from 0 to 3.
- 😀 Discrete random variables have specific, countable values (such as the number of heads or tails), while continuous random variables can take any value within a given range.
Q & A
What is a random variable?
-A random variable is the outcome of a random event or experiment that can either be measured or counted, such as the number of heads or tails in a coin toss.
How are random variables represented in statistics?
-Random variables are represented using capital letters, for example, 'T' for tails or 'Y' for heads in a coin toss experiment.
What is a sample space?
-The sample space is the set of all possible outcomes in an experiment. For example, in tossing two coins, the sample space includes outcomes like (Head, Head), (Head, Tail), (Tail, Head), and (Tail, Tail).
What does the random variable T represent in the example of tossing two coins?
-In the example of tossing two coins, the random variable T represents the number of tails that appear in the outcome.
How do we determine the values of a random variable in an experiment?
-To determine the values of a random variable, we analyze the possible outcomes in the sample space and count the number of specific events (like heads or tails) that occur in each outcome.
In the case of tossing two coins, what are the possible values of the random variable T?
-The possible values of the random variable T, which represents the number of tails, are 0, 1, and 2, based on the four possible outcomes (Head, Head), (Head, Tail), (Tail, Head), and (Tail, Tail).
What does the random variable Y represent in the example of tossing three coins?
-In the example of tossing three coins, the random variable Y represents the number of heads that appear in the outcome.
How many possible outcomes are there when tossing three coins?
-When tossing three coins, there are 8 possible outcomes, which include combinations of heads and tails in various orders.
What are the values of the random variable Y when tossing three coins?
-The values of the random variable Y, which represents the number of heads, are 0, 1, 2, and 3, depending on the outcome of the tosses.
What is the difference between discrete and continuous random variables?
-Discrete random variables take on a finite number of distinct values, such as the number of heads or tails in a coin toss. Continuous random variables, on the other hand, can take any value within a range, like the exact height or temperature in an experiment.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade Now5.0 / 5 (0 votes)