Introduction to tensile stress
Summary
TLDRIn this educational video, the concept of stress is introduced as force per unit area, using a relatable scenario of two ropes with different diameters to illustrate the idea. The video explains how stress increases with a smaller cross-sectional area and introduces 'ultimate strength' as the maximum stress a material can withstand before breaking. Through a practical example involving ropes and weights, the lesson demonstrates how to calculate the breaking point of objects made of the same material without physical testing, highlighting the importance of understanding material properties in engineering and physics.
Takeaways
- 😀 The video is an educational lesson from dapps Academy on the concept of stress and its relation to material failure.
- 🔍 The example of two strings with different diameters is used to illustrate the concept of stress and how it affects the breaking point of an object.
- 📏 The first string with a 0.1-inch diameter breaks under 100 pounds, which is used to calculate the ultimate strength of the material.
- 📐 Stress is defined as force per unit area, and understanding this helps in predicting when an object will fail under pressure.
- 🌳 The video uses the analogy of choosing between a thick and thin rope to climb down from a tree, emphasizing the intuitive understanding of stress.
- 🔢 The formula for stress is force divided by the cross-sectional area, which is crucial in determining the stress a material experiences.
- 🔍 The video explains that the units for stress can be pounds per square inch (PSI) or Newtons per square meter (Pascals).
- 🏗️ Ultimate strength is the maximum stress a material can withstand before breaking and is a material property.
- 📉 The ultimate strength of the material is calculated using the area of the cross-section of the 0.1-inch diameter string and the force at which it broke.
- 🔄 Since both strings are made of the same material, they share the same ultimate strength, which is used to calculate the breaking force of the 0.2-inch diameter string.
- 📚 The video concludes by solving the initial riddle, showing that the 0.2-inch diameter string can hold four times the weight (400 pounds) before breaking, due to its larger cross-sectional area.
Q & A
What is the main topic of the lesson in the provided transcript?
-The main topic of the lesson is understanding stress and determining when an object will break.
What is the first vocabulary word introduced in the script, and what does it describe?
-The first vocabulary word introduced is 'stress,' which describes force per unit area.
Why would a person trust a thick rope more than a thin one when climbing down from a tree?
-A person would trust a thick rope more because it has a larger cross-sectional area, resulting in less stress compared to a thin rope when the same force is applied.
What is the unit for stress commonly used in the script?
-The common units for stress mentioned in the script are PSI (pounds per square inch) and Pascal (Newtons over square meters).
What is 'ultimate strength' in the context of materials?
-'Ultimate strength' is the maximum amount of stress a material can take before it breaks.
What is the method to calculate the cross-sectional area of a circular object?
-The cross-sectional area of a circular object is calculated using the formula π times the radius squared (A = πr^2).
How did the script determine the ultimate strength of the 0.1 inch diameter string?
-The ultimate strength was determined by dividing the maximum force that broke the string (100 pounds) by its cross-sectional area (.0025π square inches).
What is the relationship between the diameters of the two strings and the force required to break them?
-The second string with a diameter of 0.2 inches can withstand a force four times greater than the first string (0.1 inch diameter) because its cross-sectional area is four times larger.
How much weight can the 0.2 inch diameter string hold before breaking, according to the script?
-The 0.2 inch diameter string can hold 400 pounds before breaking, which is four times the weight that broke the 0.1 inch diameter string.
What is the significance of the ultimate strength being a material property?
-The significance is that two objects made of the same material will have the same ultimate strength, regardless of their size or shape.
What is the humorous twist at the end of the script, and how does it relate to the lesson?
-The humorous twist is that the video, expected to have a punchline for April Fools' Day, instead provides a lesson on physics, which is an unexpected yet educational outcome.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
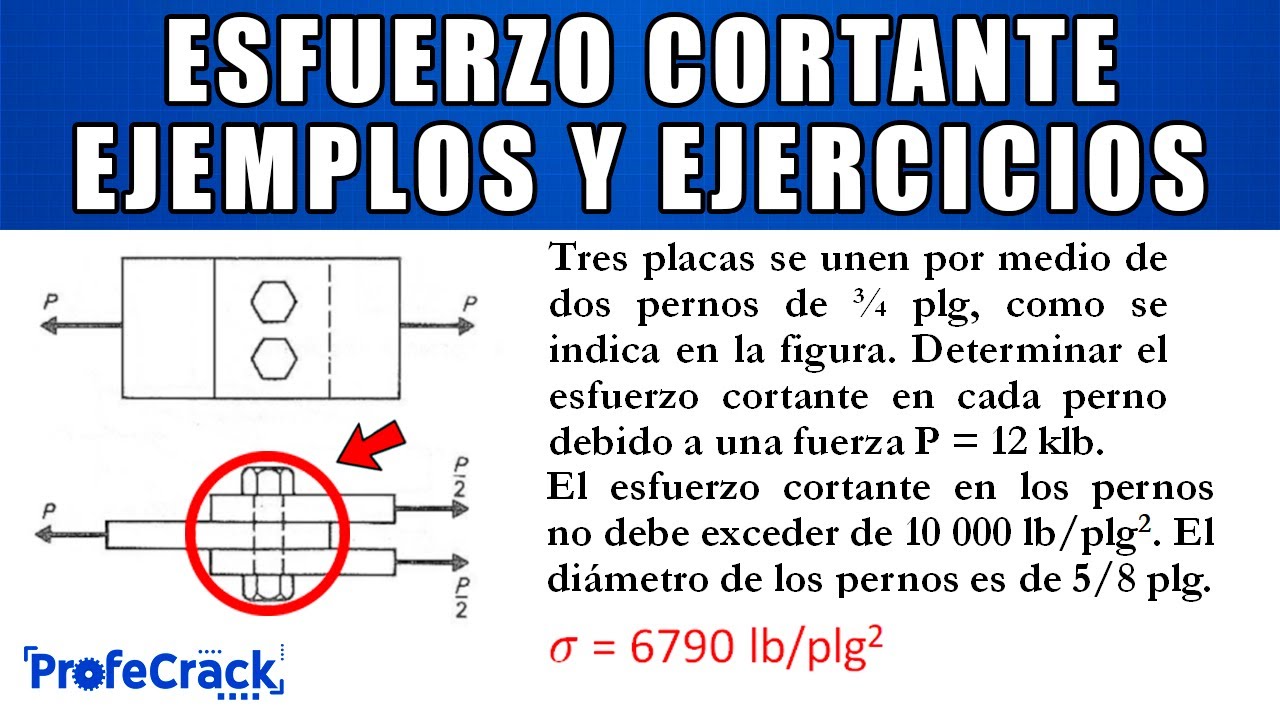
Esfuerzo Cortante: Ejemplos y Ejercicios Resueltos ✅ [Pernos y Placas] Mecánica de Materiales Fácil

Materials Science Mechanical Engineering - Part 1 Stress and Strain Explained

4.3b Pressure as Force per unit Area | AS Pressure | Cambridge A Level Physics

Billy's Pressure Dream

Hydrostatic Pressure (Fluid Mechanics - Lesson 3)

IGCSE Physics [Syllabus 1.8] Pressure
5.0 / 5 (0 votes)