TEORIA Moto di una carica in un campo magnetico uniforme AMALDI ZANICHELLI

Summary

This video explains how electric charges move in uniform linear motion and how their motion changes when a magnetic field is introduced. The charges experience a magnetic force that alters their direction but not their speed, resulting in circular motion. The work done by the magnetic force is zero because the force is always perpendicular to the displacement. The script also presents the equation for the radius of the circular motion of a charged particle in a magnetic field, explaining its relationship with mass, speed, charge, and the magnetic field strength. The force acting on the particle is known as the Lorentz force.

Takeaways

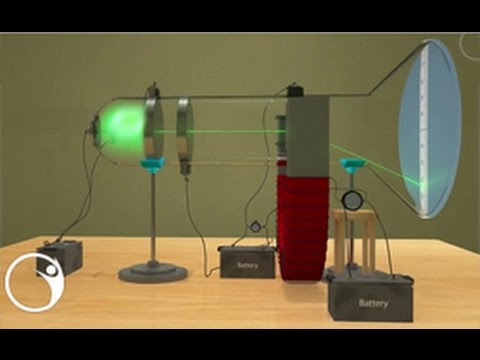
• 😀 The electric charges moving across the screen are initially in uniform linear motion.
• 😀 When a uniform magnetic field, perpendicular to the velocity of the particles, is introduced, the motion of the charges changes.
• 😀 The charged particles now experience a magnetic force, causing them to move in uniform circular motion.
• 😀 The magnetic force on the particle does not change the magnitude of its velocity, only its direction.
• 😀 Since the kinetic energy of the particle does not change, the work done by the magnetic force is zero.
• 😀 The magnetic force is always perpendicular to both the displacement and the velocity of the charged particle.
• 😀 The magnetic force acts as the centripetal force that drives the circular motion of the charged particle in the magnetic field.
• 😀 The radius of the circular trajectory depends on the mass, velocity, charge, and magnetic field strength.
• 😀 The radius (r) of the circular orbit is directly proportional to the particle's mass (m) and velocity (v), and inversely proportional to its charge (q) and the magnetic field strength (B).
• 😀 By substituting the expression for the radius into the centripetal force formula, we obtain the magnetic force on a charged particle moving perpendicular to the magnetic field.
• 😀 The magnetic force, which is perpendicular to both the velocity and the magnetic field, is called the Lorentz force.

Q & A

What type of motion do the electric charges initially have in the script?

The electric charges are initially moving in uniform linear motion.

What happens when a uniform magnetic field is suddenly applied?

When a uniform magnetic field is suddenly applied perpendicular to the velocity of the particles, the motion of the charges changes to uniform circular motion.

What effect does the magnetic force have on the particle's speed?

The magnetic force does not change the speed (magnitude of velocity) of the particle, but it alters the direction of the velocity, making it move in a circular path.

Why does the magnetic force not perform work on the charged particle?

The magnetic force does no work on the charged particle because it is always perpendicular to the displacement and the velocity of the charge. As a result, it does not change the kinetic energy of the particle.

What is the role of the magnetic force in the circular motion of a charged particle?

The magnetic force acts as the centripetal force that keeps the charged particle in circular motion, constantly changing the direction of the particle's velocity but not its speed.

How is the radius of the circular trajectory of the particle related to its properties?

The radius of the circular trajectory (r) is directly proportional to the mass (m) and the velocity (v) of the particle, and inversely proportional to the charge (q) and the strength of the magnetic field (B).

What formula is used to calculate the radius of the circular motion of a charged particle in a magnetic field?

The radius r of the circular motion is given by the formula r = (m * v) / (q * B), where m is the mass, v is the velocity, q is the charge, and B is the magnetic field strength.

What happens when you substitute the expression for the radius into the formula for centripetal force?

Substituting the radius expression into the formula for centripetal force gives the magnitude of the magnetic force acting on the charged particle, which is perpendicular to both the velocity and the magnetic field.

What is the name of the force that acts on a charged particle in a magnetic field?

The force acting on a charged particle in a magnetic field is called the Lorentz force.

How does the Lorentz force relate to the direction of motion and the magnetic field?

The Lorentz force is always perpendicular to both the velocity of the particle and the magnetic field, ensuring that it does not alter the speed but only changes the direction of motion.