Trigonometri Matematika Kelas 10 • Part 4: Perbandingan Trigonometri Sudut Istimewa

Summary

This video provides an in-depth explanation of trigonometric ratios for special angles, focusing on angles of 0°, 30°, 45°, 60°, and 90°. The presenter walks through key concepts using examples of right-angled triangles, illustrating the sine, cosine, tangent, cotangent, secant, and cosecant functions for these angles. Techniques for memorizing trigonometric values and relationships between the functions are also discussed, making it easier for students to recall them. The video concludes with a visual summary of the trigonometric values, ensuring that learners can apply these concepts effectively in future calculations.

Takeaways

• 😀 Trigonometric ratios for special angles (0°, 30°, 45°, 60°, 90°) are crucial to understand in trigonometry.
• 😀 The basic trigonometric functions include sine (sin), cosine (cos), tangent (tan), cotangent (cot), secant (sec), and cosecant (csc).
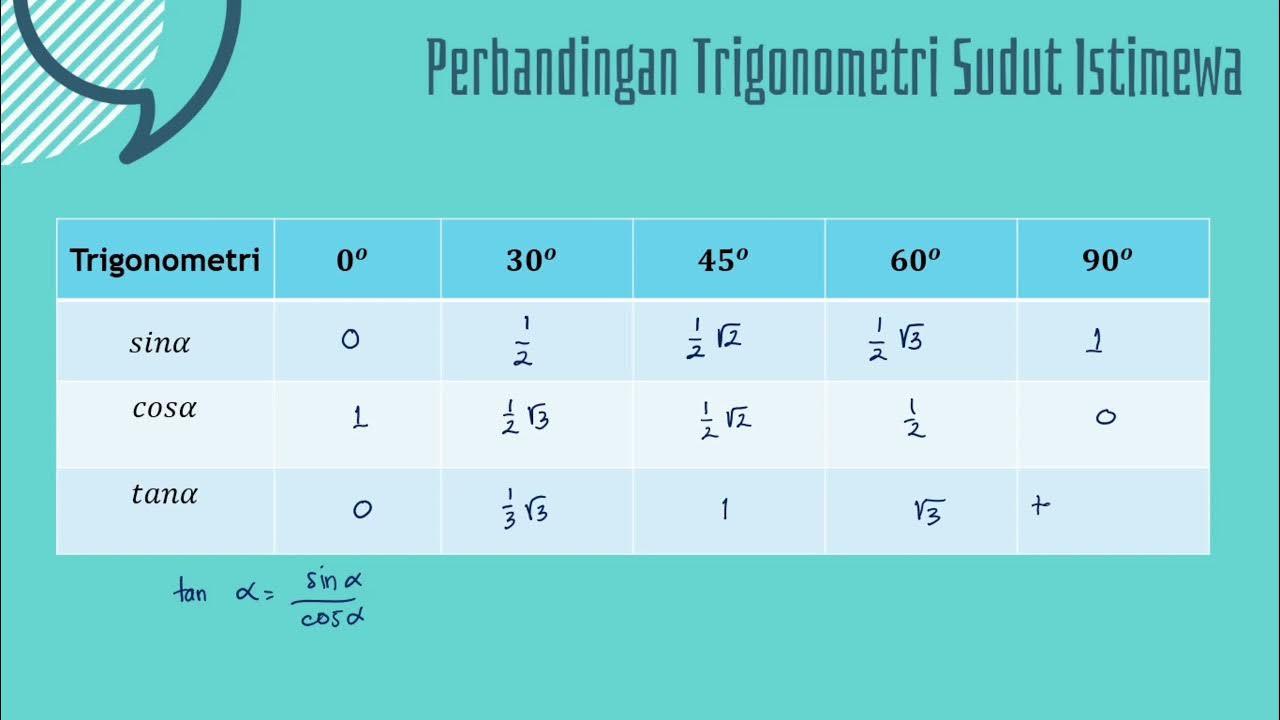
• 😀 For 30°, sin 30° = 1/2, cos 30° = √3/2, tan 30° = 1/√3, and their corresponding cot, sec, and csc values are √3, 2/√3, and 2, respectively.
• 😀 For 45°, sin 45° = 1/√2, cos 45° = 1/√2, tan 45° = 1, with cot 45° = 1, sec 45° = √2, and csc 45° = √2.
• 😀 For 60°, sin 60° = √3/2, cos 60° = 1/2, tan 60° = √3, cot 60° = 1/√3, sec 60° = 2, and csc 60° = 2/√3.
• 😀 For 0° and 90°, sin 0° = 0, cos 0° = 1, tan 0° = 0, while sin 90° = 1, cos 90° = 0, and tan 90° is undefined.
• 😀 Memorization tip: sine values follow a pattern: 0, 1/2, 1/√2, √3/2, and 1 for angles 0°, 30°, 45°, 60°, and 90° respectively.
• 😀 Cosine values are the reverse of sine values, making them easy to remember once you know the sine values.
• 😀 Tangent values can be calculated by dividing the sine value by the cosine value for each corresponding angle.
• 😀 Cotangent, secant, and cosecant are simply the reciprocals of tangent, cosine, and sine, respectively, and can be derived from them.

Q & A

What is the main topic of the video?

The main topic of the video is about trigonometric ratios of special angles, particularly for angles 0°, 30°, 45°, 60°, and 90°, and how to remember them effectively.

What are the key trigonometric ratios discussed in the video?

The key trigonometric ratios discussed are sine (sin), cosine (cos), tangent (tan), cotangent (cot), secant (sec), and cosecant (csc).

How are the trigonometric ratios derived for a right triangle?

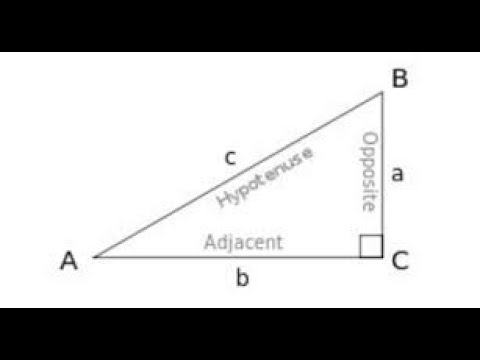
The trigonometric ratios are derived from the relationships between the sides of a right triangle: sine is the ratio of the opposite side to the hypotenuse, cosine is the ratio of the adjacent side to the hypotenuse, tangent is the ratio of the opposite side to the adjacent side, and so on for the other ratios.

What are the values of the trigonometric functions for 30°?

For 30°: sin(30°) = 1/2, cos(30°) = √3/2, tan(30°) = 1/√3, cot(30°) = √3, sec(30°) = 2/√3, and csc(30°) = 2.

What is the significance of special right triangles like 30°-60°-90° and 45°-45°-90°?

These special right triangles have fixed ratios for their sides, which make it easier to calculate the values of the trigonometric functions for the corresponding angles without using a calculator.

What is the easiest way to remember the values of sine for special angles?

The easiest way to remember the values of sine for special angles is by following a pattern: sin(0°) = 0, sin(30°) = 1/2, sin(45°) = √2/2, sin(60°) = √3/2, and sin(90°) = 1.

How are the values of cosine related to sine for special angles?

The values of cosine for special angles are the reverse of the sine values. For example, cos(0°) = 1, cos(30°) = √3/2, cos(45°) = √2/2, cos(60°) = 1/2, and cos(90°) = 0.

What happens to the tangent ratio at 0°?

At 0°, the tangent is 0 because it is the ratio of the opposite side to the adjacent side, and the opposite side is 0 at this angle.

What are the values of the trigonometric functions for 90°?

For 90°: sin(90°) = 1, cos(90°) = 0, tan(90°) is undefined (because dividing by 0 is not possible), cot(90°) = 0, sec(90°) is undefined, and csc(90°) = 1.

What is the importance of rationalizing trigonometric ratios in the video?

Rationalizing trigonometric ratios, such as multiplying by the square root of 3 in certain cases, helps to simplify the expressions and make them easier to work with.