TRIGONOMETRI SUDUT ISTIMEWA
Summary
TLDRThis video covers the topic of special right triangles in trigonometry, explaining their key properties and how to apply them in real-life situations. The instructor breaks down three specific types of special triangles: 30-60-90, 45-90, and 37-53-90 triangles, providing formulas and simple methods to quickly solve for side lengths without complex calculations. The video highlights important trigonometric ratios for these angles, such as sine, cosine, and tangent. The content is designed to help viewers efficiently solve problems involving these special triangles, offering practical examples and exercises for further practice.
Takeaways
- 😀 Triangular ratios like those in special triangles can be easily memorized, helping with trigonometric problem-solving in real-life situations.
- 😀 A special triangle has fixed side ratios based on its angle measures, making it easy to calculate sides without complicated formulas.
- 😀 The first type of special triangle discussed is a 30-60-90 triangle, where the ratio of the sides is 'A', 'A√3', and '2A'.
- 😀 In a 30-60-90 triangle, if the shortest side is A, the longest side is always 2A, and the middle side is A√3.
- 😀 The second type of special triangle is the 45-45-90 triangle, where the sides are in the ratio 'A', 'A', and 'A√2'.
- 😀 A 45-45-90 triangle is an isosceles right triangle, and its side lengths are easily calculated by multiplying the shorter side by √2.
- 😀 The third type is the 3-4-5 triangle, which is equivalent to a 37-53-90 triangle in trigonometry. The side lengths follow the ratio '3A', '4A', and '5A'.
- 😀 In a 3-4-5 triangle, if one side is known (e.g., 3), the other sides can be easily found by multiplying by A.
- 😀 Using special triangles, you can quickly determine trigonometric ratios like sine, cosine, and tangent for specific angles like 30°, 45°, and 60°.
- 😀 The application of special triangles simplifies trigonometric problem-solving, allowing for quick calculations of unknown sides and angles in right-angled triangles.
Q & A
What is the main focus of the video script?
-The video focuses on explaining 'special triangles' in trigonometry, particularly three specific types of right-angled triangles (30-60-90, 45-90, and 37-53-90) and how to use their properties for solving trigonometric problems.
What makes a triangle 'special' in trigonometry?
-A 'special' triangle in trigonometry refers to a triangle where the side ratios and angles are simple enough to memorize. These properties allow for quick, easy calculations without needing to use complex formulas.
How are the sides of a 30-60-90 triangle related?
-In a 30-60-90 triangle, if the shortest side is 'A', the hypotenuse is '2A', and the remaining side (opposite the 60° angle) is 'A√3'.
Can you give an example of a 30-60-90 triangle with specific side lengths?
-If the shortest side (opposite the 30° angle) is 5, then the hypotenuse will be 10, and the side opposite the 60° angle will be 5√3.
What are the important trigonometric ratios for the 30° and 60° angles?
-For a 30° angle, sin(30) = 1/2, cos(30) = √3/2, and tan(30) = 1/√3. For a 60° angle, sin(60) = √3/2, cos(60) = 1/2, and tan(60) = √3.
What defines a 45-90 triangle, and what are its side ratios?
-A 45-90 triangle is an isosceles right triangle where the two legs are equal in length, denoted as 'A'. The hypotenuse is 'A√2'.
How would you calculate the hypotenuse of a 45-90 triangle if the legs are 6?
-In a 45-90 triangle, if each leg is 6, the hypotenuse would be 6√2, based on the property of these triangles.
What is a 37-53-90 triangle, and how is it related to the 3-4-5 triangle?
-A 37-53-90 triangle is a right triangle where the angles are approximately 37°, 53°, and 90°. Its side ratios are similar to the well-known 3-4-5 triangle, but scaled up or down by a factor 'A'.
What is the significance of the 3-4-5 triangle in trigonometry?
-The 3-4-5 triangle is a commonly used example of a right-angled triangle with integer sides. The side ratios are useful for solving problems involving trigonometric ratios, and it is also considered a special triangle.
How can you quickly calculate side lengths in a special triangle without using complex formulas?
-You can use memorized side ratios based on the triangle's angle measures. For example, in a 30-60-90 triangle, the side ratios are 'A', 'A√3', and '2A', allowing for quick calculation without detailed formula work.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Perbandingan Trigonometri Pada Segitiga SIku-siku #Trigonometri
Matematika SMA - Trigonometri (1) - Pengenalan Trigonometri, Perbandingan Trigonometri (A)
Mariano Nathanael - Fisika Kelas X - SMAN 2 Bandung - Vektor 1 - Juni 2022 #PKGTKJABAR

Vektor Fisika • Part 1: Pengantar Trigonometri Dasar
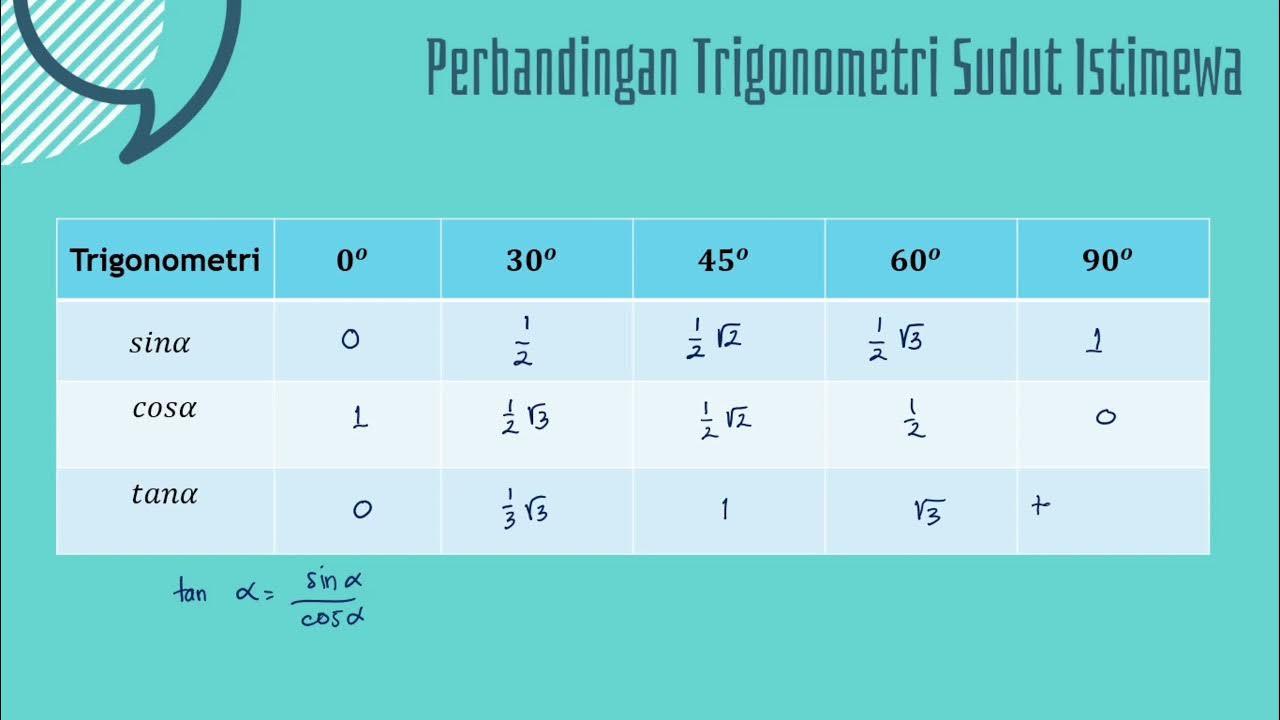
Perbandingan Trigonometri Sudut Istimewa

Area of a Triangle, Given 3 Sides, Heron's Formula
5.0 / 5 (0 votes)