Metode Clapeyron Teori

Summary

In this video lecture, the instructor discusses the classical method of Three-Moment Equations (also known as Clapeyron's Method) used to calculate the bending moments in continuous beams with static indeterminacy. The lecture covers the derivation of the equations, the concept of elastic curves, and the relationship between moments at consecutive supports. Through detailed examples, the method is explained for both simple and fixed supports, with an emphasis on solving static indeterminate structures. The instructor also touches on the use of moment areas and provides a detailed walkthrough of calculations using this approach.

Takeaways

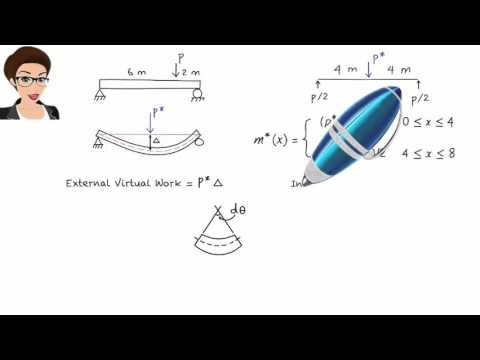
• 😀 The lecture focuses on the 'Three Moment Equation,' a classical method for calculating moments in structural analysis, particularly in continuous beams.
• 😀 The Three Moment Equation is derived from the relationship between bending moments at three consecutive supports in a structure.
• 😀 This method is part of a broader set of techniques for analyzing indeterminate structures, with other methods including the Cross Method and Slope-Deflection Equations.
• 😀 The Three Moment Equation was discovered by Clapeyron in 1857 and is sometimes referred to as Clapeyron's Theorem.
• 😀 The core concept of the Three Moment Equation revolves around the continuity of the elastic curve at the supports of the beam.
• 😀 The lecture explains the process of calculating the deflection and bending moments using various geometric relationships, such as comparing areas of triangles formed in the beam diagram.
• 😀 The script details the use of the method with a simple beam support diagram to show how to calculate moments at different points.
• 😀 An example is provided, discussing how to find the centroid of areas formed by triangular shapes in the moment diagram, aiding in the calculation of deflections and moments.
• 😀 The derivation process for the moment equations involves comparing the deflections at different points on the beam and using equilibrium equations to simplify calculations.
• 😀 The method concludes with a set of derived equations for calculating the reactions and moments at different supports, which can then be used to analyze more complex structures.

Q & A

What is the purpose of the three-moment equation discussed in the lecture?

The three-moment equation is used to calculate the bending moments at the supports of continuous beams in statically indeterminate structures. It helps in solving for the unknown moments by relating them through equilibrium and deflection principles.

How is the three-moment equation derived?

The three-moment equation is derived from the relationship between the bending moments at three consecutive supports of a continuous beam. It involves the continuity of the elastic curve and uses the equilibrium conditions to form the equation.

What are the key components involved in Clapeyron’s method for structural analysis?

Clapeyron’s method involves calculating the bending moments and deflections at various supports of a continuous beam, using the three-moment equation, moment-curvature relationships, and principles of equilibrium and superposition.

What is the significance of the terms 'moment' and 'deflection' in the context of the lecture?

In structural analysis, 'moment' refers to the bending forces at supports or points along the beam, while 'deflection' refers to the displacement of the beam from its original position due to those moments. Both are critical for analyzing the behavior of statically indeterminate structures.

What method is compared to Clapeyron’s method in the lecture?

The lecture compares Clapeyron’s method to other classical methods such as the 'Cross Method' and the 'Slope-Deflection Method.' These are all techniques for solving statically indeterminate structures.

What role do 'areas' under the moment-curvature curve play in the calculation?

The areas under the moment-curvature curve represent the work done by the bending moments and help in calculating the deflections and moments in the beam. These areas are used in the moment-area method to determine displacements and reactions.

How does the method account for different types of supports, like pinned or fixed supports?

The method accounts for different supports by analyzing how each type of support (such as fixed or roller supports) influences the bending moment distribution and deflection. For example, fixed supports have specific moments associated with them, which are incorporated into the equations.

What are the key assumptions in using Clapeyron’s method for structural analysis?

Key assumptions include the linear elasticity of the beam material, the beam's ability to resist bending without failure, and the use of static equilibrium conditions to analyze the structure. The method also assumes that the structure is continuous and statically indeterminate.

How does the lecture describe the application of the three-moment equation in practice?

The lecture explains the practical application of the three-moment equation by using it to calculate the bending moments at various supports in a continuous beam under specific loading conditions. It involves solving a system of equations that relate the moments at each support.

What is the significance of the term 'elastic curve' in the derivation of the three-moment equation?

The 'elastic curve' represents the deflection shape of the beam under loading. In the derivation of the three-moment equation, the continuity of this curve at consecutive supports is used to relate the bending moments and solve for unknown values.