Lentur Murni Pada Balok....Masa Sih Ada??

Summary

In this lecture on Mechanics of Materials, Ibu Riris introduces the concept of pure bending in beams. She covers topics like pure bending, uniform bending, and strain calculations in beams under load. The session focuses on cantilever beams subjected to bending moments, explaining how the beam deflects and forms a curve, known as the deflection curve. The discussion also explores the neutral axis of the beam, where the stress is zero, and how tensile and compressive stresses vary along the beam. Mathematical equations for beam deformation and curvature are also covered, helping students understand beam bending behavior in structural analysis.

Takeaways

• 😀 The topic of the lecture is pure bending on beams in the field of material mechanics.
• 😀 The lecture is divided into two main sections: pure bending and uniform bending, as well as strain calculations in beams.
• 😀 The first image shows a cantilever beam under load, which will bend and form a deflection curve.
• 😀 The deflection curve occurs in the XW plane, also called the plane of bending.
• 😀 Pure bending involves a beam that bends under a load, where the beam changes from a straight shape to a curved one.
• 😀 The relationship between curvature (represented by the radius) and strain and stress in the beam is addressed.
• 😀 The curvature of a beam is positive when the beam curves upward and negative when it curves downward.
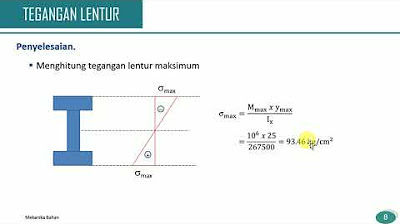
• 😀 The neutral axis in the beam experiences no stress, while the upper and lower parts of the beam undergo compression and tension, respectively.
• 😀 The lecture also discusses longitudinal strain, which can be analyzed by examining the curvature and deformation of the beam.
• 😀 The formula for the strain in the beam relates the deflection, radius, and the original length of the beam, as presented in the equations discussed.

Q & A

What is the main topic discussed in the transcript?

The main topic discussed is the study of pure bending in beams, specifically focusing on the deflection curve and the stresses and strains that occur in beams under bending forces.

What are the two main subtopics covered in this session?

The two main subtopics are pure bending and uniform bending, as well as strain calculations and deflection curves in beams.

What is meant by 'pure bending' of a beam?

'Pure bending' refers to the condition where a beam bends under a moment without any external shear forces acting on it. This causes the beam to deform into a curve, with the bending moment remaining constant along the length of the beam.

What does the deflection curve of a beam represent?

The deflection curve represents the shape or deformation of a beam when it is subjected to bending forces. It shows how the beam curves as a result of these forces, with the beam's original straight line turning into a curved shape.

What is the significance of the 'neutral axis' in bending?

The neutral axis is the line within the beam where the material experiences no longitudinal strain or stress during bending. Above this axis, the material is compressed, and below it, the material is stretched.

What is the formula for curvature in a beam?

The formula for curvature (k) in a beam is given by k = 1/R, where R is the radius of curvature. The curvature is used to determine the amount of bending in the beam.

How are stresses and strains related to deflection in a beam?

The stresses and strains in a beam under bending are directly proportional to the curvature and the amount of deflection. The greater the deflection, the higher the strain and stress experienced by the beam.

What does the term 'momen lentur' (bending moment) refer to in this context?

The 'momen lentur' or bending moment refers to the moment or torque applied to the beam, causing it to bend. It is a critical factor in determining the amount of deflection and the internal stresses within the beam.

What happens to the beam when it experiences bending, according to the script?

When the beam experiences bending, its shape changes from straight to curved. This deformation occurs because of the applied load, and the beam's top and bottom surfaces experience different types of stresses: compression on the top and tension on the bottom.

How is the length of a beam segment affected by bending?

The length of a beam segment is affected by bending in that the length of the neutral axis (L1) changes due to deformation. The beam's original length is altered based on the curvature of the bending, which is mathematically represented by the formula L1 = (roll - y) / DX.