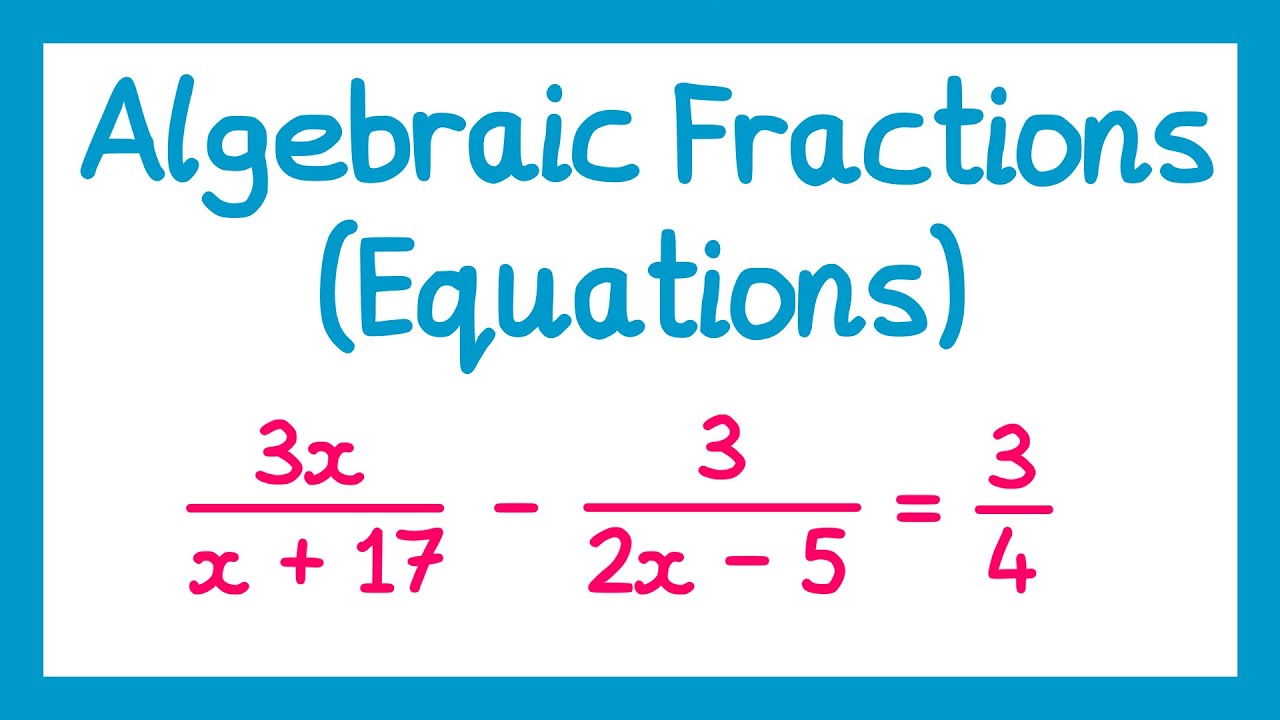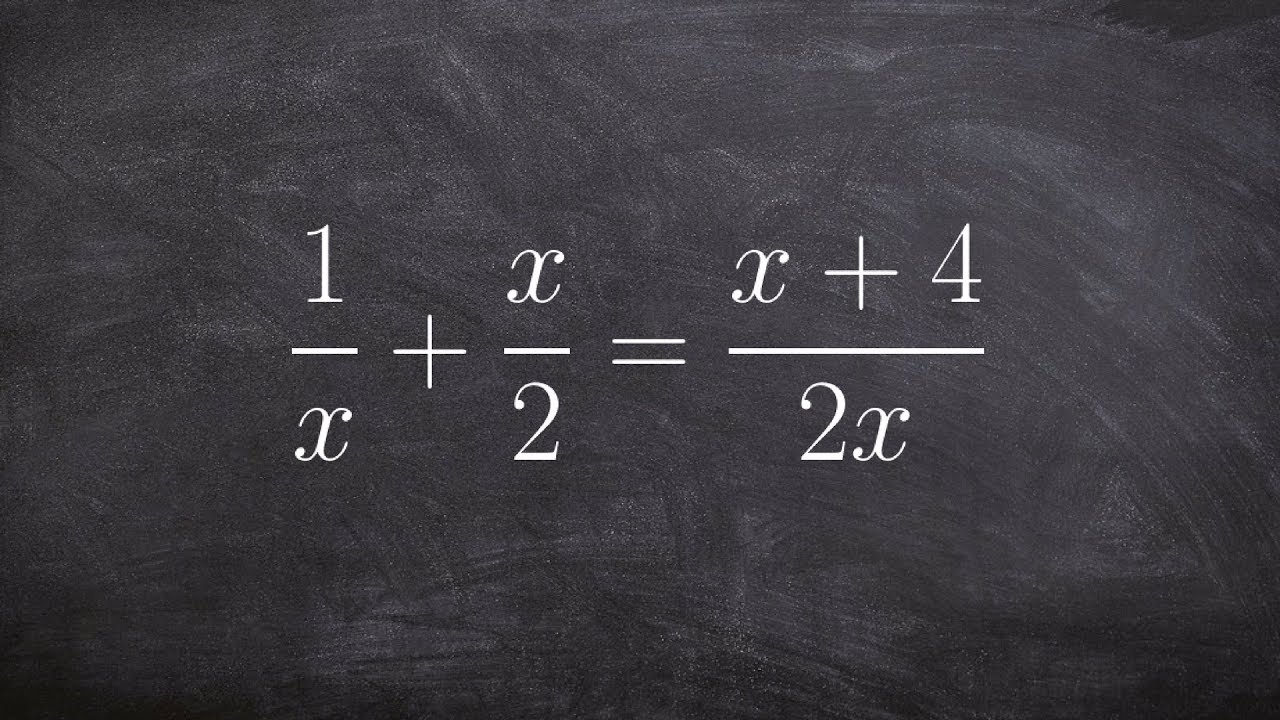Rational Equations

Summary

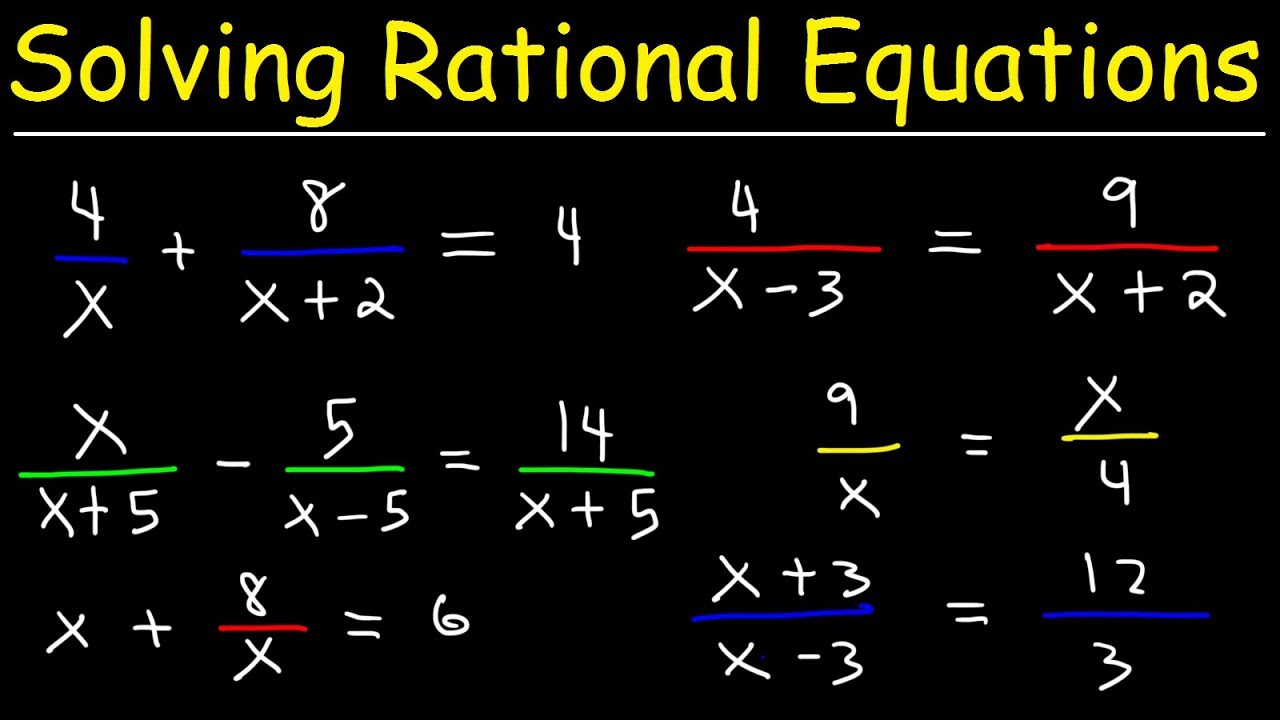
This instructional video script focuses on solving rational equations, which are equations involving fractions with variables. The approach emphasized is to gather all fractions on one side with a common denominator before proceeding with the solution. The script explains the importance of avoiding division by zero and provides step-by-step examples to illustrate the process of finding a common denominator and solving for the variable. It also highlights the significance of excluding values that would result in a zero denominator. The examples gradually increase in complexity, demonstrating how to handle different scenarios, including when denominators are factored or when there are no additions or subtractions involved. The goal is not only to solve the equations but also to prepare for tackling rational inequalities and graphing rational functions.

Takeaways

• 🧩 Rational equations involve fractions with variables, also known as rational expressions.
• ❌ Division by zero is undefined in mathematics, which is a crucial concept when solving rational equations.
• 🔍 If the numerator of a fraction is zero and the denominator is not zero, the expression evaluates to zero.
• 🚫 Exclude values that would make the denominator zero, as these cannot be solutions to the equation.
• 📚 Finding a common denominator is essential for combining fractions and solving rational equations.
• 📉 Rational equations can be solved by moving all fractions to one side of the equation and setting the sum equal to zero.
• 🔢 Factoring can simplify the process of finding a common denominator, especially when denominators can be factored.
• 📌 Multiplying the numerator and denominator of a fraction by the same non-zero expression does not change its value.
• 📝 When subtracting fractions, ensure that the entire numerator of the fraction being subtracted is accounted for.
• 🔑 Solutions to rational equations must not result in division by zero, so check the solutions against the excluded values.
• 📉 In cases where the solutions do not satisfy the original equation, it indicates there is no solution.

Q & A

What is a rational equation?

A rational equation is an equation that contains rational expressions, which are fractions with variables in the numerator and/or denominator.

Why should we avoid dividing by zero when solving rational equations?

Division by zero is undefined in mathematics. If the denominator of a rational expression is zero, the expression itself becomes undefined, which means we cannot have a solution that results in division by zero.

How does the value of zero affect the numerator and denominator of a fraction?

If the numerator of a fraction is zero, the entire expression evaluates to zero, regardless of the denominator (as long as it's not zero). However, if the denominator is zero, the expression is undefined.

What is the purpose of finding a common denominator when solving rational equations?

Finding a common denominator allows us to combine all the fractions in the equation into a single fraction, which simplifies the process of solving the equation by making it easier to isolate the variable.

What technique does the video suggest for solving rational equations, and why?

The video suggests getting all the fractions together on one side with a common denominator. This approach is used because it is also applicable to solving rational inequalities and graphing rational functions, making it a versatile technique.

What are the excluded values for the potential solution in the first example equation x - 4 / (2ar * 3x + 1) over (2x to the 3 power * 5x - 4) equals 0?

The excluded values are x = 0 and x = 4/5 because these values would make the denominator equal to zero, which is not allowed.

How do we solve the equation 5/x + 1 = 3x/(x + 1)?

We get all the fractions on one side by subtracting 3x/(x + 1) from both sides, combine the fractions since they have a common denominator, and then solve for x by setting the numerator equal to zero and ensuring the denominator is not zero.

What is the solution to the equation 6/x = -2/(x - 4)?

The solution is x = 3, as it is the value that makes the numerator zero without making the denominator zero.

How does the video approach solving rational inequalities?

The video mentions that the same technique used for solving rational equations will be applied to rational inequalities, although it does not go into detail on how this is done.

What is a shortcut mentioned in the video for finding a common denominator?

A shortcut mentioned is to factor the denominators that can be factored, which can simplify the process of finding a common denominator.

What is the solution to the equation 6/x = 11/(3x + 8)?

The solution is x = 7/24, after finding a common denominator and solving for x by setting the numerator equal to zero.

Outlines

00:00

📚 Introduction to Solving Rational Equations

This paragraph introduces the concept of solving rational equations, which are equations containing fractions with variables, known as rational expressions. The approach discussed involves moving all fractions to one side of the equation with a common denominator. The instructor emphasizes not to multiply both sides by the least common denominator to cancel out the denominators, a method that might be quicker but not the focus of this video. The importance of understanding that division by zero is undefined and zero divided by any non-zero number is zero is highlighted, as these are critical concepts in solving rational equations. The first example provided demonstrates how to solve a basic rational equation by setting the numerator to zero while ensuring the denominator is not zero.

05:02

🧩 Simplifying Rational Equations with Common Denominators

The second paragraph delves into solving more complex rational equations by combining fractions with common denominators. The process involves moving all terms to one side of the equation and then combining them into a single fraction. The example given shows how to subtract fractions with the same denominator and solve for the variable by setting the numerator to zero. The importance of excluding values that would result in a zero denominator is reiterated, as these values are not valid solutions. The paragraph also covers how to handle equations with different denominators by multiplying the numerator and denominator by appropriate factors to achieve a common denominator.

10:03

🔍 Advanced Techniques for Rational Equations with Multiple Expressions

This paragraph presents a more advanced example involving three rational expressions. The solution process includes finding a common denominator by factoring the denominators where possible, which simplifies the task. The instructor demonstrates how to multiply each term's numerator and denominator by the necessary factors to match the common denominator. After combining all terms over the common denominator, the numerator is simplified, and the equation is solved by setting the numerator equal to zero and solving for the variable. The paragraph also discusses how to handle excluded values that would result in division by zero.

15:04

📘 Special Cases in Solving Rational Equations

The final paragraph addresses special cases in solving rational equations, such as when there are no additions or subtractions in the denominators, making the process of finding a common denominator straightforward. It also covers how to handle whole numbers by converting them into fractions and then adjusting the equation to achieve a common denominator. The paragraph concludes with an example that demonstrates these concepts, resulting in a solution for the variable. The instructor advises students to write out all steps until they are comfortable with the process before attempting to simplify steps, ensuring a solid understanding of the material.

Keywords

💡Rational Equations

Rational equations are equations that contain rational expressions, which are essentially fractions with variables in the numerator and/or denominator. The video's theme revolves around solving these equations by using a common denominator to combine all fractions on one side, which is a technique that aligns with solving rational inequalities and graphing rational functions. An example from the script is the equation 'x - 4 over 2 * (3x + 1) / (2x) to the 3 power * (5x - 4) equal to 0', which demonstrates the basic setup for solving rational equations.

💡Common Denominator

A common denominator is a single denominator that can be used for all fractions within an equation, making it possible to combine them into a single fraction. This concept is crucial in the video as it is the method used to simplify rational equations before solving them. The script illustrates this by showing how to find a common denominator for fractions with different denominators, such as multiplying the numerator and denominator by a form of one to match denominators, as seen in the equation '6 / x = -2 / (x - 4)'.

💡Undefined

In mathematics, an expression is considered undefined when it involves division by zero. The video emphasizes that a rational expression becomes undefined if its denominator is zero. This is a critical concept because it sets constraints on the potential solutions to rational equations. For example, the script mentions that 'if you got anything divided by zero then this is undefined', which is a fundamental rule when dealing with fractions and rational expressions.

💡Numerator

The numerator is the top part of a fraction and can be a polynomial or any algebraic expression. In the context of the video, setting the numerator equal to zero is a method to find the solutions to a rational equation, as long as the denominator is not zero. The script uses the term when explaining how to solve the equation 'x - 4 could be 0 or 3x + 1 could be zero', which is part of the process to find the values of x that satisfy the equation.

💡Denominator

The denominator is the bottom part of a fraction and, similar to the numerator, can include variables and polynomials. A key point made in the video is that the denominator must never be zero, as this would make the expression undefined. The script illustrates this by cautioning against values of x that would make '5x - 4' or 'x' equal to zero, as these would result in a zero denominator.

💡Factored

A factored expression is one that has been broken down into its factors, which are the simplest form of the expression's components. In the video, having a factored expression simplifies the process of identifying what makes an equation equal to zero. The script mentions that 'this side everything's factored for us', which makes it easier to find the values of x that satisfy the equation 'x - 4' or '3x + 1'.

💡Excluded Values

Excluded values are the values that cannot be solutions to an equation because they would result in an undefined expression or violate the conditions of the problem. In the context of rational equations, these are often values that make the denominator zero. The video script refers to excluded values when it states 'X can't be zero and 5x - 4 can't be zero', which are values that must be excluded from potential solutions.

💡Rational Inequalities

Rational inequalities involve comparing rational expressions to other rational expressions or numbers, similar to rational equations but with inequality signs. The video mentions that the technique used to solve rational equations will also be applied to rational inequalities, indicating a broader application of the concepts being taught. The script states, 'we're going to use the same technique to solve rational inequalities', highlighting the relevance of the method beyond just equations.

💡Rational Functions

Rational functions are a type of algebraic function that can be expressed as the ratio of two polynomials. The video script mentions that the techniques used to solve rational equations will also be useful when graphing rational functions, suggesting that understanding rational equations is foundational to more advanced topics in algebra. The script refers to rational functions when it says, 'and we're going to use similar techniques when we graph rational functions'.

💡Least Common Denominator (LCD)

The least common denominator, or LCD, is the smallest multiple that all the denominators of fractions in an equation share. While the video chooses not to use the LCD method for solving rational equations, it does mention this alternative approach. The script notes, 'you multiply both sides by the least common denominator to cancel all the denominators', acknowledging the existence of this method but opting for a different technique in the lesson.

Highlights

Introduction to solving rational equations with a focus on using common denominators.

Explanation of rational expressions as fractions with variables.

Approach to solving rational equations without multiplying by the least common denominator.

Importance of avoiding division by zero in rational expressions.

Zero numerator results in an expression evaluating to zero, unless the denominator is zero.

Guide on finding a common denominator for fractions.

First example solving a basic rational equation with zero on one side.

Exclusion of values that make the denominator zero from potential solutions.

Identification of valid solutions by setting the numerator to zero.

Second example involving combining fractions with the same denominator.

Third example with different denominators requiring adjustment for a common denominator.

Technique of multiplying by one to adjust fractions for a common denominator.

Fourth example with rational expressions and an inequality.

Method for solving rational equations with three rational expressions.

Factoring denominators to find a common denominator.

Handling of an equation with no addition or subtraction in the denominators.

Conversion of whole numbers into fractions to facilitate common denominators.

Transcripts

today we're going to look at solving

rational equations meaning equations

with rational

expressions rational expressions are

just fractions but they have variables

in them they have polinomial and the

approach we're going to take here is

getting all the fractions together on

one side with a common denominator and

going from there the reason we're going

to do that is because we're going to use

the same technique to solve rational

inequalities

and we're going to use similar

techniques when we graph rational

functions so even though there might be

a faster way to solve rational equations

and I'll mention it uh you multiply both

sides by the least common denominator to

cancel all the denominators that's not

how I'll be approaching it in this video

so let's go ahead and get

started so again a rational equation has

fractions and the in mathematics means

fraction because it has the word

ratio a couple things you need to know

about fractions is if you

got anything divided by

zero then this is undefined you cannot

divide by zero there's no number that

you get if you try to do this but if you

divide Zero by something as long as it's

something not equal to zero well that's

always zero so if your numerator is zero

the the expression evaluates to

zero if your denominator is zero the

expression is undefined that's going to

be key as we go through solving these

equations so the other thing you need to

know is how to get a common denominator

so if you're not as familiar with that

you might want to slow down there in

that part of the video in the examples

and and see if you can catch on so let's

do our first example let's try to solve

this equation

how about x -

4^

2ar * 3x +

1 over

2x to the 3 power * 5x - 4 equal

0 now our first example here is going to

be pretty basic and just hit home at the

concepts we were just talking about the

idea here here is the right side's

already zero that's good for us and the

left side all we have to do to solve

this equation is figure out what makes

the numerator equals

zero and doesn't make the denominator

equal zero so I'm going to make a note

here in this step I don't want my

denominator to be zero and the two

values that would do that is if x were

equal to

zero or if five so we don't want X to be

zero and we don't want 5x - 4 to be zero

you can check that if either one of

those are zero and you multiply this

denominator out you'll get

zero so X can't be zero and 5x - 4 can't

be zero that means that X can't be if

you add four and divide by five x also

can't be four fths so these are these

are values we want to exclude from our

potential solution we don't want those

however if we want to figure out what

makes the expression equal to zero we

look at the numerator right we want the

numerator to be zero well in that case x

- 4 could be 0er or 3x + 1 could be

zero now the reason this is so easy is

because we have zero on one side and

this side everything's factored for us

that's super

nice having it factored makes it simple

to identify what makes it equal zero so

X could be 4 or X could be

1/3 and those are both valid Solutions

because they're not either one of our

excluded values from above so that's a

pretty basic one let's try something a

little more

involved let's try to solve 5/x + 1

equal uh how about 3x overx +

1 so this is set up a bit differently

than the last one what we want to do is

get all the fractions together on one

side so um we could subtract the 3x

overx plus one fraction over or we could

subtract the 5 over X+ one fraction over

it doesn't really matter um let's

subtract the 3x over X+1

fraction so 5 overx + 1 minus 3x overx +

1 equal 0er we like the zero over here

because that means all of our terms are

together on the left side we just have

to combine these now these two fractions

the good news is they have the same

denominator so all we have to do is put

them together as one fraction over that

common denominator and subtract the two

numerators 5 -

3x and now this is set up like our last

example a fraction equals z so we can

see that our denominator we don't want

that to be zero so X can't

be1 our numerator equals 0o will give us

our solution so let's subtract five and

divide by

-3 and negative divided negative is

positive and 5/3 is our solution because

it is not our excluded

value so that not not too bad right they

had the same denominator so that made it

pretty simple let's try

another let's try something like uh how

about 6

overx = uh -2 over x -

4 so again we want all of our fractions

on the same side so I'm going to add the

fraction from the right over to the left

to give us 6X + 2x - 4 =

Z and now the two fractions on the left

side do not have the same denominator so

this is what we're going to do I'm going

to take my 6 overx fraction and I'm

going to multiply the top and the Bottom

by X+ 4 or Sorry xus 4 you'll see why

shortly one thing I want to note is by

multiplying by x - 4 over x - 4 that's

the same thing as multiplying by one

right cuz x - 4 x - 4 is just 1 and we

know that multiplying by one doesn't

change the value of what we have so this

is perfectly fine to do you're allowed

to multiply by

one then we take our 2x - 4 fraction and

we want the denominators to match well

so what I'm going to do is multiply the

top and the bottom of this fraction by

X and we can see that the denominators

now are x * x - 4 in both cases

so now what we get is we get we're going

to put these together over one

denominator x * x - 4 and on top I'll go

ahead and combine like terms so I get 6x

-

24 +

2x that equals zero and then let's just

uh combine like terms on top we get 8x -

24 over x * x - 4

equal

0 well we can see from our denominator

that X can't be 0 or

4 and in our

numerator if we want our numerator to be

zero then add 24 and divide by 8 we can

see that X is three so three is the

solution because it's not either of our

excluded

values so again the key to getting a

common denominator is multiplying top

and the Bottom by something so that the

denominators in all the fractions match

and that that can take some

practice okay let's try another one how

about 5 overx - 2 is greater than sorry

is equal to 3 over x -

3 so let's get our fractions on the same

side by

subtracting 3 overx - 3

then we need a common denominator so I'm

going to take a little bit of a shortcut

here I'm going to go ahead and multiply

the first fraction on top and bottom by

xus

3 I'm just going to put it in this step

but I'll use a different

color and over here I'm going multiply

top and bottom by xus

2 and so now our denominators match so

we can put both fractions

together into to a single fraction with

that common

denominator and on top I'm going to get

5x -

15 and this negative here distributes

with the three because I'm subtracting

this entire numerator so I get -3x + 2

so 5x -3x is

2x minus the 15 + the 6 is -

99 and our excluded values are 3 and two

and our numerator is zero if x =

9es okay so hopefully you're getting the

idea we can start skipping some some of

those smaller steps but I always

encourage students write as many steps

as it

needs a whole lot until you really get

the idea down before you start to skip

steps

okay now let's try one that's got three

rational

expressions X over x -

4 + 6 over x -

9 = 7 x - 48 over x^2 -

13x +

36 now we know we're going to try to put

all these fractions together with a

common denominator and one thing we

haven't seen yet is when trying to find

a common denominator it can be handy if

you factor the denominators that can be

factored so x^2 - 13x + 36 you could use

the AC method or whatever method you

like but it factors as x - 9 * x -

4 that's going to be handy uh when we

try to find a common denominator so I'm

going to subtract this fraction over x

overx - 4 and I can already see that

this will be my common denominator so

what I'm going to do is go ahead and

write

in on this fraction I'm multiply top and

bottom by x -

9 you know write more steps if you need

to but um hopefully this makes sense my

next fraction 6 overx - 9 I'll have to

multiply top and bottom by x -4

and then I'm going to subtract this

entire fraction over when I when I do

that I could put minus this whole

fraction actually let's go ah and do

that

minus 7 x - 48 over x - 9 * x

-4 equals 0 and again I just want to

remind you when you got a minus in front

of a fraction that minus applies to the

entire numerator

Okay the reason I say that is because in

the next step we're going to put all

these numerators together over the

common denominator x - 9 * x -

4 and so I get X squar that's the only X

squ term I'm going to have on top so

I'll write that down then I get -

9x and here I get plus 6X so that's -

3x -

7x is - 10 x okay

so we had - 99x + 6 x is -3 - 7 is -10

now we've used all the terms here we get

a -4 out of this guy and we get a

positive 48 out of that guy so we get

plus

24 all right so now our we've put

everything together our excluded values

are X can't be 9 or

4 and if we want to if we want the

numerator to be Z Z we we need to factor

this or use the quadratic formula well

luckily it factors very nicely as x - 6

* x -

4 and we can see we get two solutions x

- 6 could be zero meaning x = 6 or x - 4

could be 0 which means x =

4 but we already said X can't be four so

we throw that guy out and we only get

xal 6 if you plug 6 in for x to the

original equation you should get a true

statement and you can see if you try to

plug four in well it would cause you to

try to divide by zero which you can't

even

do there are cases where none of your

Solutions work and when that happens you

just say no

solution let's do one last example

solve the

equation 6 /x =

11 over

3x +

8 and this one's a little more a

little different than the others there's

no adding or subtracting in the

denominators that actually makes this

one a little bit easier but since it

doesn't look the same as the others it

might might throw you off a little bit

what I'm going to do this time is I'm

going to subtract the 6 overx to the

right because I already have two of my

terms over

here so I've got 11 over

3x and I've got this eight what I like

to do with an eight is make it into a

fraction so I'll call it plus 8 over 1

and then finally - 6

overx and I want the all three

denominators here to match so 3x is what

I can turn all of them into for example

on this fraction I can multiply the top

and the Bottom by a

three on this fraction I can multiply

top and bottom by

3x because 1 * 3x is

3x so we get

11 +

24x minus 18 I could have probably

combined the like terms there before and

that's all over the common denominator

of

3x and I can see at this step um my

excluded value is zero I don't want X to

be zero because I would make my

denominator zero and then on

top to make the fraction equals z to

solve the equation I'd have

24x 11 - 18 is

-7 and then I can add 7 and divide by

24 to get X by

itself and the solution we get is X =

724 so a couple new things here um when

you don't have addition or subtraction

in your denominators it's just

multiplication so no problem in building

a common denominator and when you have

an integer or a whole number turn it

into a fraction put it over one and then

you can multiply top and bottom by

whatever you need to get the same

denominator