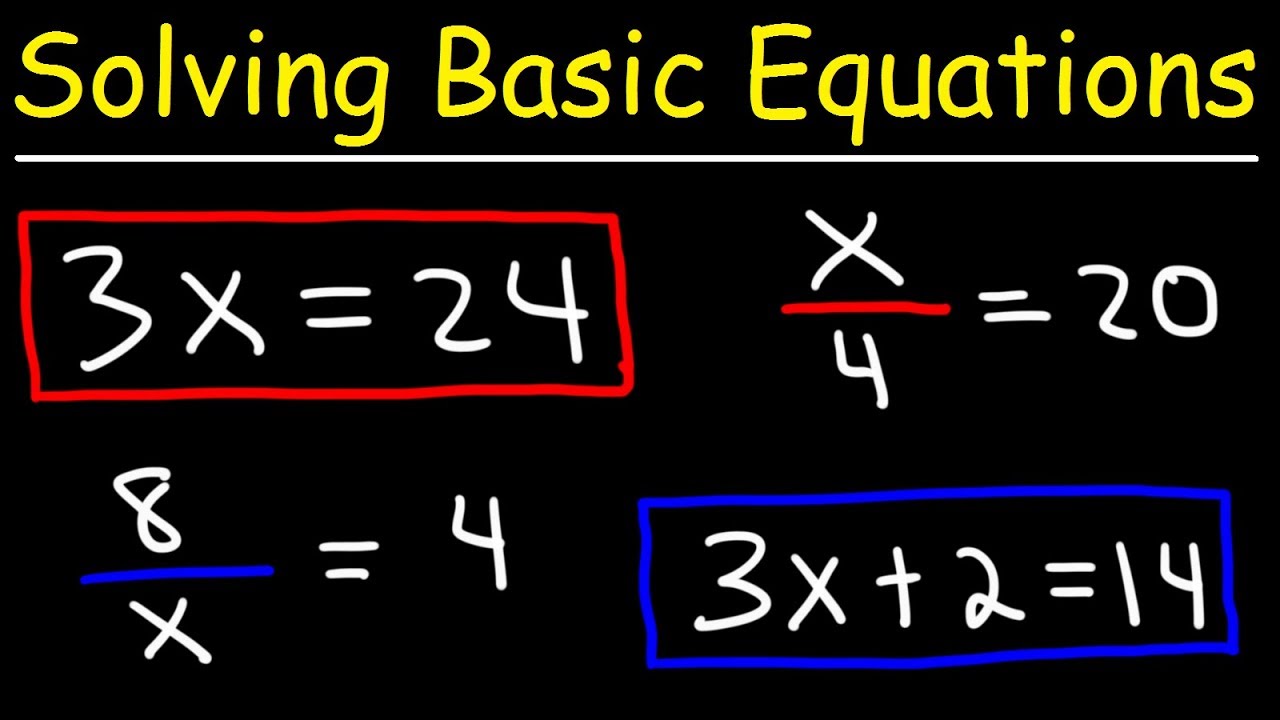Algebra - How To Solve Equations Quickly!
Summary
TLDRThis video tutorial offers a comprehensive guide to solving two-step equations, covering a range of scenarios including those with fractions, decimals, and parentheses. The instructor demonstrates techniques such as isolating variables, using opposite operations, and applying the distributive property. Examples with multiple variables and fractions are also addressed, with a focus on simplifying equations and finding the value of 'x'. The video concludes with a recommendation for an algebra course on Udemy, suggesting further resources for those seeking to deepen their understanding of algebraic concepts.
Takeaways
- 🔢 To solve two-step equations, the first step is often to isolate the variable by performing operations such as subtraction or division to eliminate constants or coefficients.
- ➖ When dealing with equations like 3x + 5 = 17, subtracting 5 from both sides helps to isolate the variable term.
- 🔄 The opposite operation principle is key: if a number is added to a variable, subtract it; if a variable is multiplied, divide by the coefficient.
- 📉 Subtraction is used to eliminate constants on one side of the equation to simplify the process of isolating the variable.
- ➗ Division is necessary to separate the variable from its coefficient, especially after simplifying the equation.
- 🔄 When an equation includes fractions, multiplying every term by the least common multiple (LCM) of the denominators can eliminate the fractions.
- 🔄 Parentheses in equations can be handled by using the distributive property to simplify and solve the equation.
- 📉 The process of moving all variable terms to one side and constants to the other is crucial for solving equations with variables on both sides.
- 🔢 Dealing with decimals in equations can be simplified by multiplying all terms by a power of 10 to eliminate the decimal places.
- 📚 The script suggests using algebra courses, like those on Udemy, for further practice and understanding of algebraic concepts, including operations, equations, and functions.
Q & A
What is the first step in solving a basic two-step equation like 3x + 5 = 17?
-The first step is to isolate the variable x by performing the opposite operation of what is done to it. In this case, since 5 is added to 3x, you subtract 5 from both sides of the equation.
How do you solve the equation 4x + 3 = 19?
-You start by subtracting 3 from both sides to eliminate the constant term on the left side. Then, you divide both sides by 4 to isolate x, resulting in x = 4.
What is the strategy for solving an equation with a variable on both sides, like 17 - 5x = 2?
-The strategy is to get all the variable terms on one side and all the constant terms on the other side. For this equation, you subtract 17 from both sides and then divide by -5 to solve for x.
How do you handle a fraction in an equation like 9 = 3 + x/4?
-You eliminate the fraction by multiplying every term by the denominator's opposite, which in this case is 4. This transforms x/4 into x and allows you to solve for x as if it were a simple equation.
What if you have an equation with multiple x terms, such as 3x + 8 + 5x = 32?
-You first combine like terms, adding 3x and 5x to get 8x. Then you proceed with the usual steps of isolating the variable by performing operations on both sides of the equation.
How do you solve an equation with parentheses, like 3 * (2x - 4) + 1 = 7?
-You use the distributive property to eliminate the parentheses by multiplying the term outside the parentheses by each term inside. Then you combine like terms and solve for x.
What is the approach to solving equations with variables on both sides, such as 13 - 2x = 4x?
-You move all x terms to one side by adding or subtracting them from both sides and all constant terms to the other side. Then you solve for x by isolating it on one side.
How do you handle equations with decimals, like 2x + 0.3 = 1.5?
-You can either solve it directly or eliminate decimals by multiplying every term by a power of 10 that moves all decimal points to the right of the number, effectively turning them into whole numbers.
What if an equation has multiple fractions, like 2x/3 + 4/2 = 3x/2 + 1?
-You find a common denominator to eliminate the fractions, or you can multiply every term by the least common multiple of the denominators to clear the fractions from the equation.
How do you approach an equation with parentheses on both sides, like 2 + 4 * 3x + 2 = 3 - 4 * 5x + 1 + 14?
-You apply the distributive property to eliminate the parentheses and then combine like terms. After that, you isolate the variable x by moving all x terms to one side and constants to the other, and then solve for x.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
5.0 / 5 (0 votes)