Statistika - Ukuran Pemusatan Data (Mean, Median, Modus)
Summary
TLDRThis tutorial offers a comprehensive introduction to statistical measures of central tendency, focusing on the mean (average), median, and mode. The video explains how to calculate these values for both individual and grouped data using clear examples. It also covers the necessary formulas and provides step-by-step guidance on how to calculate the mean, median, and mode, including handling frequency distributions and cumulative frequencies. Aimed at students learning basic statistics, this video simplifies the concepts for easy understanding and application in real-life data analysis.
Takeaways
- 😀 The video explains three main measures of central tendency in statistics: mean, median, and mode.
- 😀 The mean is calculated by summing all the values and dividing by the number of data points.
- 😀 Median represents the middle value of a dataset when arranged in ascending or descending order, with different formulas for odd and even datasets.
- 😀 Mode refers to the most frequently occurring value in a dataset.
- 😀 For single data, the mean can be calculated using a simple formula where the sum of all values is divided by the total number of values.
- 😀 When the data is grouped into frequency distribution tables, the mean requires finding the midpoint of each class and using the formula involving the frequencies.
- 😀 The median formula differs between odd and even datasets: for odd, it's the middle value, and for even, it's the average of the two middle values.
- 😀 For grouped data, the cumulative frequency and class intervals are essential in calculating the median.
- 😀 The mode for single data is simply the value with the highest frequency, while for grouped data, it involves a more complex formula with the class boundaries.
- 😀 The video emphasizes the importance of understanding both single data and grouped data calculations for these measures, providing clear examples and step-by-step guides.
- 😀 It concludes by encouraging viewers to ask questions and interact with the content, while also reminding them of the importance of accuracy and effort in learning statistics.
Q & A
What are the three main measures of central tendency discussed in the video?
-The three main measures of central tendency discussed in the video are mean (average), median, and mode.
How is the mean (average) calculated for individual data?
-For individual data, the mean is calculated by summing all data values and then dividing the total by the number of data points.
What is the difference between the mean calculation for individual data and grouped data?
-For individual data, the mean is calculated directly by summing the values. For grouped data, the mean is calculated using the frequency and midpoint (class mark) of each group, with the sum of the frequencies multiplied by the midpoints.
What is the formula for calculating the median in individual data when the number of data points is odd?
-For odd-numbered data, the median is calculated as the value at the position (n+1)/2, where n is the number of data points.
How do you calculate the median for even-numbered data?
-For even-numbered data, the median is the average of the values at positions n/2 and (n/2)+1, where n is the number of data points.
What is the purpose of cumulative frequency in median calculation?
-Cumulative frequency helps identify the class or data point that contains the median by summing the frequencies progressively, making it easier to locate the middle value in a set of data.
How is the mode determined in individual data?
-In individual data, the mode is the value that appears most frequently. If multiple values share the highest frequency, they are all considered modes.
What is the formula for finding the mode in grouped data?
-In grouped data, the mode is calculated using the formula: Mode = Lower boundary of the modal class + (D1 / (D1 + D2)) * class width, where D1 is the difference between the frequency of the modal class and the previous class, and D2 is the difference between the frequency of the modal class and the next class.
How is the class midpoint (titik tengah) calculated for grouped data?
-The class midpoint is calculated by averaging the lower and upper boundaries of each class interval.
What is the significance of class width in calculating the mode for grouped data?
-The class width is important for calculating the mode because it is used in the formula to adjust for the frequency differences between the modal class and its neighboring classes.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Statistika 1 ~ Ukuran Pemusatan Data (Mean/Rata-Rata, Median, Modus) ~ Materi PJJ Kelas VIII / 8 SMP
Mean, Median and Mode in Statistics | Statistics Tutorial | MarinStatsLectures

Statistics: The average | Descriptive statistics | Probability and Statistics | Khan Academy
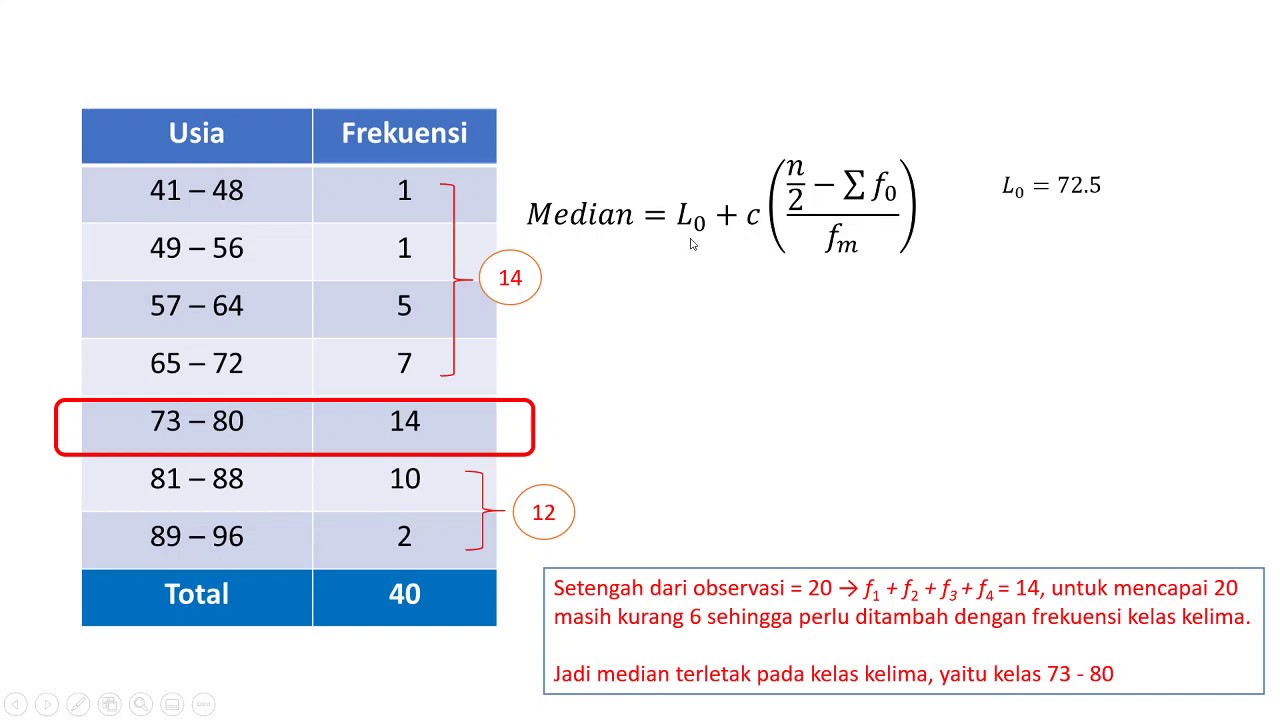
UKURAN PEMUSATAN DATA BERKELOMPOK | Rataan Median Modus Kuartil Desil Persentil

Statistika Dasar Ukuran Pemusatan Data (Mean, Modus, Median) Data Tunggal dan Data Kelompok

Tendensi Sentral: Mean, Median, Modus
5.0 / 5 (0 votes)