PENYELESAIAN PDP DENGAN PEMISAHAN VARIABEL BAG 3
Summary
TLDRThis video explores the method of separation of variables for solving partial differential equations (PDEs), with a focus on Fourier series applied to a vibrating string. The instructor discusses using superposition principles to derive solutions, the application of Fourier series to satisfy boundary and initial conditions, and the calculation of Fourier coefficients. Emphasis is placed on solving wave equations, especially when the string is fixed at both ends, with future lessons covering example problems. The lecture is designed for students of physics and mathematics, helping them understand complex wave phenomena through Fourier analysis.
Takeaways
- 😀 The script discusses solving partial differential equations (PDEs) using the method of separation of variables, specifically in the context of vibrating strings.
- 😀 The process involves applying Fourier series to solve the wave equation, taking into account boundary conditions where both ends of the string are fixed.
- 😀 The principle of superposition is highlighted, indicating that the sum of multiple solutions to the PDE will also satisfy the equation.
- 😀 The Fourier series solution is presented as a sum of terms involving sinusoidal functions (sine and cosine), which can represent the motion of the vibrating string.
- 😀 The boundary conditions lead to simplifications, where terms that involve cosine and sine functions are eliminated when evaluated at the ends of the string.
- 😀 The Fourier coefficients are derived through integration of the function at the given boundaries, with formulas provided for specific cases.
- 😀 The solution involves considering both the displacement (UX) and velocity (UT) of the string at any point along its length and time.
- 😀 In cases where the initial velocity is zero, the solution simplifies to a form where only the displacement function is considered.
- 😀 The script provides insight into how to handle different initial conditions, including when there is no initial velocity (GX = 0).
- 😀 The importance of carefully selecting the right equation based on initial conditions (whether velocity is zero or not) is emphasized for proper application of Fourier series in solving the wave equation.
- 😀 The video concludes by mentioning that future content will include worked examples for better understanding of the method applied to real-world problems involving vibrating strings.
Please replace the link and try again.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

PENYELESAIAN PDP DENGAN PEMISAHAN VARIABEL BAG 2
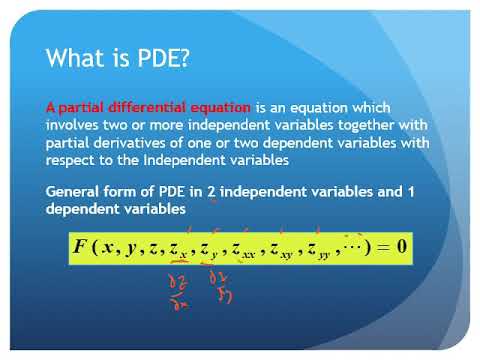
Persamaan Diferensial Parsial (Pengertian, Definisi, dan Klasifikasi)

FOURIER SERIES LECTURE 1 | STUDY OF DEFINITION AND ALL BASIC POINTS @TIKLESACADEMY
Transform Calculus and its applications in Differential Equations

Oxford Calculus: Separable Solutions to PDEs

A concept of Differential Equation
5.0 / 5 (0 votes)