KALKULUS | APLIKASI INTEGRAL | LUAS DAERAH
Summary
TLDRThis video explores the application of integrals to calculate areas and volumes, focusing primarily on finding the area of regions bounded by curves. It explains the process of partitioning a region into small slices and approximating the area using integral calculus. The video includes several examples, such as calculating the area under a parabola, between two curves, and within specific boundaries, with detailed step-by-step solutions. Key concepts like vertical and horizontal slicing methods are also discussed, making the video an insightful resource for understanding how integrals are used to solve real-world geometric problems.
Takeaways
- 😀 The video discusses two main applications of integrals: calculating areas of regions and the volumes of solids of revolution.
- 😀 To calculate the area of a region bounded by a curve and the x-axis, the area can be approximated by partitioning the region into small vertical slices (using Δx), where the area of each slice is approximated by the area of a rectangle.
- 😀 As the number of partitions (n) approaches infinity, the area can be found using the definite integral of the function with respect to x from the lower to the upper limits of the region.
- 😀 When the area is bounded by two curves, the area between them can be calculated by subtracting the lower function from the upper function, and then integrating over the specified interval.
- 😀 For the first example, the area bounded by the parabola y = x^2 and the line x = 2 was calculated using the integral of x^2 from 0 to 2, yielding an area of 8/3 square units.
- 😀 In the second example, the area between the line y = x + 4 and the parabola y = x^2 - 2 was calculated using definite integrals, and the result was 125/60 square units.
- 😀 The third example discusses calculating the area between the line y = -x + 2 and the parabola y = x^2, with a segmented approach, considering two parts of the region: from x = 0 to 1, and from x = 1 to 2.
- 😀 The fourth example involves calculating the area in the first quadrant between the parabola y = x^2 and the line y = 4, with an area of 16/3 square units using vertical slices and definite integrals.
- 😀 In cases where partitioning the region horizontally (with respect to y) is more convenient, the integral must be expressed in terms of y, such as for the area bounded by y = x^2 and y = 4, where the function was transformed to x = √y.
- 😀 The last example involved finding the area between the parabola x = 3 - y^2 and the line x = y + 1, requiring integration with respect to y. The result was 9/2 square units.
Q & A
What is the first application of integrals discussed in the script?
-The first application discussed is calculating the area of a region bounded by a curve, which is the 'luas daerah' or area calculation.
How is the area of a region under a curve approximated in the script?
-The area is approximated by partitioning the region into small sections (n parts) and calculating the area of each section as a rectangle. As the number of sections increases towards infinity, the sum of these areas approaches the exact value, which is represented by the integral.
What does the integral represent in the context of finding the area of a region?
-The integral represents the limit of the sum of areas of infinitely many small rectangles, which gives the exact area under the curve between two specified limits (a and b).
What is the formula for the area of a region between two curves, as discussed in the video?
-The area between two curves is calculated as the integral of the difference between the two functions (f(x) - g(x)) over the interval [a, b].
What function is used to calculate the area under the curve y = x² between x = 0 and x = 2?
-The function used is y = x², and the area is calculated by integrating x² from 0 to 2, resulting in the formula 1/3 x³ with limits 0 and 2, giving an area of 8/3 square units.
How do you find the intersection points of the parabola y = x² and the line y = x + 4?
-To find the intersection points, you set the two equations equal to each other, i.e., x² = x + 4. This leads to solving the quadratic equation x² - x - 4 = 0, which gives the intersection points x = -2 and x = 3.
What is the method used to calculate the area between the curve y = x² - 2 and the line y = x + 4?
-The area is found by integrating the difference between the two functions, x + 4 and x² - 2, from the x-values where they intersect (-2 to 3).
In the case where the region is bounded by a curve and the x-axis, how is the area computed?
-The area is computed by integrating the function representing the curve over the interval where it intersects the x-axis, which gives the total enclosed area.
What happens when the region is bounded by multiple curves and the x-axis, as seen in the examples involving the curve y = x² and the line y = -x + 2?
-When the region is bounded by multiple curves and the x-axis, the area is split into different parts. Each part is calculated separately, and then the areas are added together to find the total area.
What is the significance of partitioning the area either vertically or horizontally in integral calculations?
-Partitioning the area vertically or horizontally depends on the nature of the curves and the problem. In vertical partitions, the x-values are integrated, while in horizontal partitions, the y-values are integrated. Both methods can be used to calculate the area, but the choice depends on the given functions and boundaries.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
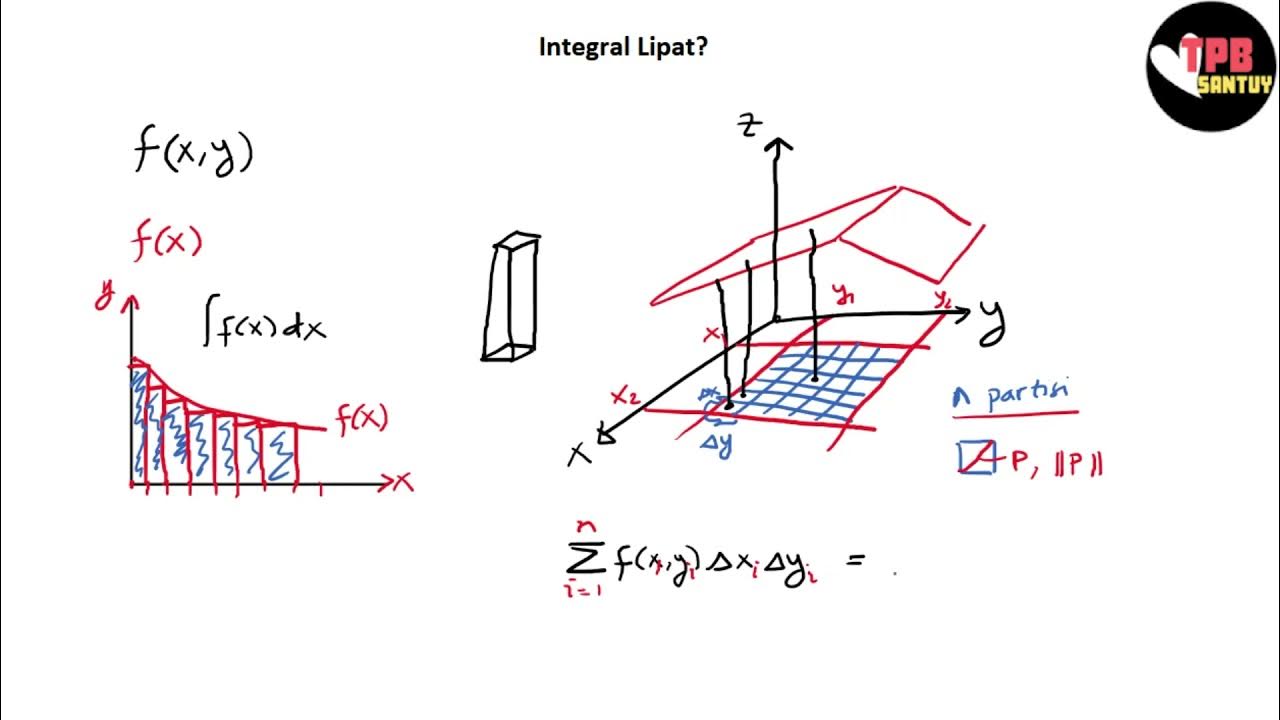
Integral Lipat | Integral Lipat (Part 1) | Kalkulus
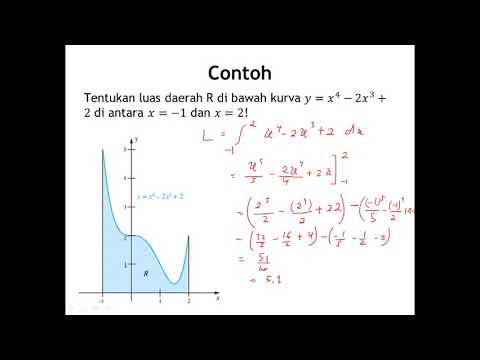
5 1 Luas Daerah Bidang Rata
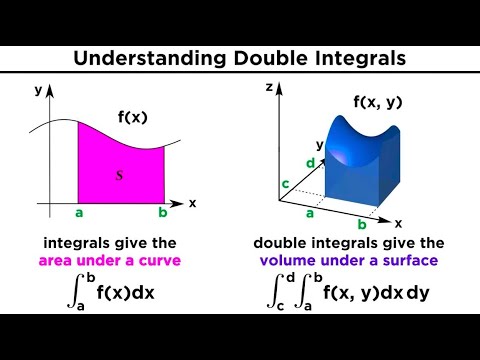
Double and Triple Integrals
Integrals: Crash Course Physics #3

M202 Kalkulus : Pengantar Integral dan aplikasinya (part a) - Definisi dan Pembuktian

Menentukan Volume Benda Putar dengan Menggunakan Integral (Integral Part 5) M4THLAB
5.0 / 5 (0 votes)