Complex Numbers : Modulus and Argument | ExamSolutions

Summary

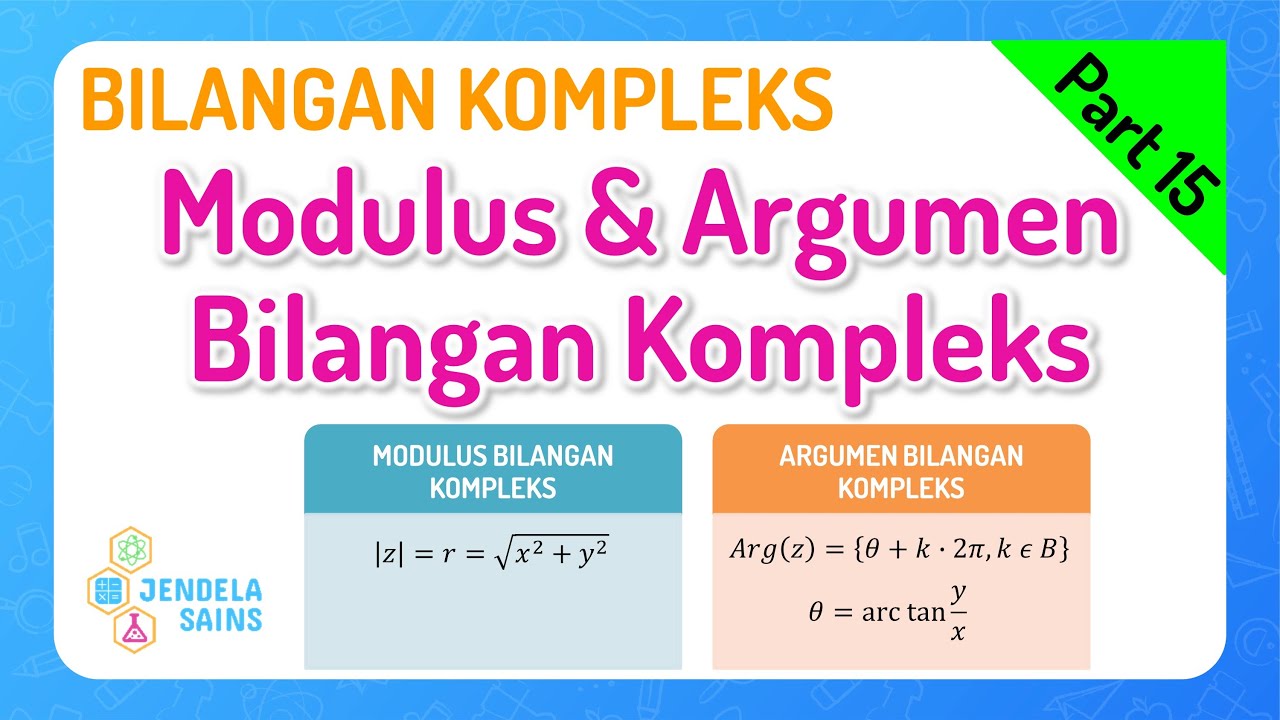
This video introduces the concepts of the modulus and argument of complex numbers. It explains how to calculate the modulus as the distance from the origin using the Pythagorean theorem, and how to find the argument as the angle between the positive real axis and the complex number. The video includes practical examples for calculating both the modulus and argument in different quadrants, emphasizing the importance of drawing sketches for accuracy. Key formulas and the careful handling of negative arguments in certain quadrants are also covered to ensure proper calculations.

The video is abnormal, and we are working hard to fix it.
Please replace the link and try again.

Q & A

What is the modulus of a complex number?

The modulus of a complex number Z, denoted as |Z|, refers to the length of the line drawn from the origin to the point representing the complex number on the Argand diagram. It is calculated using the Pythagorean theorem, i.e., the square root of the sum of the squares of the real and imaginary parts of the complex number.

How do you calculate the modulus of a complex number in the form Z = a + bi?

The modulus of a complex number Z = a + bi is calculated as the square root of (a^2 + b^2), where 'a' is the real part and 'b' is the imaginary part of the complex number.

What are some common notations used for the modulus of a complex number?

The modulus of a complex number is often written as |Z|, which is referred to as 'mod Z'. Some authors may also denote the modulus as 'R', where R = √(a² + b²).

What is the argument of a complex number?

The argument of a complex number, denoted as arg(Z), is the angle θ between the positive real axis and the line representing the complex number on the Argand diagram. This angle is measured in radians and lies between -π and π.

How is the argument of a complex number calculated?

The argument of a complex number is calculated by finding the acute angle α in the right triangle formed by the real and imaginary parts of the complex number. This angle can be determined using the arctangent function: α = arctan(|b| / |a|), and the argument θ is then adjusted based on the quadrant the complex number lies in.

Why is it important to always draw a sketch when calculating the argument of a complex number?

Drawing a sketch helps visualize the position of the complex number on the Argand diagram and aids in identifying the correct quadrant. This is crucial for correctly determining the argument, as angles vary depending on which quadrant the complex number is in.

What is the significance of using radians rather than degrees when calculating the argument of a complex number?

Radians are the standard unit of measurement for angles in most mathematical contexts, including when working with complex numbers. The argument of a complex number is typically measured in radians because the mathematical operations involving complex numbers, such as trigonometric functions, are more naturally expressed in radians.

What do you do if the complex number lies in the third or fourth quadrant?

If the complex number lies in the third or fourth quadrant, the argument θ will be negative. You can calculate the acute angle α using the arctangent function and then adjust the argument by subtracting α from π for the third quadrant, or adding a negative value to α for the fourth quadrant.

How can you avoid mistakes when calculating the argument of a complex number?

To avoid mistakes, always draw a diagram, clearly mark the real and imaginary parts, and determine the acute angle first. Afterward, adjust the angle based on the quadrant the complex number is located in. It is also important to correctly interpret the signs of the real and imaginary parts.

What is the difference in approach when calculating the modulus and argument for complex numbers in different quadrants?

For the modulus, the calculation remains the same regardless of the quadrant, as it depends only on the magnitudes of the real and imaginary parts. However, for the argument, the approach differs based on the quadrant: in the first quadrant, the angle is positive; in the second, the angle is also positive but adjusted by subtracting from π; and in the third and fourth quadrants, the angle is negative and adjusted accordingly.