المعنى الحقيقي للظل، و ظل التمام (الجزء الأول) Real meaning of tan and cotan ( Part 1)
Summary
TLDRThe video script explains the mathematical concepts of tangent (tan) and cotangent (cot) using real-life examples, particularly focusing on how light creates shadows. It describes the relationship between these functions and angles, emphasizing their relevance in understanding trigonometry. The speaker illustrates how the length of a shadow corresponds to the angle of elevation of a light source, establishing a practical context for these mathematical terms. By discussing complementary angles, the speaker enhances comprehension of how tangent and cotangent interrelate, encouraging viewers to engage further with the content.
Takeaways
- 😀 The speaker introduces the concepts of tangent (tan) and cotangent (cot) in trigonometry.
- 😀 The discussion emphasizes the importance of understanding these concepts in real-life contexts.
- 😀 The video illustrates how light behaves when it strikes an object at an angle, creating shadows.
- 😀 The tangent of an angle is described as the length of the shadow created by that angle.
- 😀 The complementary angle to any angle θ (theta) is crucial, as its sum with θ equals 90 degrees.
- 😀 If θ is 50 degrees, its complementary angle is 40 degrees, demonstrating how they add up to 90.
- 😀 The speaker defines tangent (tan) as the ratio of the opposite side to the adjacent side in a right triangle.
- 😀 Cotangent (cot) is presented as the ratio of the adjacent side to the opposite side.
- 😀 The relationship between tangent and cotangent is explained, highlighting their complementary nature.
- 😀 The video encourages viewers to engage further by subscribing and sharing the content for continued learning.
Q & A
What is the definition of tangent in trigonometry?
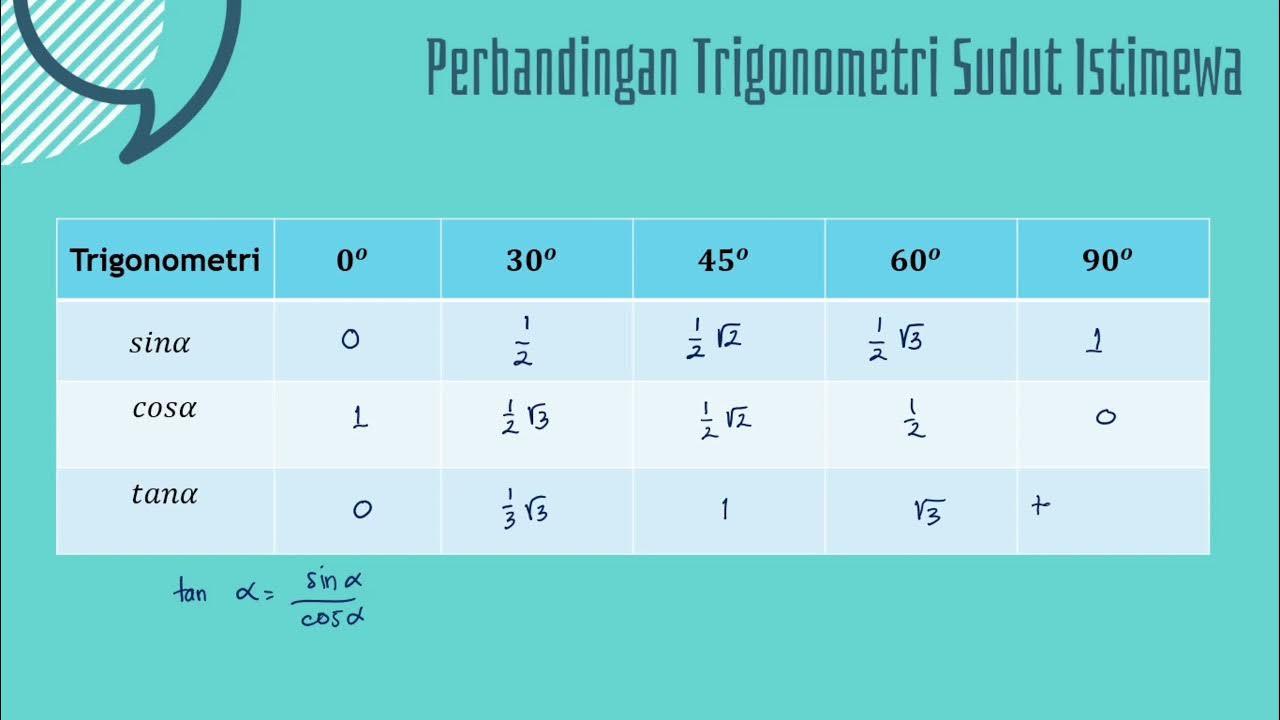
-Tangent (tan) of an angle (θ) is defined as the ratio of the length of the opposite side to the length of the adjacent side in a right triangle.
How is cotangent defined in relation to tangent?
-Cotangent (cot) is defined as the reciprocal of tangent, expressed as cot(θ) = 1/tan(θ) = adjacent/opposite.
What does the geometric interpretation of tangent involve?
-The geometric interpretation involves visualizing a right triangle formed by a vertical pole and its shadow when light is cast on it, with tangent representing the length of the shadow relative to the height of the pole.
What are complementary angles in trigonometry?
-Complementary angles are two angles that sum up to 90 degrees (π/2 radians). For an angle θ, its complement is (90° - θ).
What is the relationship between tangent and cotangent for complementary angles?
-For complementary angles, tan(θ) = cot(90° - θ) and cot(θ) = tan(90° - θ). This means that the tangent of one angle is equal to the cotangent of its complement.
How is the tangent of an angle visually represented?
-The tangent of an angle can be visually represented as the length of the shadow created by a vertical line (like a pole) when light shines on it at a certain angle.
What role does the pole's height play in the tangent calculation?
-The height of the pole represents the opposite side of the triangle, and its length is a key factor in calculating the tangent of the angle formed with the light ray.
Can you provide an example of calculating tangent using complementary angles?
-If θ is 30 degrees, its complement is 60 degrees. We know tan(30°) = 1/√3, so cot(60°) = 1/tan(60°) = 1/(√3) = tan(30°).
What should one keep in mind regarding the angles when using tangent and cotangent?
-One should remember that tangent and cotangent are interconnected through complementary angles, and knowing one can help determine the other.
How can the concepts of tangent and cotangent be applied in real-life situations?
-These concepts can be applied in fields such as engineering, physics, and architecture, where understanding angles and distances is crucial for design and analysis.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
5.0 / 5 (0 votes)