Incenter, Circumcenter, Orthocenter & Centroid of a Triangle - Geometry

Summary

This educational video script delves into the geometric centers of a triangle, explaining the incenter found by angle bisectors, the centroid located at the medians' intersection, the orthocenter where altitudes meet, and the circumcenter at the perpendicular bisectors' convergence. It clarifies the position of these centers in acute, right, and obtuse triangles, providing a comprehensive review of their identification and properties.

Takeaways

• 📐 The incenter of a triangle is found at the intersection of the three angle bisectors and is always inside the triangle.
• 🔵 The incenter is the center of the inscribed circle of the triangle, which touches all three sides.
• 📏 The centroid, found at the intersection of the three medians, is always inside the triangle and divides each median into segments with a ratio of 2:1.
• 📍 The orthocenter is the intersection of the three altitudes and can lie inside, on, or outside the triangle depending on whether it's acute, right, or obtuse.
• 🔺 In an acute triangle, the orthocenter is inside; in a right triangle, it's at the right angle vertex; and in an obtuse triangle, it's outside.
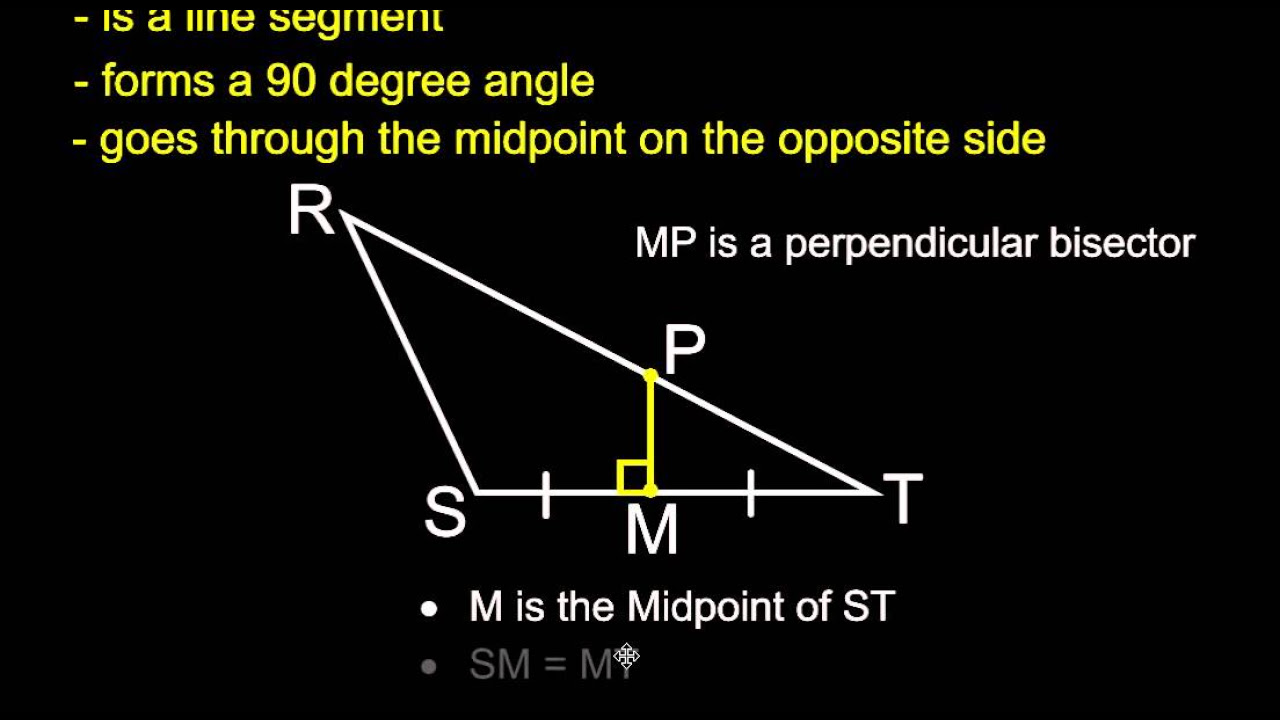
• 🌐 The circumcenter is the intersection of the three perpendicular bisectors and can also be inside, on, or outside the triangle depending on its type.
• 🔶 For acute triangles, the circumcenter is inside; for right triangles, it's at the midpoint of the hypotenuse; and for obtuse triangles, it's outside.
• 🔄 The perpendicular bisector is a line that divides a side into two equal lengths and is perpendicular to that side.
• 🔵 The incenter and circumcenter are related to the inscribed and circumscribed circles, respectively, with the incenter being the center of the inscribed circle and the circumcenter being the center of the circumscribed circle.
• 📘 The script provides a comprehensive review of identifying the incenter, centroid, orthocenter, and circumcenter of a triangle.

Q & A

What is the incenter of a triangle?

The incenter of a triangle is the point where the three angle bisectors of the triangle intersect. It is always located inside the triangle and is the center of the inscribed circle (incircle) of the triangle.

How do you find the centroid of a triangle?

The centroid of a triangle is found by the intersection of the three medians of the triangle. A median is a line segment from a vertex to the midpoint of the opposite side.

What is the relationship between the segments AP and PE in terms of the centroid?

For the centroid, the segment AP is two-thirds the length of the median AE, and PE is one-third the length of AE. In other words, AP is twice the length of PE.

Can the orthocenter of a triangle always be found inside the triangle?

No, the orthocenter of a triangle does not always lie inside the triangle. It can be inside an acute triangle, on the right triangle if it's a right triangle, or outside the triangle if it's an obtuse triangle.

How is the orthocenter of a triangle located?

The orthocenter is located at the intersection of the three altitudes of the triangle. An altitude is a perpendicular line from a vertex to the opposite side.

What is the circumcenter of a triangle?

The circumcenter of a triangle is the point where the three perpendicular bisectors of the sides of the triangle intersect. It is the center of the circumscribed circle (circumcircle) around the triangle.

Is the circumcenter always inside the triangle?

No, the circumcenter's location depends on the type of triangle. It is inside an acute triangle, on the right triangle if it's a right triangle, and outside an obtuse triangle.

What is the difference between an incenter and a circumcenter?

The incenter is the center of the inscribed circle inside the triangle, found by the intersection of the angle bisectors. The circumcenter is the center of the circumscribed circle around the triangle, found by the intersection of the perpendicular bisectors of the sides.

How does the location of the orthocenter differ in a right triangle compared to an obtuse triangle?

In a right triangle, the orthocenter is located at the right angle vertex, while in an obtuse triangle, the orthocenter lies outside the triangle.

Can you identify the circumcenter of a right triangle by its position on the hypotenuse?

Yes, the circumcenter of a right triangle is located at the midpoint of the hypotenuse.

What property do the perpendicular bisectors have in relation to the circumcenter?

The perpendicular bisectors of the sides of a triangle are the lines that are both perpendicular to a side and bisect it, thus creating two congruent segments. The circumcenter is the point where all three of these bisectors intersect.

Outlines

00:00

📐 Introduction to Triangle Centers

This paragraph introduces the concept of the incenter, centroid, orthocenter, and circumcenter of a triangle. The incenter is described as the point where the three angle bisectors intersect, always located inside the triangle. It is also the center of the inscribed circle. The centroid is found at the intersection of the three medians and is also inside the triangle, with specific segment ratios that define its location. The orthocenter's location varies depending on the type of triangle: inside for acute, on the triangle for right, and outside for obtuse.

05:00

📏 Identifying the Orthocenter

The orthocenter is the point where the three altitudes of a triangle intersect. In an acute triangle, it is inside the triangle. For a right triangle, the orthocenter coincides with the right angle vertex. In an obtuse triangle, the orthocenter is located outside the triangle. The process involves drawing altitudes from each vertex to the opposite side, ensuring they are perpendicular. The right triangle's orthocenter is specifically at the right angle, while the obtuse triangle's orthocenter is found by extending the sides and drawing the altitudes outside the triangle.

10:00

🌐 Locating the Circumcenter

The circumcenter is the point where the perpendicular bisectors of the sides of a triangle intersect. Similar to the orthocenter, its location can be inside an acute triangle, on a right triangle, or outside an obtuse triangle. To find the circumcenter, one must identify the midpoints of each side and draw lines perpendicular to these midpoints. The circumcenter is the center of the circumscribed circle around the triangle. For a right triangle, the circumcenter is at the midpoint of the hypotenuse, while for an obtuse triangle, it is outside the triangle, found by the intersection of the perpendicular bisectors.

15:02

🔍 Summary of Triangle Centers

This final paragraph summarizes the methods for identifying the incenter, centroid, orthocenter, and circumcenter of a triangle. The incenter is found at the intersection of the angle bisectors, the centroid at the medians' intersection, the orthocenter where the altitudes meet, and the circumcenter at the perpendicular bisectors' intersection. The summary emphasizes the differences in location for each center depending on the triangle's type and provides a clear review of the concepts discussed in the video.

Keywords

💡Incenter

The incenter is the point where the angle bisectors of a triangle intersect. It is always located inside the triangle and is the center of the inscribed circle, which touches all three sides of the triangle. In the video, the incenter is identified by drawing angle bisectors from each vertex to the opposite side, and the point where they intersect is the incenter.

💡Angle Bisector

An angle bisector is a line that divides an angle into two equal parts. In the context of the video, angle bisectors are used to find the incenter of a triangle. The script describes the process of drawing angle bisectors from each of the triangle's angles and how their intersection point is the incenter.

💡Centroid

The centroid is the point where the medians of a triangle intersect. It is also always located inside the triangle and represents the center of mass or balance point of the triangle. The video explains that the centroid can be found by drawing medians from each vertex to the midpoint of the opposite side.

💡Median

A median of a triangle is a line segment joining a vertex to the midpoint of the opposite side. The video script uses medians to locate the centroid, emphasizing that the centroid is where all three medians intersect, and provides a geometric property that the segment from the centroid to a vertex is twice as long as the segment from the centroid to the midpoint of the side.

💡Orthocenter

The orthocenter is the point where the altitudes of a triangle intersect. Unlike the incenter and centroid, the orthocenter's location can vary; it may lie inside, on, or outside the triangle depending on whether the triangle is acute, right, or obtuse. The video illustrates how to find the orthocenter by drawing altitudes from each vertex to the opposite side.

💡Altitude

An altitude of a triangle is a perpendicular segment from a vertex to the line containing the opposite side. The script explains that the orthocenter is found by the intersection of the altitudes and provides examples for acute, right, and obtuse triangles to show how the orthocenter's location changes with the type of triangle.

💡Circumcenter

The circumcenter is the point where the perpendicular bisectors of a triangle's sides intersect. It is the center of the circumscribed circle around the triangle. The video script describes that the circumcenter's location can be inside, on, or outside the triangle, depending on whether the triangle is acute, right, or obtuse.

💡Perpendicular Bisector

A perpendicular bisector is a line that is both perpendicular to a segment and bisects it into two equal parts. The video uses perpendicular bisectors to find the circumcenter, explaining that the circumcenter is where all three perpendicular bisectors of the triangle's sides intersect.

💡Acute Triangle

An acute triangle is a triangle where all angles are less than 90 degrees. The video script explains that in an acute triangle, the orthocenter and circumcenter are located inside the triangle, providing a visual example of how these centers are determined.

💡Right Triangle

A right triangle has one angle that is exactly 90 degrees. The script specifies that in a right triangle, the orthocenter is located at the vertex of the right angle, and the circumcenter is at the midpoint of the hypotenuse, illustrating these points with examples.

💡Obtuse Triangle

An obtuse triangle has one angle greater than 90 degrees. The video explains that for an obtuse triangle, the orthocenter and circumcenter are located outside the triangle, showing how to find these points by extending the sides and drawing altitudes and perpendicular bisectors.

Highlights

Introduction to the concepts of the centroid, orthocenter, incenter, and circumcenter of a triangle.

The incenter is always inside the triangle and is found by the intersection of the three angle bisectors.

The incenter is the center of the inscribed circle of the triangle.

The centroid is located by the intersection of the three medians of a triangle and always lies inside.

The centroid's segments have specific ratios: the long segment is twice the value of the short segment.

The orthocenter's location varies depending on the type of triangle: inside for acute, on for right, and outside for obtuse.

The orthocenter is found by the intersection of the three altitudes of a triangle.

In a right triangle, the orthocenter is located at the vertex of the right angle.

For an obtuse triangle, the orthocenter lies outside the triangle.

The circumcenter is identified by the intersection of the three perpendicular bisectors of the sides.

The circumcenter's position also varies by triangle type: inside for acute, on for right, and outside for obtuse.

In an acute triangle, the circumcenter is inside, and for a right triangle, it's at the midpoint of the hypotenuse.

In an obtuse triangle, the circumcenter is outside and can be found using perpendicular bisectors.

The incenter and circumcenter are differentiated by their respective circles: inscribed and circumscribed.

A recap of the methods to identify the incenter, centroid, orthocenter, and circumcenter of a triangle.

The importance of understanding the geometric properties and relationships of triangles for these concepts.

Transcripts

in this video we're going to talk about

the in center of a triangle

the centroid

the orthocenter and the circumcenter of

a triangle

so let's start with a picture

so let's call this

a

b

and c

how can we identify the in center

of this triangle

what would you say

in center is always inside of the

triangle

and you could find it by the

intersection of the three angle

bisectors

so let's draw an angle bisector of angle

a

it should look something like that

or maybe

more like this

and then draw the angle bisector of

c and do the same thing for

b

they should intersect at the middle

so these two angles

must be congruent

those two angles have to be congruent to

each other

and these two

must be congruent

so the point of intersection

is known as the end center

now if you draw a circle

that's inscribed

of the triangle

the in center

is the center of that circle

now granted my draw is not perfect but

if you do it perfectly

the incentive should be the center of

the inscribed circle

so let's talk about the centroid

of a triangle

how can we identify the location

of the centroid

the centroid can be found by the

intersection of

the three medians of a triangle

so let's identify the midpoint of each

side

and then draw a line from the vertex

to the midpoint of the other side

so the centroid

is approximately in that region

so like the incenser the centroid always

lies inside of the triangle

let's call this

point d

e

and f

let's call this

point p now it turns out that ap

is two thirds of a e

and p e

is one third of ae

and ap is twice the value of pe

so let's say if pe is 5

ap is 10.

if fp is 4

pc is twice the value it's eight

if bp is 12

pd is half the value of six

now the total length of ae

10 plus five that's 15.

we can see that

ap

is two-thirds of fifteen

two-thirds of fifteen is ten

p-e is one-third of fifteen

one-third of fifteen is five

and so anytime you have a centroid

the long side or the long segment will

be twice the value of the short segment

so to review

the centroid

inside a triangle can be identified

by the intersection of the three medians

so if a e is the median

that means e

is the midpoint of bc

which means that b e

and e c are congruent

now bd is the median to ac so d is the

midpoint of ac so a d

and dc have to be congruent

f is the midpoint of a b since fc is the

median

so fb and af are congruent

and so that's it for a centroid

let's move on to our next topic

so let's focus on identifying

the orthocenter

of a triangle

now the orthocenter

doesn't have to lie

inside of the triangle it could be on

triangle or outside of the triangle

now if we have an acute triangle

like this particular example

it's going to lie inside of the acute

triangle

if we have a right triangle it lies on

the right triangle

and if we have an obtuse triangle it's

going to lie outside of the obtuse

triangle

now the location of the orthocenter

can be found by the intersection of the

three altitudes

so let's draw an altitude from vertex a

to the opposite side side bc

so we need to draw in such a way that

it's perpendicular to the opposite side

which is probably going to be around

here

it looks perpendicular at that point

and then do the same for vertex c

so around there should be perpendicular

and then

let's draw a line from b to ac

so as we can see the orthocenter is

approximately somewhere in this region

so if you have an acute triangle

the orthocenter

lies inside of the acute triangle

now let's focus on a right triangle

let's identify the location

of the orthocenter

so first let's extend each side

so i'm going to extend side bc

side a b

and also

ac

i probably don't need to do that but i'm

going to do it anyway

so

what we need to do is draw an altitude

from vertex a to bc

and notice that the altitude is here

because that's where it meets bc at a

right angle

so this is the altitude from vertex a to

bc

now let's draw the altitude

from vertex c to side a b

so that's going to be here

because it's perpendicular to side a b

now let's draw the altitude from vertex

b

to ac

so that's going to be

actually let's do that again

it should be like this

okay this is approximately a right angle

it's not exactly 90 but it's close to it

either case you could see that

this

is the location of the ortho center

the orthocenter is located at the right

angle of a right triangle

so let's say if this is the right

triangle

the orthocenter will be located at the

right angle

or if we have another right triangle

that looks like this

the orthocenter

is going to be located right there

and so that's how you can identify the

orthocenter of a right triangle

it's always located on the right

triangle at the right angle

now what if we have

an obtuse triangle

so let's call this a

b

and c

so i'm going to extend

each

side this is bc

and here we have

a b

and let's extend

ac let me do that

again so

let's draw the altitude starting from

vertex b to the opposite side

ac i'm going to do it in blue

so this is a right angle

and then let's draw

the altitude from vertex a

to side

bc

so remember it has to be perpendicular

so notice that it's perpendicular

at bc at this point

if you draw it here

this is not going to be a 90 degree

angle

so

for an obtuse triangle we could see that

the orthocenter is going to lie outside

of the obtuse triangle

now let's try the third line

and it's going to be from vertex c

perpendicular to a b

and so we see here it's approximately 90

degrees

and so they intersect at that region

so that's the orthocenter

of an obtuse triangle

an obtuse triangle is a triangle that

has

one angle that's a greater than 90. so

this angle is more than 90. it could be

like maybe 110 that makes it an obtuse

triangle

so now you know how to identify an

orthocenter it's the location where all

three altitudes of a triangle intersect

now there's one more term that we need

to go over and that is the circumcenter

so how can we identify

the location of the circumstance

let's call this triangle abc

the circumcenter

can be identified

from the intersection of the three

perpendicular bisectors

of the three sides of a triangle

and it's very similar to the orthocenter

in that it doesn't always have to lie on

the inside of the triangle

now if you have an acute triangle

the circumcenter is going to lie on the

inside

of the acute triangle

if you have a right triangle

it lies on the right triangle

and if you have an obtuse triangle

it will be located

outside

of the obtuse triangle

so first let's identify the midpoint of

each side

a perpendicular bisector is basically

the combination of an altitude and a

medium it has properties of both

so the perpendicular bisector for ac

would be here

it doesn't have to touch a vertex b

even though it may look like it's going

to touch it

but however

these two sides must be congruent

because this point has to be the

midpoint

and also

the two lines have to be perpendicular

to each other

so let's draw something that's

perpendicular to

this side

okay that's approximately perpendicular

to it

and these two sides are congruent

and that's approximately perpendicular

to this side

so we can see that the circumcenter

is located on the inside of an acute

triangle

now let's draw a circle

okay that circle wasn't looking good

so notice that

the circumcenter

is the center of a circle that is

circumscribed about the triangle

the in center of a triangle

is the center of a circle that is

inscribed

of a triangle so hopefully you see the

difference between a circumcenter and an

incenter so just to recap

the circumcenter of a triangle

is the center of the circle when the

circle is circumscribed about the

triangle

and the in center of a triangle

is the center of the circle

when the circle is inscribed or inside

of the triangle so hopefully that makes

sense

now let's move on to our next example

and that is the right triangle

so where is the circumcenter

of the right triangle

so let's identify the three midpoints

and then draw

a

perpendicular line to each

to each

midpoint

so we have a perpendicular bisector

so as you can see

the circumcenter lies on the right

triangle

in fact

it's at the midpoint of the hypotenuse

so this line is perpendicular to a b

and this is the perpendicular bisector

of bc

and here we have the perpendicular

bisector of ac

so remember that the circumcenter

can be found by

the intersection of the three

perpendicular bisectors

now let's draw the circle

so as we can see

the circumcenter lies on the center of

the circle that's circumscribed about

the right triangle

now let's move on to our next example

and that is

the obtuse

triangle

so this is a

b and c

and so first let's identify the midpoint

of

each side

and then let's draw the perpendicular

bisectors

of those midpoints

actually that shouldn't be there

so we can see this is the right angle

this is a right angle

and that's right angle

and then

this point is the midpoint of a b

these two sides are congruent and those

two sides are congruent

so notice that

the circumcenter lies outside

of the obtuse triangle

now let's draw the circle

it should be something like that

but as you can see my circles is not

perfect but if it was

you can see that this should be the

center

of the circle that's circumscribed about

triangle abc

so now let's review what we've learned

so far

the in center of a triangle

can be found by the intersection

of the three angle bisectors

next we talked about the centroid

the centroid

can be found by the intersection of the

three

medians

of a triangle

and if you wish to find the orthocenter

you can do so by finding where

the three altitudes meet

and finally

to identify the location of the

circumstances

you need to draw the three perpendicular

bisectors

so hopefully that gave you a good review

and so now you know how to identify

the in center of a triangle the centroid

the orthocenter and the circumcenter

so thanks again for watching

you