Triangle Centers Identification

Summary

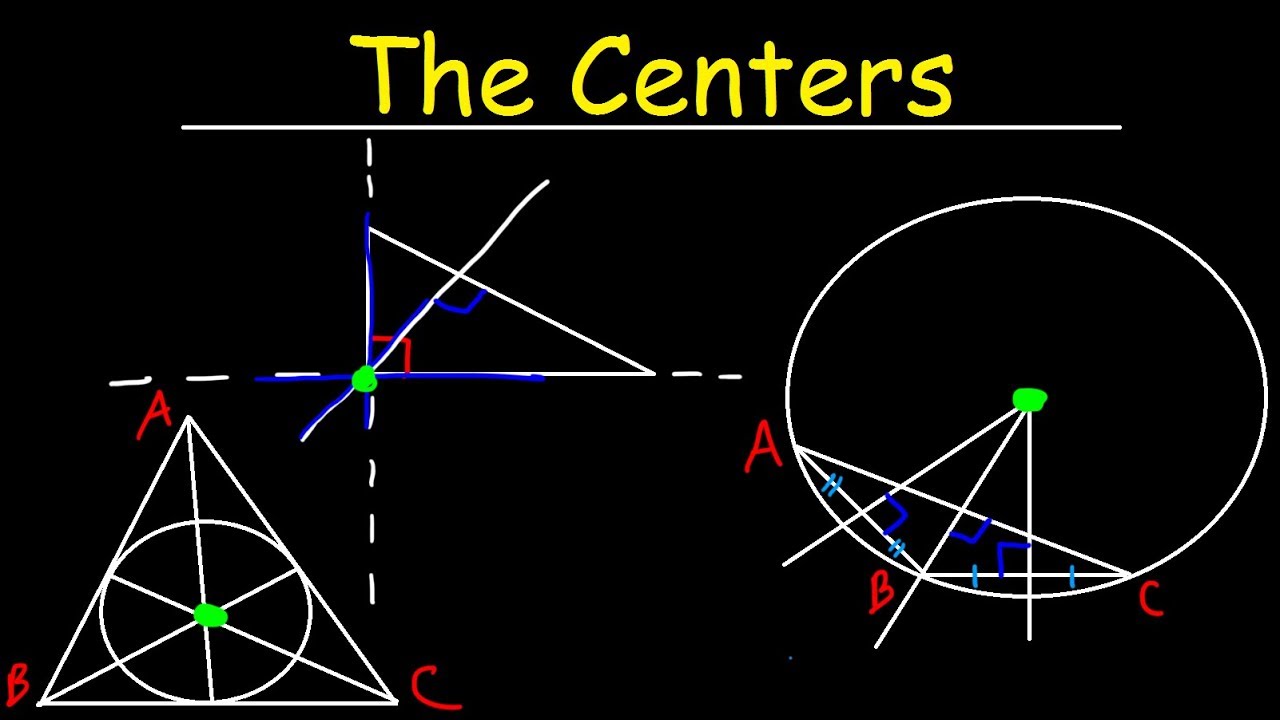
In this episode of 'Math with Sewn,' the focus is on identifying and distinguishing between the various triangle centers: circumcenter, incenter, centroid, and orthocenter. The video explains how each center is determined by specific geometric properties such as perpendicular bisectors, angle bisectors, and medians. It also highlights the congruent relationships that characterize each center. The script provides practical tips for recognizing these centers by examining the angles and sides of triangles, and it includes practice problems to reinforce the concepts discussed.

Takeaways

• 📐 The circumcenter is found by the intersection of the perpendicular bisectors of a triangle's sides.
• 🔄 The circumcenter creates equal angles from the vertices to the center, forming congruent triangles.
• 📏 The incenter is located at the intersection of the angle bisectors, creating congruent segments from the sides to the center.
• 🔺 The centroid is found by the intersection of the medians, which connect the midpoints of the sides to the opposite vertices, and it does not involve 90-degree angles.
• ⚖️ The centroid has a special relationship where the segments from the midpoints to the centroid are proportional to the sides of the triangle.
• 📍 The orthocenter is the intersection of the triangle's altitudes, and it is characterized by 90-degree angles.
• 🔶 The orthocenter does not create congruent triangles and has no proportional relationship between the sides.
• 🧩 To identify the triangle centers, one can draw lines to make 90-degree angles and check if angles or sides are bisected.
• 🔍 Practice problems in the script involve identifying the type of center based on given geometric properties.
• 📚 The script suggests using a reference guide to compare the properties of each center when solving problems.
• 🌟 The video concludes with a reminder to stay positive and look forward to the next episode.

Q & A

What is the circumcenter of a triangle?

The circumcenter of a triangle is the point where the perpendicular bisectors of the sides intersect. It is equidistant from all the vertices of the triangle.

How are the perpendicular bisectors related to the circumcenter?

The perpendicular bisectors of the sides of a triangle create equal segments from the center to the midpoints of the sides, and they intersect at the circumcenter.

What is the incenter of a triangle?

The incenter of a triangle is the point where the angle bisectors of the triangle's angles intersect. It is the center of the inscribed circle that is tangent to each side of the triangle.

How does the incenter differ from the circumcenter in terms of congruence?

Unlike the circumcenter, the incenter does not create congruent triangles from the sides or angles. Instead, it is associated with the angle bisectors and the congruence is from the side to the center.

What is the centroid of a triangle?

The centroid of a triangle is the point where the medians intersect. It is also the center of mass or balance point of the triangle.

How is the centroid found in relation to the sides of the triangle?

The centroid is found by drawing medians from each vertex to the midpoint of the opposite side, and the intersection of these medians is the centroid.

What is the orthocenter of a triangle?

The orthocenter of a triangle is the point where the altitudes of the triangle intersect. It is the vertex of the orthic triangle.

Why is the orthocenter sometimes referred to as the 'weird uncle' of triangle centers?

The orthocenter is sometimes called the 'weird uncle' because it does not have as many distinct properties or congruences as the other triangle centers, and it is less commonly discussed.

How can you identify the circumcenter in a problem with given information?

You can identify the circumcenter by looking for the point that is equidistant from the vertices of the triangle, often indicated by perpendicular bisectors or congruent angles to the center.

What is a characteristic of the centroid that helps distinguish it from other triangle centers?

The centroid is characterized by the fact that it does not have any 90-degree angles associated with its construction, unlike the circumcenter and orthocenter.

How can you use the given information in a problem to determine if a point is the incenter?

If the problem indicates that the angles are bisected and the resulting segments are congruent from the side to the center, then the point is likely the incenter.

What is a strategy for solving problems involving the identification of triangle centers?

A strategy is to draw the lines that would create 90-degree angles and then determine whether the sides or angles were bisected, which can help identify the circumcenter, incenter, centroid, or orthocenter.

Outlines

00:00

📚 Introduction to Triangle Centers

This paragraph introduces the topic of triangle centers, explaining the concept of circumcenter, incenter, centroid, and orthocenter. The circumcenter is created by the perpendicular bisectors of the sides, which results in equal angles from the circumcenter to the vertices. The incenter is formed by the angle bisectors, creating congruent segments from the sides to the center. The centroid is found by the medians of the triangle, which connect the midpoints of the sides to the opposite vertices, and it does not have any congruent triangles. Lastly, the orthocenter is the intersection of the triangle's altitudes, characterized by 90-degree angles and not having congruent triangles. The paragraph also suggests practicing identifying these centers based on given information.

05:01

🔍 Identifying Triangle Centers Through Practice Problems

This paragraph delves into practice problems to help viewers identify the different triangle centers. It suggests a methodical approach: first, determine if angles or sides are bisected and if there are 90-degree angles present. The presence of 90-degree angles and sides bisected indicates the circumcenter, while the centroid is identified by medians without 90-degree angles. The incenter is recognized by angle bisectors creating congruent triangles from the corners. The orthocenter is unique as it is where the altitudes intersect, with 90-degree angles but no congruent triangles. The paragraph concludes with a reminder to use the reference guide to solidify understanding of the characteristics of each triangle center.

Keywords

💡Circumcenter

The circumcenter is the point where the perpendicular bisectors of a triangle intersect. It is the center of the circle that passes through all the vertices of the triangle, hence the name circumcenter. In the video, it is described as being created by the perpendicular bisectors of the sides of the triangle, which cut the sides in half and go straight up at a 90-degree angle. The video emphasizes that the distances from the circumcenter to the vertices are equal, which is a key characteristic of the circumcenter.

💡Perpendicular Bisectors

Perpendicular bisectors are lines that divide a line segment into two equal parts at a 90-degree angle. In the context of the video, they are used to define the circumcenter of a triangle. The script mentions that the circumcenter is found using the perpendicular bisectors, which cut the sides of the triangle in half and create equal segments from the vertices to the circumcenter.

💡Incenter

The incenter is the point where the angle bisectors of a triangle meet. It is the center of the triangle's inscribed circle, which is tangent to all three sides of the triangle. The video explains that the incenter is created by bisecting each angle of the triangle, resulting in congruent segments from the sides to the incenter. Unlike the circumcenter, the incenter does not involve 90-degree angles.

💡Angle Bisectors

Angle bisectors are lines that divide an angle into two equal parts. They are used to locate the incenter of a triangle, as mentioned in the video. The script describes the process of bisecting the angles to find the incenter, which is different from the process used to find the circumcenter, which involves perpendicular bisectors.

💡Centroid

The centroid is the point where the medians of a triangle intersect. It is also the center of mass of the triangle and is located two-thirds of the way from each vertex to the midpoint of the opposite side. The video describes the centroid as being found by taking the medians of the triangle, which are lines drawn from the midpoint of each side to the opposite vertex. The centroid does not involve 90-degree angles, unlike the circumcenter and orthocenter.

💡Medians

Medians are lines that connect the midpoints of the sides of a triangle to the opposite vertices. They are used to locate the centroid of a triangle, as explained in the video. The script mentions that the centroid is found by drawing medians from the midpoints of the sides to the opposite vertices, creating a point where the medians intersect.

💡Orthocenter

The orthocenter is the point where the altitudes (or heights) of a triangle intersect. It is one of the triangle centers and is characterized by the 90-degree angles formed by the altitudes. The video describes the orthocenter as the 'weird uncle' of triangle centers because it does not have as many congruent relationships as the other centers. It is found by taking the heights of the triangle, which are the perpendicular lines from the vertices to the opposite sides.

💡Altitudes

Altitudes, also known as heights, are the perpendicular lines drawn from a vertex to the line containing the opposite side of a triangle. They are used to locate the orthocenter, as mentioned in the video. The script explains that the orthocenter is found at the intersection of all the altitudes of the triangle, which are lines that go straight up from each vertex at a 90-degree angle.

💡Congruence

Congruence in geometry refers to the property of two figures being identical in shape and size. In the context of the video, congruence is used to describe the relationships between the triangle centers and the sides or angles of the triangle. For example, the circumcenter creates congruent segments from the vertices to the center, and the incenter creates congruent segments from the sides to the center.

💡Practice Problems

Practice problems are exercises given in the video to help viewers apply their understanding of the concepts discussed. The script includes several practice problems that challenge the viewer to identify the triangle centers based on the characteristics described. These problems are designed to reinforce the concepts of circumcenter, centroid, incenter, and orthocenter by asking viewers to analyze given geometric configurations.

Highlights

Introduction to the concept of triangle centers and their identification.

Explanation of the circumcenter as the intersection of perpendicular bisectors of the sides.

Description of the properties of the circumcenter, including the creation of equal triangles.

Clarification on how the incenter is created by the angle bisectors of the triangle.

Identification of the congruent parts in the incenter, which are from the side to the center.

Discussion on the centroid, its relation to the medians of the triangle, and the absence of 90-degree angles.

Explanation of the orthocenter as the intersection of the triangle's altitudes.

Characteristics of the orthocenter, including the presence of 90-degree angles and the lack of congruent triangles.

Introduction to practice problems to identify the different triangle centers.

Method to determine the incenter by bisecting the angles of the triangle.

Strategy for identifying the orthocenter by looking for 90-degree angles and altitude intersections.

Technique to find the centroid by connecting the midpoints of the sides to the opposite vertices.

Advice on drawing lines to make 90-degree angles to help identify the circumcenter.

Illustration of how to use congruent triangles to identify the incenter.

Final review and summary of the characteristics and identification methods for triangle centers.

Encouragement to practice and stay positive in learning about triangle centers.

Transcripts

[Music]

hello and welcome back to another

episode of math with sewn today we are

going to be looking at

all of the triangle centers seeing and

comparing how they're different

figuring out how to identify them given

specific information

okay so let's get into it the

circumcenter

is going to be created by the

perpendicular bisectors so they take the

sides and they cut them in half and they

go straight up with a 90 degree angle

so it is found with the perpendicular

bisectors

that is how it is created

but because these are the perpendicular

bisectors that is creating the triangles

that are equal

from the sides right here and the sides

would create this scenario where from

the angle to the circumcenter and from

the angle to the circumcenter and from

this angle to the circumcenter would be

equal

okay so the circumcenter creates that

scenario

now i put a single hash mark here i

should have put one two three four

and one two three four because we've

already used so many hash marks we've

already used

one two and three up so i had to use the

four

which is almost absurd so perpendicular

bisectors

and the parts that are equal are from

the center

to the corners of the angle

so you cut the sides in half with a 90

degree angle and it's from the center

to the corners that are congruent the in

center however is created by the angle

bisectors or you cut the corners in half

so you got to bisect each corners it is

created by

the angle bisector and

the part that's congruent is here

from the side to the center from the

side to the center and from the side of

the center

the triangles that are congruent are

from the corners

as well not from the sides

and that the centroid

is having zero triangles that are

congruent

it is a little more recognizable because

there's no 90 degree angles in it either

notice how the other two had 90 degree

angles

this one does not so the centroid

is found by just taking the sides and

going

straight up it is created by the medians

of the triangle which is going from the

midpoint straight to the other angle

not straight up but straight to the

other angle and it does create that

special relationship where

if this is 20 that part would be 10 it

is half two times the short part equals

the longer part

new one for most people is probably

going to be the ortho center orthocenter

is kind of

no it's the weird uncle nobody talks

about orthocenter doesn't have a lot

going on there's not much that it

actually does

um it is the height

of the triangle which is kind of neat

because if we were going straight

here that would be how tall this

triangle is so it's found

by taking the heights it's the

intersections of all the heights

of the triangle which is also called the

altitude

i like height of the triangle

it does have 90 degree angles because in

order to go straight up you got to go

straight up being perpendicular to the

bottom of it

and that is all that really

characterizes the orthocenter there's no

congruent triangles at all there's no

relationship between the short and

longer side at all

and it's just where they intersect from

the heights or altitudes

okay so we're going to take a few

practice problems and we're going to see

which if we can identify which is which

okay

so here are some practice problems

we have to identify what's happening and

if we can identify what's happening then

we're going to be able to name

that center so we're going to call it

the circumcenter the

centroid or the in center let's figure

out which one's which

let's fix the zoom there we go zoom

better so here it looks like we took the

angles and cut them in half and then we

got to figure out which one cuts the

angles in half

and the angles are cut in half from the

in

center so you'd have to go back to your

reference guide and figure out which one

cuts the angles in half

here the only thing we're given

is that these are 90 degrees and it goes

straight up every time so

which one has 90 degrees here and goes

straight to the other side so there's

a few of them that connect the side all

the way to the angle straight

and that would either be the centroid or

the orthocenter the orthocenter goes

straight with a 90 degree where the

centroid doesn't have to

so this one right here is the

orthocenter

three we took the angles we are escaped

we took the sides we cut them in half

and they're not with a 90 degree angle

so it's not going to be anything with a

90 degree angle and that means that

there's only one possibility

this must be the centroid because we

took the middle

and we went straight to the other angle

number four here

we did take we we bisected the side so

which one bisects the sides

with a 90 degree angle perpendicular

bisector is going to be the

circumcenter

keeping our triangles right along here

it looks like we have we went from this

the angles straight over and the angle

straight over

so what i would suggest when you have

problems like this and you might not be

sure

draw the line that would make these

90 degrees and then determine

whether or not the sides were cut in

half or the angles were cut in half

so in this case

it doesn't look like these two triangles

right here and here are really congruent

and it really looks like these are the

ones that are congruent

and that means that this side had to

have been bisected

with a 90 degree angle that means that

this

again is the circumcenter

and if we go back back to our reference

guide that was what we established with

our circumcenter that from the angle to

the circumcenter

is congruent the in center it's from the

side

the side to the in center is congruent

excuse me

number six it looks exactly like number

four or

number three did this is the centroid

you found the middle of each one and you

went straight to the other angle

what about number seven

hmm number 7

doesn't have a lot of information here

and i believe that the problem meant to

say that those are congruent

so if these are congruent this is going

to be the

in center and if i drew my line straight

to

the angles here you can kind of see

how these triangles that i'm making from

each corner these triangles all of these

ones coming from the corners here and

here they're going to be equal

this is by far the in center

and that in center it's from the side to

the center and from the side to the

center is going to be the equal part

what do we got here we got not cutting

the sides in half not cutting the angles

in half we are looking like

this is the height of the triangle we

went with a 90 degree angle straight to

the other corner

so this is going to be the ortho center

all right so that's just a quick review

of

all of them and a little way to identify

them

and get a little bit more

characteristics built into our like

structure of

what a triangle center is short video

until next time have a great day stay

positive

i will see everybody later