Bissetrizes e Incentro - MAT GIO: Matemática com Giovanna
Summary
TLDRThis math lesson, led by Giovana, explores key concepts related to triangles, specifically focusing on bisectors and notable points. She explains how angle bisectors divide angles into two equal parts and how the point of intersection of these bisectors, called the incenter, is significant. The incenter is the center of the inscribed circle that touches all three sides of the triangle. The video also covers the properties of the incircle, tangency, and the calculation of the radius. The lesson progresses to exercises, applying the concepts to solve problems involving angles and triangle geometry.
Takeaways
- 😀 The script introduces the topic of notable segments and points in a triangle, specifically focusing on the bisector of an angle.
- 😀 The bisector is a ray that divides an angle into two equal parts, with its endpoint at the opposite side of the triangle, not necessarily at the midpoint.
- 😀 A triangle can have three bisectors, one from each angle. These bisectors intersect at the incenter, the center of the inscribed circle.
- 😀 The incenter is equidistant from all three sides of the triangle, and it is the center of the circle that touches all three sides at exactly one point.
- 😀 The radii of the inscribed circle are perpendicular to each side of the triangle, creating a 90-degree angle where they meet the sides.
- 😀 The incenter plays an important role in geometry, specifically when calculating the radius of the inscribed circle in later lessons.
- 😀 In a given example, the bisectors divide the angles into smaller parts, and the angles' measures are used to solve for missing values in the triangle.
- 😀 A key equation to remember is that the sum of angles in any triangle equals 180 degrees.
- 😀 The concept of alternate interior angles is applied when dealing with parallel lines and bisectors, helping identify congruent angles in the figure.
- 😀 The perimeter of a smaller triangle within the original triangle is calculated by summing the lengths of its sides, leveraging known values and relationships between the angles and bisectors.
Q & A
What is a bisector in the context of a triangle?
-A bisector in a triangle is a segment of a line that divides an angle of the triangle into two equal parts, originating from the vertex and reaching the opposite side.
How does the bisector of an angle in a triangle behave?
-The bisector divides the angle into two equal parts and reaches the opposite side, but it does not necessarily meet at the midpoint of the side.
What is the point where the bisectors of a triangle meet?
-The point where the bisectors of a triangle meet is called the incenter, which is the center of the inscribed circle that touches all three sides of the triangle.
What does the incenter represent in a triangle?
-The incenter represents the center of the inscribed circle in the triangle. This circle tangentially touches all three sides, with the distance from the incenter to each side being equal.
What is the relationship between the incenter and the sides of a triangle?
-The incenter is equidistant from all three sides of the triangle, and this distance is the radius of the inscribed circle.
What is the significance of the radius of the inscribed circle in a triangle?
-The radius of the inscribed circle is the perpendicular distance from the incenter to any side of the triangle.
What happens when two parallel lines are cut by a transversal in the context of bisectors?
-When two parallel lines are cut by a transversal, corresponding angles are congruent. This property is used when bisectors are involved, as certain angles formed by bisectors and parallel lines will be equal.
How does the concept of isosceles triangles apply to the bisectors in this lesson?
-In the context of bisectors and parallel lines, an isosceles triangle is formed where two angles are congruent, meaning the opposite sides are also congruent.
What is the method used to calculate the perimeter of a triangle involving bisectors?
-The perimeter of the triangle is calculated by adding the lengths of the sides. In some cases, the lengths of the sides can be represented by variables, and the perimeter can be derived algebraically by solving the system of equations.
How do the properties of bisectors help in solving geometry problems?
-The properties of bisectors, such as dividing angles into equal parts and leading to the incenter, provide a foundation for solving various geometric problems, including calculating distances, angles, and perimeters.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Toán lớp 7 Bài 6 Tính chất ba đường trung trực của tam giác | trang 71, 72 | Chương 8 | Tập 2 | CTST

Triangle Centers Identification

CRITERI DI CONGRUENZA DEI TRIANGOLI, criterios de congruencia de triangulos, triangoli congruenti

teorema pythagoras kelas 8 "bagian 4" (mudah): menentukan perbandingan sisi-sisi segitiga siku-siku
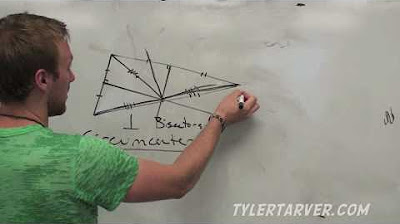
Bisectors of Triangles // GEOMETRY

MATEMATIKA KELAS 8 HALAMAN 142-143 KURIKULUM MERDEKA EDISI 2021
5.0 / 5 (0 votes)