TRIGONOMETRI SUDUT ISTIMEWA
Summary
TLDRIn this educational video, the presenter provides a clear and step-by-step explanation of solving trigonometric problems involving special angles (0°, 30°, 45°, 60°, and 90°). Using values from the table of special angles, the presenter demonstrates how to simplify expressions with sine, cosine, and cotangent functions. The tutorial covers five different problems, breaking each one into manageable steps for students at various educational levels (SD, SMP, SMA). The video aims to make trigonometry easier to understand while encouraging viewers to engage with the channel through likes, comments, and shares.
Takeaways
- 😀 Trigonometry can be easy if you understand special angles like 30°, 45°, and 60°.
- 😀 The video provides a step-by-step breakdown of solving five trigonometric problems.
- 😀 Always start by recalling the standard values of sine, cosine, and cotangent for special angles.
- 😀 Simplification techniques like factoring out common terms can make solving problems easier.
- 😀 The video encourages viewers to submit difficult math problems for free help via their account.
- 😀 Understanding trigonometric identities is essential for tackling problems with ease.
- 😀 The problems focus on using basic trigonometric functions like sine, cosine, and cotangent.
- 😀 The importance of simplifying expressions and factoring common terms to solve problems quickly is highlighted.
- 😀 The problems demonstrate various operations such as addition, multiplication, and division of trigonometric functions.
- 😀 The video concludes with a reminder to like, subscribe, and share, wishing viewers the best in their learning journey.
Q & A
What are special angles in trigonometry?
-Special angles in trigonometry refer to specific angles between 0° and 90° whose sine, cosine, and other trigonometric ratios have exact known values. These angles include 0°, 30°, 45°, 60°, and 90°.
How do you calculate Sin 60° + Cos 45°?
-To calculate Sin 60° + Cos 45°, you use their known values: Sin 60° = √3/2 and Cos 45° = √2/2. The sum is then written as (√3 + √2)/2.
What is the result of Sin 30° * Sin 45° + Cos 30° * Sin 45°?
-First, calculate the individual sine and cosine values: Sin 30° = 1/2, Sin 45° = √2/2, and Cos 30° = √3/2. Multiply and add them to get the result: (1/4)(√2 + √6).
How do you simplify the expression Sin 60° * Cos 30° - Cos 60° * Sin 30°?
-To simplify this expression, substitute the known values: Sin 60° = √3/2, Cos 30° = √3/2, Cos 60° = 1/2, and Sin 30° = 1/2. The result simplifies to (1/4)(3 - 1) = 1/2.
How do you compute Sin 45° * Sin 60° / Cot 45°?
-Using the known values: Sin 45° = √2/2, Sin 60° = √3/2, and Cot 45° = 1. The calculation simplifies to (1/4)√6, as dividing by 1 keeps the expression unchanged.
What are the important steps to solve a trigonometric problem like Sin 60° + Cos 45°?
-First, recognize the known values of the trigonometric functions. Then, perform the necessary arithmetic operations. Finally, simplify the result by factoring out common terms if needed.
Why is it important to understand special angles in trigonometry?
-Understanding special angles is crucial because they provide exact values for trigonometric functions, making calculations easier and faster, especially in problems involving trigonometric identities and equations.
What does the term 'special angles' refer to in trigonometry?
-'Special angles' refer to angles whose trigonometric values (like sine, cosine, tangent) are easy to remember and calculate, such as 0°, 30°, 45°, 60°, and 90°.
How can we calculate trigonometric values for angles other than the special angles?
-For angles other than special angles, you typically use a calculator or reference table, or apply trigonometric identities and formulas, such as the sine and cosine addition formulas.
What role does simplification play in solving trigonometric problems?
-Simplification helps in reducing complex expressions into simpler forms, making them easier to understand and solve. It often involves factoring, combining like terms, or using trigonometric identities.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео
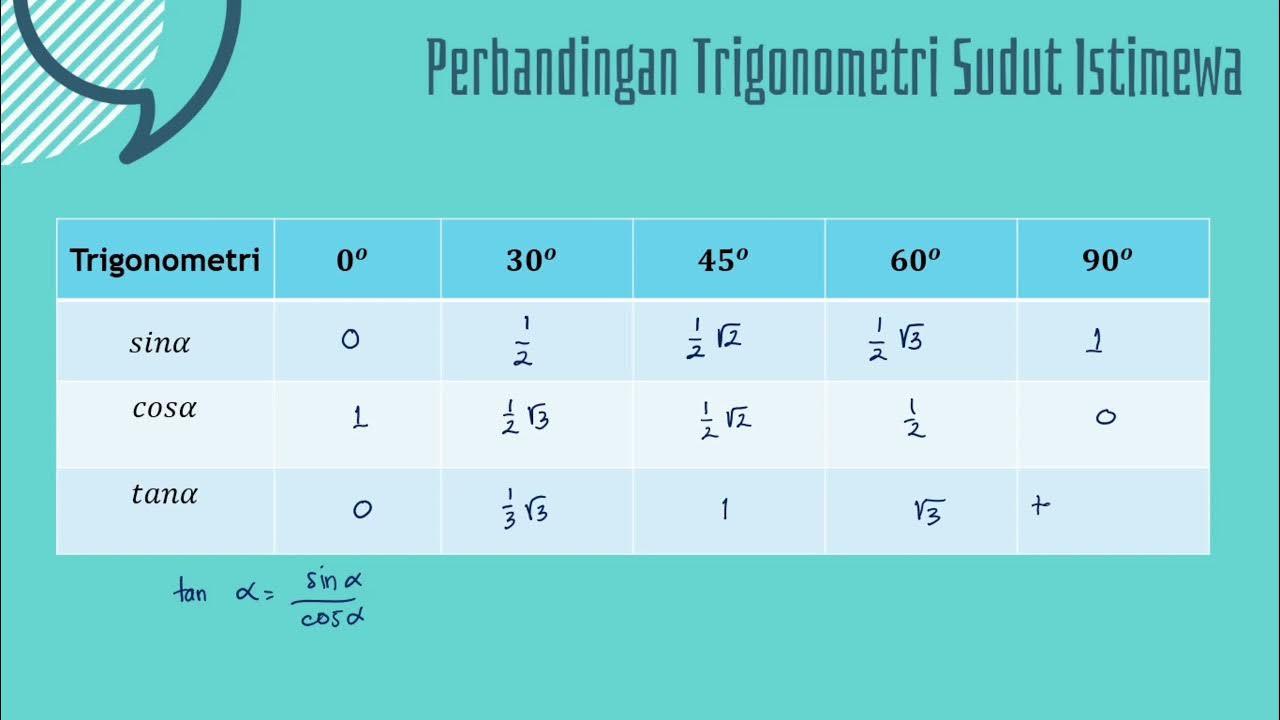
Perbandingan Trigonometri Sudut Istimewa
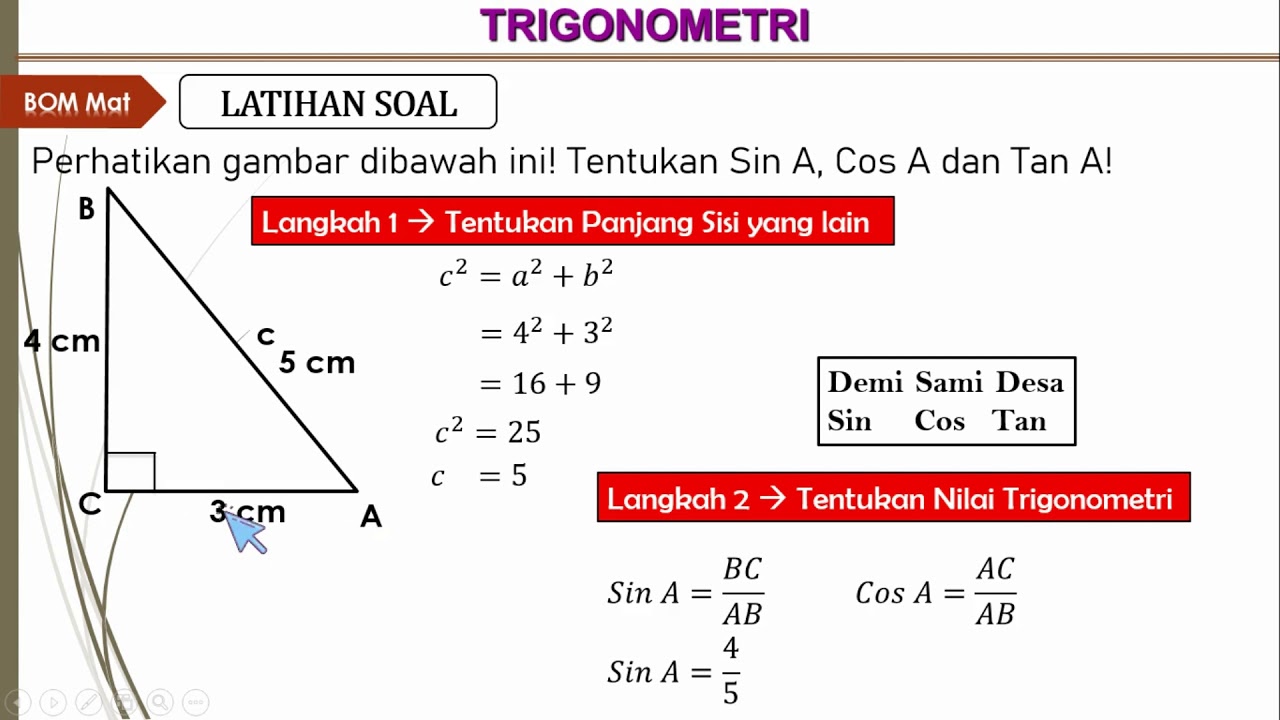
Perbandingan trigonometri pada segitiga siku siku, Menjelaskan rasio trigonometri
Materi Trigonometri

Video Pembelajaran Matematika Materi Trigonometri Kelas 10 - Nilai Trigonometri pada Sudut Istimewa

Trigonometri Matematika Kelas 10 • Part 4: Perbandingan Trigonometri Sudut Istimewa

TRIGONOMETRI ( PERBANDINGAN TRIGONOMETRI DLM SEGITIGA SIKU SIKU & SUDUT SUDUT ISTIMEWA )
5.0 / 5 (0 votes)
