Relativitas • Part 1: Kecepatan Relatif dan Postulat Einstein Tentang Relativitas Khusus

Summary

This video explains key concepts of Einstein’s theory of special relativity, focusing on relative velocity and the two postulates of special relativity. The video uses real-world examples, such as the motion of a train and spaceships approaching a planet, to illustrate how motion is relative to the observer's frame of reference. Viewers learn how to calculate relative velocities both in everyday scenarios and relativistic contexts. The video also covers important formulas, demonstrating how they apply to both classical and relativistic motion, ensuring a clear understanding of these fundamental physics concepts.

Takeaways

• 😀 Motion is relative, meaning the movement of an object depends on the observer's reference frame.
• 😀 The speed of an object is described relative to a specific reference point, and different observers may perceive different speeds.
• 😀 The formula for relative velocity is: v_AB = v_A - v_B, where v_A and v_B are the velocities of objects A and B respectively.
• 😀 When a person inside a train moves, their speed relative to an observer on the platform depends on the direction of motion (same or opposite direction).
• 😀 If an observer is stationary at the train station, the relative speed of a worker moving inside the train will be the sum or difference of their speeds depending on the direction.
• 😀 Einstein's first postulate of special relativity states that the laws of physics are the same in all inertial reference frames.
• 😀 The second postulate of special relativity asserts that the speed of light in a vacuum is constant (3 x 10^8 m/s) and is independent of the motion of the light source or observer.
• 😀 Classical velocity addition does not work at speeds close to the speed of light. Relativistic velocity addition must be used for objects moving near light speed.
• 😀 For two objects moving towards each other at high speeds, the relativistic velocity addition formula ensures their relative velocity never exceeds the speed of light.
• 😀 In relativistic calculations, when speeds are near the speed of light, the formula becomes v_AB = (v_B - v_A) / (1 - (v_A * v_B) / c^2), ensuring the speed limit of light is respected.

Q & A

What is the main topic discussed in this video?

The video focuses on the concept of relativity, specifically Einstein's postulates for special relativity and the concept of relative velocity.

How does the concept of relative motion apply to the example of a train and a person standing by the tracks?

According to the video, both the observer on the train and the observer by the tracks can perceive motion differently. The person by the tracks sees the train moving, while the person on the train sees the trees moving backwards. This illustrates that motion is relative to the observer's reference frame.

What is the equation for relative velocity introduced in the video?

The equation for relative velocity is: v_app = v_a - v_b, where v_app is the relative velocity, v_a is the velocity of object A, and v_b is the velocity of object B relative to A.

What is the significance of the minus sign in the relative velocity equation?

The minus sign indicates that when objects move in opposite directions, their velocities are subtracted, whereas when they move in the same direction, their velocities are added.

Can you explain the example involving the train conductor and the speed of the train?

In this example, a train is moving at 20 m/s. If a conductor inside the train walks at 2 m/s in the same direction, the speed of the conductor relative to an observer at the station is 22 m/s. If the conductor walks in the opposite direction, the speed relative to the observer at the station would be 18 m/s.

What are Einstein’s two postulates of special relativity?

Einstein’s first postulate states that the laws of physics are the same in all inertial frames of reference. The second postulate states that light travels at the same speed, c, in a vacuum, regardless of the motion of the source or the observer.

How does the video explain the application of relativity when objects are moving at speeds close to the speed of light?

The video mentions that the relativistic velocity formula, which is v_app = (v_a + v_b) / (1 + (v_a * v_b / c²)), should be used when objects move at speeds approaching the speed of light, c. This formula ensures that the speed of light is never exceeded.

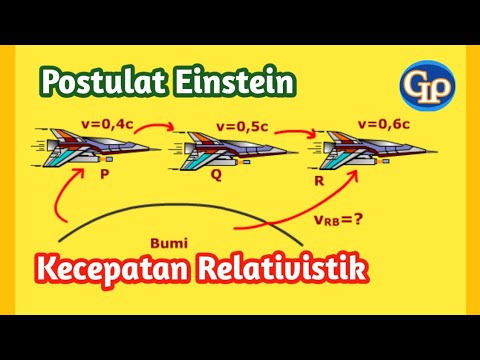
What happens when two spacecraft are moving toward a planet at high velocities?

When two spacecraft are moving toward a planet at high velocities, such as 0.6c and 0.8c, the relative velocity between the two spacecraft is calculated using the relativistic formula. This ensures that the relative velocity does not exceed the speed of light, adhering to the laws of relativity.

What is the result of using the relativistic velocity addition formula in the case of the two spacecraft moving toward each other?

Using the relativistic velocity addition formula, the relative velocity of the two spacecraft is calculated as 1.4c, which cannot exceed the speed of light. The result is adjusted to comply with the theory of relativity, where the relative velocity is found to be approximately 0.35c.

Why does the video suggest that the speed of the train conductor relative to the observer on the station can be treated with simple addition or subtraction in most cases?

Since the speeds involved in most everyday scenarios, such as the speed of the train conductor (2 m/s) and the train (20 m/s), are much smaller than the speed of light, the simple addition or subtraction of velocities works well. However, for speeds approaching the speed of light, relativistic formulas must be applied.