5 Ideal Gas Law Experiments - PV=nRT or PV=NkT

Summary

This video explores five engaging experiments based on the ideal gas law, demonstrating the relationships between pressure, volume, and temperature. Viewers will see experiments with balloons, canned air, marshmallows, and a bike pump, each illustrating key principles like Boyle's Law, Charles' Law, and Gay-Lussac’s Law. The experiments showcase practical applications, such as how cooling a balloon shrinks it, or how compressing gas increases temperature. The video also highlights the importance of safety while handling materials like compressed air and introduces simple, accessible experiments to help viewers grasp fundamental gas behavior in an entertaining way.

Takeaways

• 😀 The Ideal Gas Law equation (PV = NRT) governs the relationship between pressure, volume, temperature, and the amount of gas in a system.
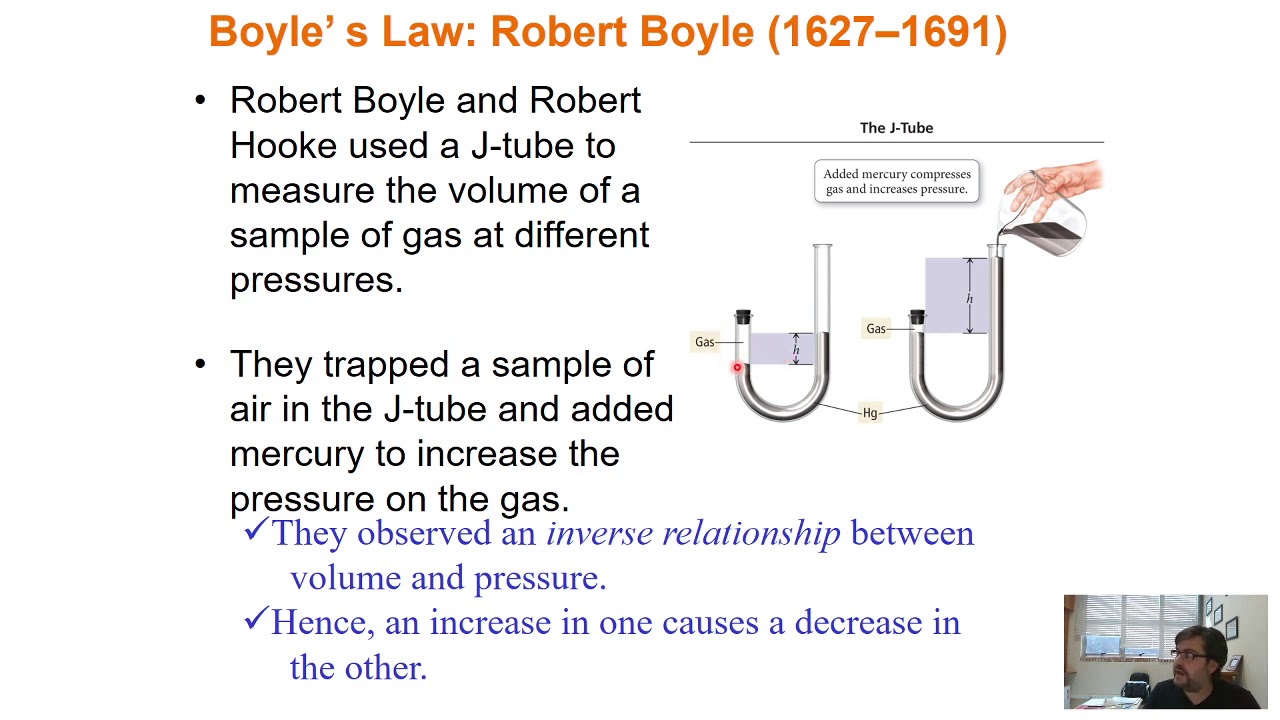
• 😀 Increasing the pressure causes the volume to decrease, and vice versa, maintaining balance as per the Ideal Gas Law.
• 😀 A balloon's volume can be increased by raising pressure in your mouth, demonstrating the relationship between pressure and volume.
• 😀 Changing the temperature of a gas, such as using liquid nitrogen or canned air, directly impacts its volume, shrinking or expanding it.
• 😀 Canned air, when sprayed upside down, rapidly cools the surrounding area, causing gases to contract and the balloon to shrink.
• 😀 Applying pressure to a gas causes its temperature to drop, as seen when the pressure inside a canister decreases during use.
• 😀 The use of canned air in experiments demonstrates how a decrease in pressure leads to a drop in temperature, resulting in rapid cooling.
• 😀 By building a small apparatus with a bottle and straw, it’s possible to observe how changing temperature impacts pressure inside a container.
• 😀 Marshmallow volume changes illustrate how pressure affects the size of objects, as the marshmallow expands when the pressure decreases.
• 😀 Increasing the pressure inside a sealed container, such as a 2-liter bottle with a valve, results in an increase in temperature as per the Ideal Gas Law.

Q & A

What is the Ideal Gas Law equation?

The Ideal Gas Law is expressed as PV = nRT (or sometimes nKT), where P is pressure, V is volume, n is the number of moles of gas, R is the gas constant, and T is temperature.

How does the volume of a balloon change when pressure is applied?

When pressure is increased in a balloon, the volume decreases, demonstrating Boyle's Law, which states that pressure and volume are inversely proportional when temperature is constant.

How does temperature affect the volume of a balloon?

When the temperature of a balloon is increased, its volume increases, and when the temperature is decreased, the volume shrinks. This is consistent with Charles' Law, where temperature and volume are directly proportional at constant pressure.

What happens when you spray compressed air upside down on a balloon?

Spraying compressed air upside down on a balloon cools it rapidly. This cooling effect causes the balloon to shrink as the temperature drops, demonstrating the relationship between pressure and temperature as outlined in Gay-Lussac's Law.

Why does a marshmallow shrink when compressed in a syringe?

When the marshmallow is compressed in a syringe, the volume decreases, causing the pressure inside the syringe to increase. This compression collapses the air pockets inside the marshmallow, causing it to shrink. When the pressure is released, the marshmallow returns to its normal size.

What is the effect of increasing pressure inside a sealed bottle?

When pressure is increased inside a sealed bottle, the temperature also rises. This is a demonstration of the direct relationship between pressure and temperature, as explained by Gay-Lussac’s Law.

What safety precautions should be taken when performing the experiments with compressed air?

Safety precautions include handling the compressed air cans carefully to avoid frostbite, using protective gloves and eyewear, and ensuring that the pressure in experimental setups like the 2-liter bottle does not exceed 60 psi to prevent dangerous explosions.

What is the purpose of using a bike pump in the final experiment?

The bike pump is used to increase the pressure inside a 2-liter bottle, demonstrating how increasing pressure results in an increase in temperature. This shows the relationship between pressure and temperature, as described by Gay-Lussac’s Law.

How does the use of liquid nitrogen help demonstrate the Ideal Gas Law?

Liquid nitrogen is used to rapidly cool a balloon, causing its volume to shrink as the temperature decreases. This helps demonstrate the relationship between temperature and volume (Charles' Law) in a tangible way.

What are the risks of using compressed air cans in the experiments?

The risks include potential frostbite from the cold mist if the can is held upside down, the possibility of inhaling harmful chemicals designed to deter huffing, and the danger of over-pressurizing containers, which could lead to explosion or injury.