Introduction to point-slope form | Algebra I | Khan Academy

Summary

The video script explains the process of deriving the equation of a line given a point on the line and its slope. It starts by defining the slope as the change in y over the change in x and uses this to establish a relationship between any point on the line and the given point. The script then simplifies the equation into the point-slope form, illustrating how to convert it into more familiar forms like slope-intercept form. The example provided uses a line with a slope of 2 passing through the point (-7, 5), demonstrating how to write the equation in both point-slope and slope-intercept forms.

Takeaways

• 📐 The script explains how to derive the equation of a line given a point on the line and its slope.
• 🟡 The yellow line in the script represents a line with a known slope 'm' and a known point 'a, b'.
• ✏️ Any point 'x, y' on the line must satisfy the condition that the slope between 'a, b' and 'x, y' equals 'm'.
• 🔍 The slope between two points is calculated as the change in y over the change in x (Δy/Δx).
• 📉 The change in y (Δy) is represented as 'y - b' and the change in x (Δx) as 'x - a'.
• ✅ The equation of the line is derived by setting the slope between 'a, b' and 'x, y' equal to 'm', resulting in the equation (y - b) / (x - a) = m.
• 🔄 Multiplying both sides of the equation by (x - a) eliminates the fraction and leads to the point-slope form of the line equation: y - b = m(x - a).
• 📚 The point-slope form of a line's equation is y - y1 = m(x - x1), where (x1, y1) is a point on the line and 'm' is the slope.
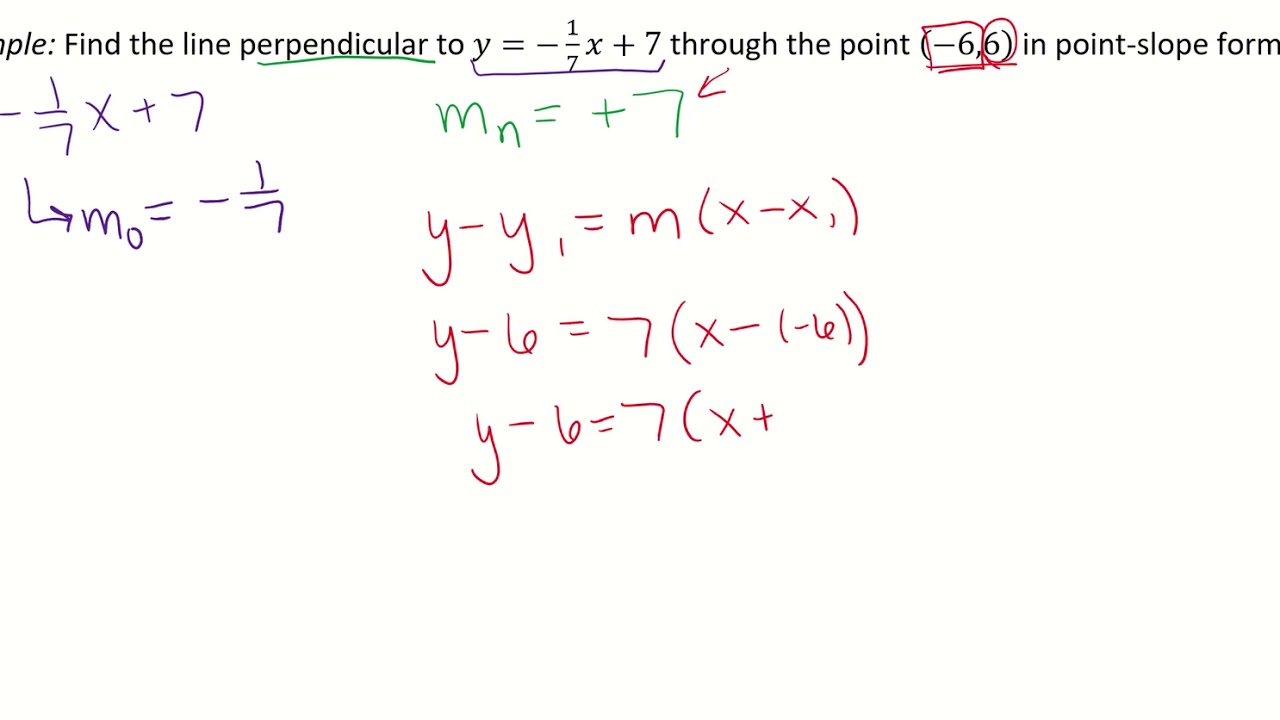
• 📈 An example is provided using a line with a slope of 2 that passes through the point (-7, 5), resulting in the equation y - 5 = 2(x + 7).
• 🔄 The point-slope form can be converted to the slope-intercept form by distributing and simplifying, resulting in y = 2x + 19.
• 📝 The slope-intercept form of a line's equation is y = mx + b, where 'm' is the slope and 'b' is the y-intercept.

Q & A

What is the purpose of the yellow line in the script?

The yellow line represents a line in a coordinate plane, which is used to illustrate how to derive the equation of a line given a point on the line and its slope.

What are the two pieces of information given about the line in the script?

The two pieces of information given are that the line has a slope of 'm' and that the point 'a, b' lies on the line.

How is the slope of a line defined in the script?

The slope of a line is defined as the change in y (Δy) over the change in x (Δx), which is the rise over run between two points on the line.

What is the equation derived from the condition that the slope between a point on the line and the point 'a, b' must be equal to 'm'?

The equation derived is \((y - b) = m(x - a)\), which is the point-slope form of the line's equation.

Why is multiplying both sides of the equation by (x - a) useful?

Multiplying both sides by (x - a) is useful because it eliminates the denominator, simplifying the equation and making it easier to work with.

What is the point-slope form of a line's equation?

The point-slope form of a line's equation is \(y - y_1 = m(x - x_1)\), where \( (x_1, y_1) \) is a point on the line and 'm' is the slope.

How can the point-slope form be used to find the equation of a line with a given slope and point?

The point-slope form can be used by substituting the given slope 'm' and the coordinates of the given point 'a, b' into the equation \((y - b) = m(x - a)\).

What is the slope-intercept form of a line's equation, and how is it derived from the point-slope form?

The slope-intercept form is \(y = mx + b\), where 'm' is the slope and 'b' is the y-intercept. It is derived from the point-slope form by distributing 'm' and then isolating 'y' on one side of the equation.

What is the significance of the 'y-intercept' in the slope-intercept form of a line's equation?

The 'y-intercept' in the slope-intercept form represents the point where the line crosses the y-axis, which is the value of 'y' when 'x' is zero.

How can the script's explanation of deriving a line's equation be applied to a real-world scenario?

The script's explanation can be applied to real-world scenarios where understanding the relationship between two variables is necessary, such as in physics, economics, or any field that involves linear regression analysis.