Irisan Kerucut - Elips • Part 11: Contoh Soal Persamaan Garis Singgung Elips
Summary
TLDRThis educational video from 'Jendela Sains' explores the mathematics of ellipses, focusing on the equations of tangent lines. It begins with finding the tangent line equation at a specific point on the ellipse \( x^2/8 + y^2/32 = 1 \), confirming the point's location on the ellipse before deriving the tangent line formula. The video then tackles a problem involving an ellipse with a given gradient, using the point-slope form to find the tangent line equations. Lastly, it solves for tangent lines parallel to a given line on a different ellipse equation, emphasizing the relationship between gradients. Throughout, the video provides step-by-step calculations and clear explanations, making complex mathematical concepts accessible.
Takeaways
- 📚 This video is an educational tutorial focused on ellipses, specifically discussing the equations of tangent lines to ellipses.
- 🔍 The video explains how to determine the equation of a tangent line to an ellipse at a given point, using the example of the ellipse equation \( \frac{x^2}{8} + \frac{y^2}{32} = 1 \) at the point (2, 4).
- 📈 The process involves verifying if the given point lies on the ellipse, which is done by substituting the point into the ellipse equation.
- 🧮 After confirming the point is on the ellipse, the equation of the tangent line is derived using the formula \( \frac{x_1x}{a^2} + \frac{y_1y}{b^2} = 1 \), where \( (x_1, y_1) \) is the given point.
- 📉 The video demonstrates the calculation by substituting the values and simplifying to get the tangent line equation \( y = -2x + 8 \).
- 📐 The tutorial also covers finding the tangent line equation when the gradient (slope) is known, using the point-slope form of a line equation.
- 📘 Another example provided is determining the tangent line equation for an ellipse with a given gradient, using the standard form of the ellipse equation and the slope.
- 🔢 The video explains how to handle ellipse equations that are not in standard form, by normalizing them to the standard form \( \frac{x^2}{a^2} + \frac{y^2}{b^2} = 1 \) before applying the tangent line formula.
- ✅ The video concludes with the solutions to the example problems, providing the equations of the tangent lines for the given ellipses.
- 💬 The presenter encourages viewers to check the playlist for more videos on the topic, and to leave comments with questions or feedback.
Q & A
What is the equation of an ellipse given in the video?
-The equation of the ellipse discussed in the video is \( \frac{x^2}{8} + \frac{y^2}{32} = 1 \).
How do you determine if a point lies on an ellipse?
-To determine if a point lies on an ellipse, you substitute the coordinates of the point into the ellipse equation and check if it satisfies the equation.
What is the equation of the tangent line to the ellipse at the point (2,4)?
-The equation of the tangent line to the ellipse at the point (2,4) is \( y = -2x + 8 \).
What is the significance of the gradient (m) in the equation of a tangent line to an ellipse?
-The gradient (m) in the equation of a tangent line to an ellipse represents the slope of the tangent line at the point of tangency.
How do you find the coordinates of the center (h,k) of an ellipse from its equation?
-The center (h,k) of an ellipse is found by looking at the terms in the ellipse equation. If the equation is in the form \( (x-h)^2/a^2 + (y-k)^2/b^2 = 1 \), then (h,k) is the center.
What is the process to find the equation of a tangent line to an ellipse with a given gradient?
-To find the equation of a tangent line to an ellipse with a given gradient, you use the formula \( y - y_1 = m(x - x_1) \), where m is the gradient, and (x_1, y_1) is a point on the ellipse.
What does it mean for a line to be tangent to an ellipse?
-A line is tangent to an ellipse if it touches the ellipse at exactly one point, indicating that the line and the ellipse have only one point of intersection.
How do you calculate the new equation of a tangent line when the gradient is given?
-When the gradient is given, you substitute the gradient and the coordinates of a point on the ellipse into the tangent line formula to calculate the new equation.
What is the role of a and b in the equation of an ellipse?
-In the equation of an ellipse \( \frac{x^2}{a^2} + \frac{y^2}{b^2} = 1 \), a and b represent the semi-major and semi-minor axes, respectively, and determine the shape of the ellipse.
How do you determine if two lines are parallel given their equations?
-Two lines are parallel if their gradients are equal. Given the equations of the lines, you can determine their gradients and compare them to check for parallelism.
What is the significance of the term 'a^2' and 'b^2' in the ellipse equation?
-In the ellipse equation, 'a^2' and 'b^2' are the denominators of the fractions associated with the x and y terms, respectively, and are related to the lengths of the semi-major and semi-minor axes of the ellipse.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Irisan Kerucut - Hiperbola • Part 16: Persamaan Garis Singgung di Suatu Titik di Luar Hiperbola
Persamaan Garis Singgung dan Garis Normal Fungsi Trigonometri - Aplikasi Turunan Fungsi Trigonometri
MATEMATIKA VIII Bab 4 Bagian 2 PERSAMAAN GARIS dengan KEMIRINGAN

Matriks Matematika Kelas 11 • Part 23: Menyelesaikan SPLTV dengan Metode Determinan Matriks
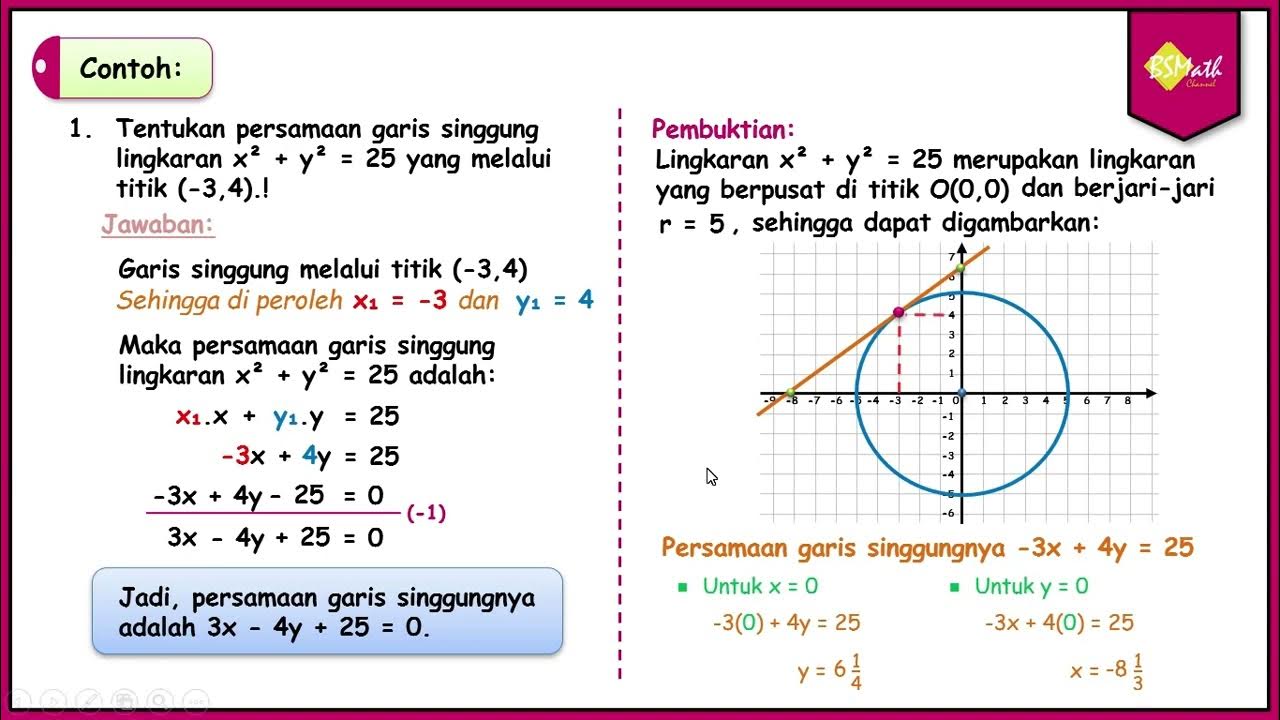
Persamaan Garis Singgung Lingkaran di Suatu Titik Pada Lingkaran - SMA Kelas XI Kurikulum Merdeka

[Math 20] Lec 1.5 Lines and Circles
5.0 / 5 (0 votes)