LINGKARAN DALAM | LINGKARAN LUAR SEGITIGA | 8 SMP.

Summary

This educational video provides an in-depth lesson on the concepts of the incircle and circumcircle of a triangle. The content covers how to find the radii of both types of circles, using various examples and problem-solving steps. The script demonstrates how the incircle touches the sides of the triangle and the circumcircle passes through its vertices. Key topics include calculating the perimeter of a triangle and solving for the radius of both circles using geometric properties and Pythagoras' theorem. The lesson is filled with practical problems to enhance the viewer's understanding of these fundamental geometric concepts.

Takeaways

• 😀 The video discusses the concept of the in-circle and circumcircle of a triangle, explaining how these circles interact with the triangle's sides and vertices.
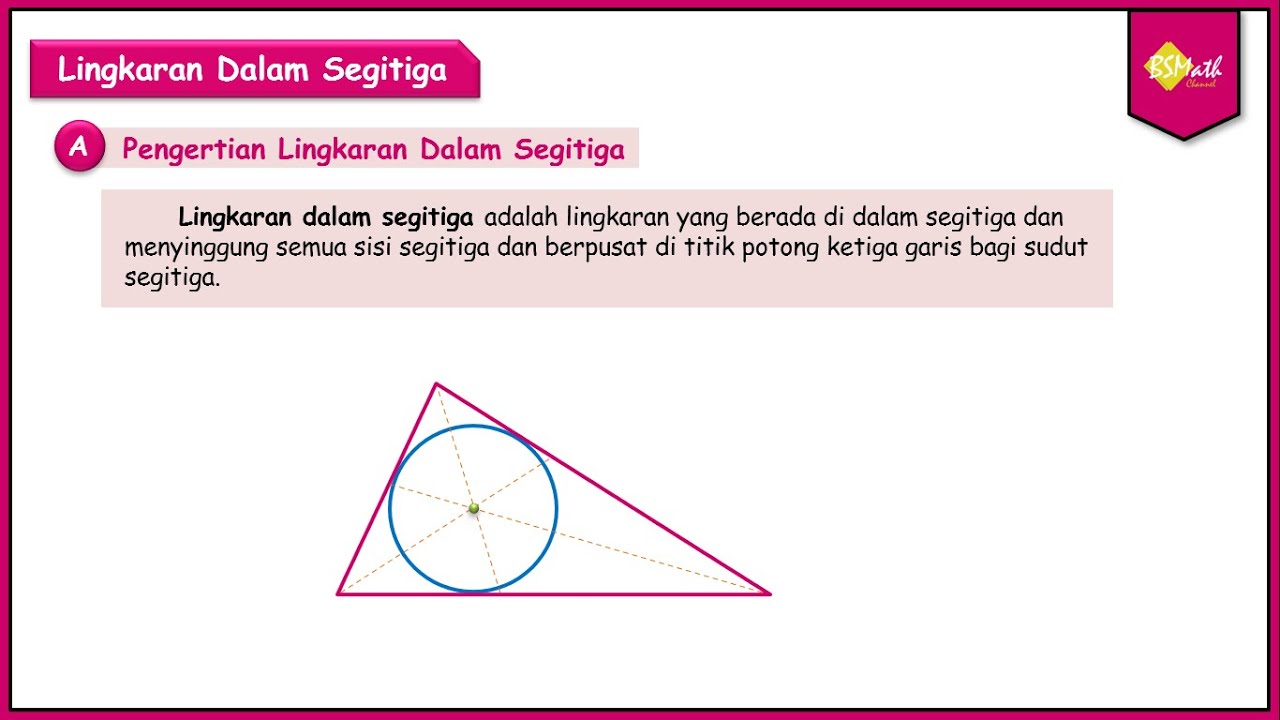
• 😀 The in-circle touches the sides of a triangle at points P, Q, and R, and these points are called 'tangent points.' The circle is referred to as the 'incircle' of the triangle.
• 😀 The circumcircle passes through the vertices of the triangle (A, B, and C) and is referred to as the 'circumcircle' of the triangle.
• 😀 The video explains that the lengths of the segments from the vertices to the tangent points are equal on both sides of the triangle.
• 😀 A practical problem is given where the lengths of various segments are provided, and the perimeter of the triangle is calculated by summing the lengths of the sides.
• 😀 Another problem is introduced involving the lengths of the sides of a triangle (AB, AC, BC), where the relationship between the tangential segments is used to solve for unknown values.
• 😀 The video uses the example of a right-angled triangle to demonstrate the calculation of the radius of the incircle and the circumcircle using geometric properties.
• 😀 The relationship between the sides of a right-angled triangle is used to calculate the radius of the incircle, with a focus on using known formulas.
• 😀 The importance of understanding the properties of the in-circle and circumcircle is emphasized for solving various geometric problems involving triangles.
• 😀 The video concludes with a motivational message encouraging viewers to practice with additional problems to strengthen their understanding and skills in geometry.

Q & A

What is an incircle in a triangle?

An incircle is a circle that is tangent to the sides of a triangle. The points where the circle touches the sides are called tangency points.

What is an excircle in a triangle?

An excircle is a circle that is tangent to one side of the triangle and the extensions of the other two sides. The circle is located outside the triangle.

What is the relationship between the lengths of the tangents from a point to a circle?

The lengths of the tangents drawn from an external point to a circle are equal. For example, in the incircle of a triangle, the tangents from the vertices to the tangency points have equal lengths.

How is the perimeter of a triangle calculated using the lengths of the tangents?

The perimeter of a triangle can be calculated by adding the lengths of the segments formed by the tangency points. For example, if the tangents from points A, B, and C are known, the perimeter is the sum of these tangent lengths.

In the given example, if AB = 10 cm, AP = 3 cm, and CQ = 6 cm, how do you calculate the perimeter of triangle ABC?

In this case, BP = 7 cm (since AB - AP = 10 - 3). The perimeter is the sum of all the tangent lengths: 3 cm + 7 cm + 7 cm + 6 cm + 6 cm + 3 cm = 32 cm.

How do you find the length of the tangent AP given AB = 13 cm, AC = 20 cm, and BC = 21 cm?

Let AP = a, PB = b, and CQ = c. From the given conditions, a + c = 20, a + b = 13, and b + c = 21. Solving these equations, we find that AP = 6 cm.

How do you calculate the radius of the incircle of a right triangle?

In a right triangle, the radius of the incircle can be calculated using the formula r = (a + b - c) / 2, where a and b are the legs of the right triangle, and c is the hypotenuse.

What is the relationship between the diameter and the radius of an excircle?

For a right triangle, the excircle's diameter is equal to the length of the hypotenuse, and its radius is half of the hypotenuse length.

How do you calculate the radius of an excircle when given AB = 10 cm, BC = 6 cm, and AC = 8 cm?

Since AB is the hypotenuse of the right triangle ABC, the radius of the excircle is half of AB. Therefore, the radius is 10 cm / 2 = 5 cm.

What is the significance of the Pythagorean theorem in the context of the problems discussed?

The Pythagorean theorem is used to confirm that a triangle is a right triangle. In the case of the excircle, the theorem helps identify the hypotenuse, which is crucial for calculating the radius of the excircle.