Rotational Form of Newton's Second Law - Introduction
Summary
TLDRIn this lesson, the focus is on the rotational form of Newton's Second Law of Motion. The discussion begins with a review of Newton's Second Law in its translational form, emphasizing net force, mass, and acceleration. The video then shifts to the rotational form, which involves net torque, moment of inertia, and angular acceleration. Key concepts include the importance of identifying the object(s), axis of rotation, and direction of positive torque when applying this law. The lesson concludes by reinforcing how net torque leads to angular acceleration, proportional to torque and inversely proportional to rotational inertia.
Takeaways
- 😀 Newton's Second Law of Motion states that net force equals mass times acceleration, with both force and acceleration being vectors.
- 😀 The translational form of Newton's Second Law involves summing all the forces acting on an object or objects.
- 😀 When applying Newton's Second Law, it is crucial to identify the object(s) and the direction of the forces.
- 😀 The rotational form of Newton's Second Law concerns net torque, which is a vector, and is equal to the moment of inertia times angular acceleration.
- 😀 Torque is the rotational counterpart to force, and moment of inertia is the rotational counterpart to mass.
- 😀 Angular acceleration is the rotational equivalent of linear acceleration in Newton's Second Law.
- 😀 Just like with net force, when using the rotational form, it's important to identify the object(s) and the axis of rotation.
- 😀 The direction of positive torque should be identified to avoid confusion, often marked with an arrow and plus sign in diagrams.
- 😀 Torque refers to the ability of a force to cause an angular acceleration in an object.
- 😀 The equation for the rotational form of Newton's Second Law shows that angular acceleration is directly proportional to net torque and inversely proportional to moment of inertia.
- 😀 The magnitude of net torque determines the magnitude of angular acceleration, assuming rotational inertia remains constant.
Q & A
What is Newton's Second Law of Motion?
-Newton's Second Law of Motion states that the net force acting on an object is equal to the object's mass times its acceleration (F = ma), where both force and acceleration are vectors.
What is the translational form of Newton's Second Law?
-The translational form of Newton's Second Law involves not just a single force but the net force, which is the sum of all forces acting on an object or objects.
What must be identified when using Newton's Second Law?
-When using Newton's Second Law, it's essential to identify the object or objects on which forces are being summed, as well as the direction of the forces.
What does the rotational form of Newton's Second Law involve?
-The rotational form of Newton's Second Law involves the net torque, which is the sum of all torques acting on an object or objects, and it is equal to the moment of inertia times angular acceleration.
What is torque and how is it related to angular acceleration?
-Torque is the ability of a force to cause an angular acceleration in an object. The larger the net torque, the greater the angular acceleration, assuming the object's rotational inertia remains constant.
What is moment of inertia?
-Moment of inertia, or rotational inertia, is the rotational equivalent of mass in linear motion. It quantifies an object's resistance to angular acceleration.
How is the rotational form of Newton's Second Law similar to the translational form?
-Both forms are similar in that they involve the relationship between a force or torque, the mass or moment of inertia, and acceleration or angular acceleration. In the rotational form, net torque equals moment of inertia times angular acceleration.
What additional factors must be identified when using the rotational form of Newton's Second Law?
-When using the rotational form of Newton's Second Law, it's important to identify the object or objects being summed for torques, the axis of rotation, and the direction of positive torque.
Why is it important to identify the direction of positive torque?
-Identifying the direction of positive torque helps avoid confusion and ensures consistency when analyzing rotational motion.
What is the relationship between angular acceleration and net torque?
-The angular acceleration of an object produced by a net torque is directly proportional to the magnitude of the net torque and in the same direction, while it is inversely proportional to the object's moment of inertia.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示
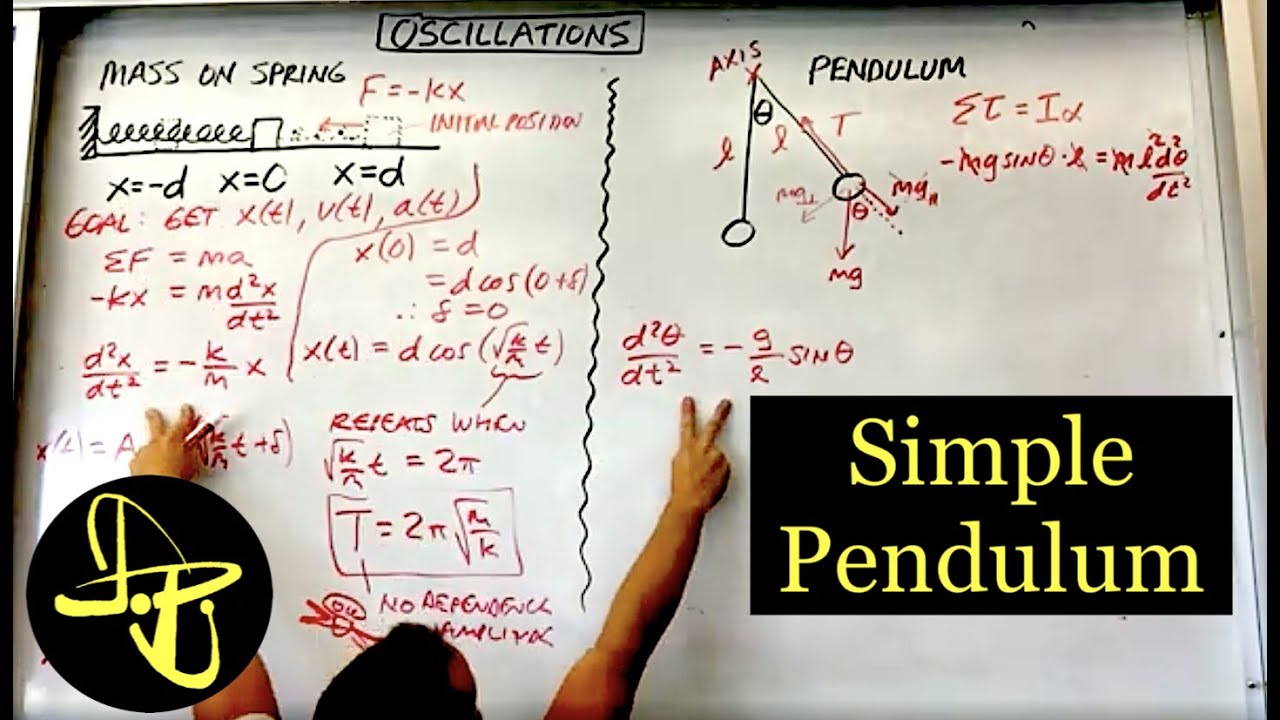
Simple Pendulum Motion Derived Using Torque and the Small Angle Approximation
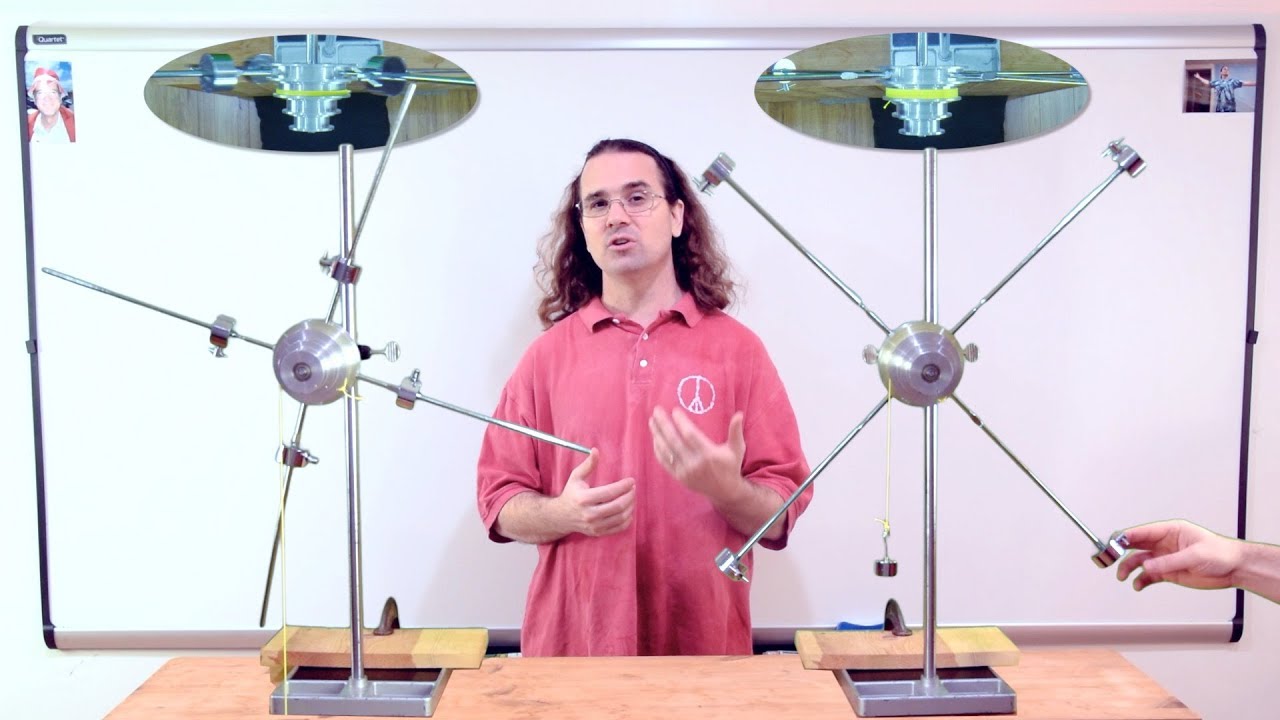
Demonstrating Rotational Inertia (or Moment of Inertia)

(1 of 2) Measuring the Rotational Inertia of a Bike Wheel

4.1 Newton's First and Second Laws

Fisika kelas 11 | Dinamika rotasi dan kesetimbangan benda tegar (part 1)

Lecture3 part2 video
5.0 / 5 (0 votes)
