Graph & illustrates Linear Function Find the Domain Range Table of values Intercepts & Slope Math 8
Summary
TLDRIn this lesson, Teacher Mel explains how to graph and analyze a linear function, focusing on its slope, intercepts, and table of values. Using the example function f(x) = -2x + 4, she demonstrates how to find the slope, x-intercept, and y-intercept. The lesson includes creating a table of values, graphing the function, and discussing its domain and range, which are both all real numbers. This video offers a comprehensive introduction to linear functions, ideal for students looking to master these key concepts in algebra.
Takeaways
- 😀 A linear function is expressed as f(x) = mx + b, where m is the slope and b is the y-intercept.
- 😀 The slope (m) represents the rate of change in a linear function and should not be equal to zero.
- 😀 The y-intercept (b) is the point where the graph of the function crosses the y-axis (x = 0).
- 😀 To find the x-intercept, set f(x) = 0 and solve for x.
- 😀 The degree of a linear function is always 1, meaning the graph is a straight line.
- 😀 The function f(x) = -2x + 4 has a slope of -2 and a y-intercept of 4.
- 😀 To find the x-intercept of f(x) = -2x + 4, set f(x) = 0 and solve for x, yielding x = 2.
- 😀 A table of values helps plot points to graph the function, providing specific values for given x-values.
- 😀 For the function f(x) = -2x + 4, when x = 0, y = 4; when x = 1, y = 2; and when x = 2, y = 0.
- 😀 The domain of a linear function is all real numbers, from negative infinity to positive infinity.
- 😀 The range of a linear function is also all real numbers, as the graph continues infinitely in both directions.
Q & A
What is a linear function?
-A linear function is a function of the form f(x) = mx + b, where m is the slope and b is the y-intercept. It represents a straight line when graphed, and the degree of the function is 1.
What do the variables m and b represent in the equation f(x) = mx + b?
-In the equation f(x) = mx + b, m represents the slope of the line, and b represents the y-intercept, which is the point where the line crosses the y-axis.
What does it mean if the slope m is not equal to zero?
-If the slope m is not equal to zero, the graph of the function will be a non-horizontal line. A slope of zero would result in a horizontal line, but here, the slope is -2, so the graph will be a declining line.
How do you find the slope of the line in the function f(x) = -2x + 4?
-In the function f(x) = -2x + 4, the slope is represented by m, which is -2. This means that for every 1 unit increase in x, the value of f(x) (or y) decreases by 2 units.
How do you find the y-intercept from the equation f(x) = -2x + 4?
-The y-intercept is the point where the line crosses the y-axis, which occurs when x = 0. Substituting x = 0 into the equation gives f(0) = 4, so the y-intercept is at (0, 4).
What is the process for finding the x-intercept?
-To find the x-intercept, set f(x) equal to 0 and solve for x. For the function f(x) = -2x + 4, setting 0 = -2x + 4 and solving gives x = 2. Therefore, the x-intercept is at (2, 0).
What does a table of values represent in graphing a linear function?
-A table of values shows the corresponding y-values for a given set of x-values. These points are plotted on the graph to visualize the linear relationship between x and y.
What points are used in the table of values for the function f(x) = -2x + 4?
-The points chosen for the table of values are x = 0, 1, 2, 3, and 4. For each x-value, the corresponding y-value is calculated using the equation f(x) = -2x + 4.
How do you graph the function f(x) = -2x + 4?
-To graph the function, plot the points from the table of values (e.g., (0, 4), (1, 2), (2, 0), etc.) and then connect them with a straight line. The graph will be a straight line that slopes downward due to the negative slope of -2.
What is the domain and range of the function f(x) = -2x + 4?
-The domain and range of a linear function are both all real numbers, as the graph extends infinitely in both directions along the x-axis and y-axis. Thus, the domain and range are both (-∞, ∞).
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Função do 1º Grau (Função Afim) | Matemática do Zero | Funções - Aula 01

Write and graph a linear function by examining a real-life scenario
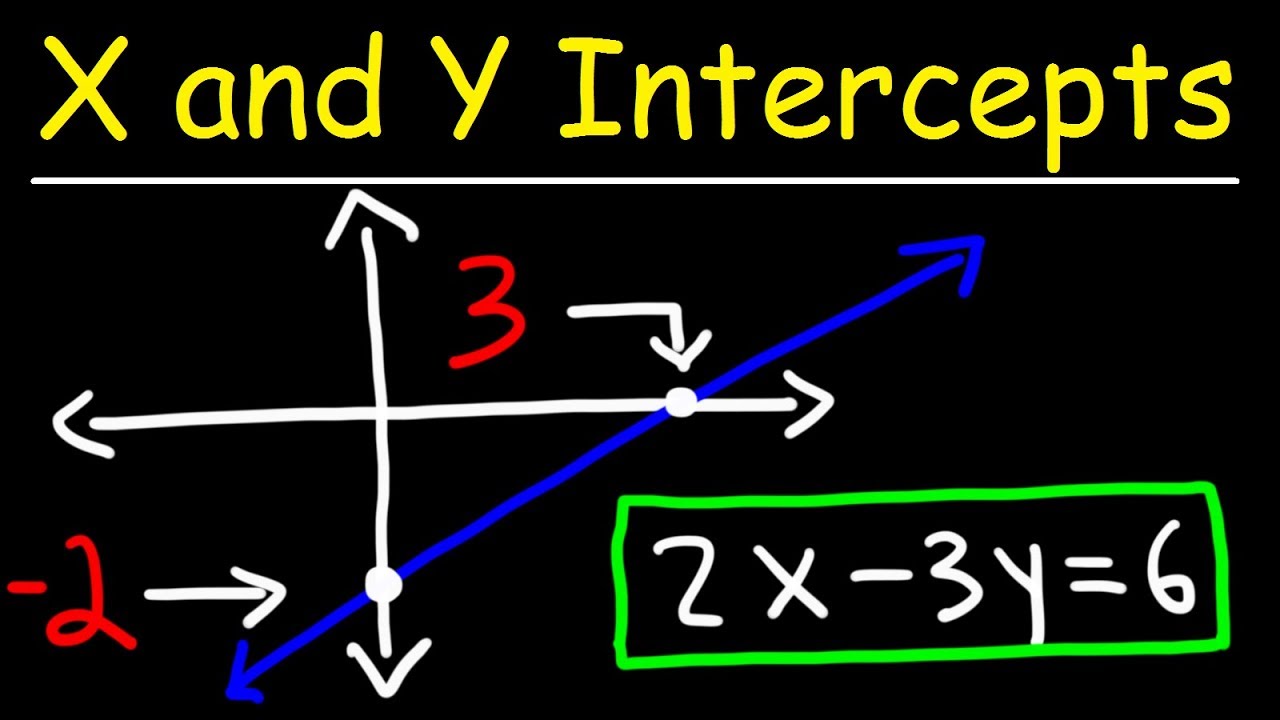
How To Find The X and Y Intercepts of a Line

Taxa de Variação da Função Afim
ILLUSTRATING A LINEAR FUNCTION || GRADE 8 MATHEMATICS Q2

Graph of Linear Equations Using Slope Intercept (Part 1)
5.0 / 5 (0 votes)
