Simple Linear Regression in R | R Tutorial 5.1 | MarinStatsLectures
Summary
TLDRIn this educational video, Mike Marin introduces 'simple linear regression' using R programming language. He demonstrates how to model the relationship between age and lung capacity with lung capacity as the dependent variable. The video covers creating a scatter plot, calculating Pearson's correlation, and fitting a linear regression model with the 'lm' command. It also explains interpreting the model summary, extracting coefficients, adding regression lines to plots, and generating confidence intervals. The tutorial concludes with a preview of regression diagnostic plots for the next video.
Takeaways
- 📚 The video introduces 'simple linear regression' using R, a statistical method for modeling the relationship between two numeric variables.
- 📈 Simple linear regression can be applied even when the explanatory variable is categorical, but this is discussed in a later video.
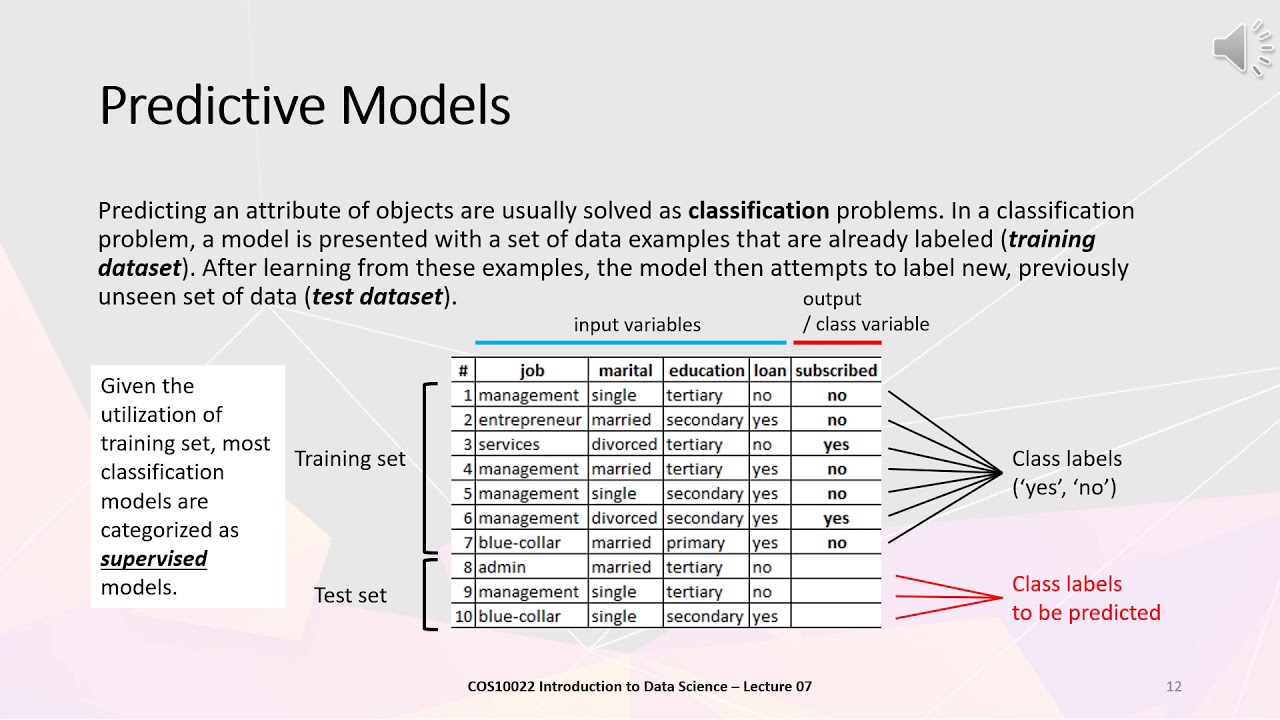
- 🗂️ The video uses lung capacity data, focusing on the relationship between 'Age' and 'Lung Capacity', with 'Lung Capacity' as the dependent variable.
- 📊 A scatter plot is created to visualize the relationship, with 'Age' on the x-axis and 'Lung Capacity' on the y-axis.
- 🔍 Pearson's correlation is calculated to assess the linear association between 'Age' and 'Lung Capacity', showing a positive correlation.
- 📝 The 'lm' command in R is used to fit a linear regression model, with the formula structured as Y ~ X.
- 🔍 The summary of the model provides insights into residuals, intercept, slope, and their statistical significance.
- 📊 The 'abline' function in R adds a regression line to the scatter plot, allowing for visual interpretation of the model fit.
- 📊 The 'coef' command is used to extract model coefficients, which are crucial for understanding the model's parameters.
- 📐 The 'confint' command provides confidence intervals for the model coefficients, indicating the precision of the estimates.
- 📊 The 'anova' command generates an ANOVA table, offering a statistical test for the overall model fit.
- 🔍 The video concludes with a mention of regression diagnostic plots to be discussed in the next video, focusing on regression assumptions.
Q & A
What is the main topic of the video presented by Mike Marin?
-The main topic of the video is introducing 'simple linear regression' using R programming language.
What is the purpose of simple linear regression in data analysis?
-Simple linear regression is used to examine or model the relationship between two numeric variables.
Can simple linear regression be applied using a categorical explanatory variable?
-While it is possible to fit a simple linear regression using a categorical explanatory variable, the video mentions that this topic will be covered in a later video.
What data set is used in the video to demonstrate simple linear regression?
-The lung capacity data set is used to demonstrate the relationship between age and lung capacity in the video.
Which variable is considered the outcome or dependent variable in the lung capacity example?
-In the lung capacity example, Lung Capacity is considered the outcome or dependent variable.
How is a scatter plot created in the video to visualize the data?
-A scatter plot is created by plotting Age on the x-axis and Lung Capacity on the y-axis, with a title added for clarity.
What statistical measure is used to quantify the linear association between Age and Lung Capacity?
-Pearson's correlation is used to quantify the linear association between Age and Lung Capacity.
How is a linear regression model fitted in R, as demonstrated in the video?
-A linear regression model is fitted in R using the 'lm' command, with the dependent variable entered first, followed by the independent variable.
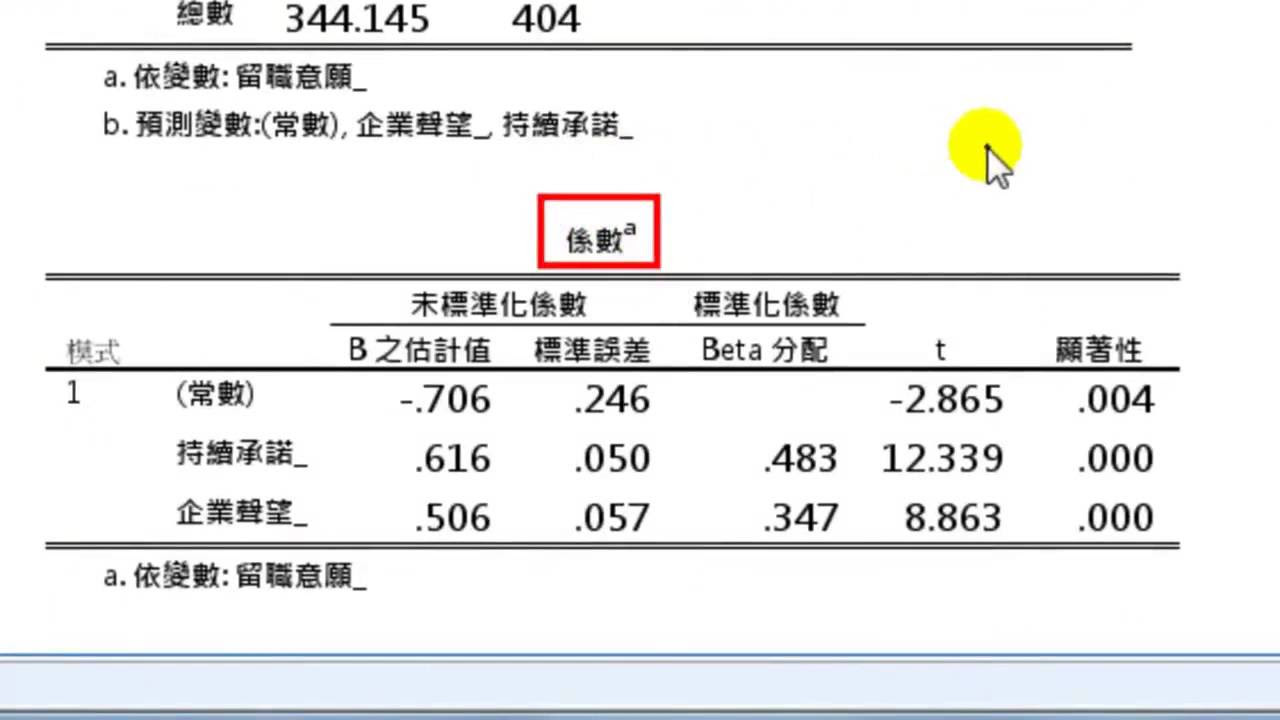
What does the summary output of a linear regression model in R provide?
-The summary output provides information about the residuals, estimates of the intercept and slope, their standard errors, test statistics, p-values, residual standard error, r-squared, and adjusted r-squared, among other things.
How can the coefficients of the regression model be extracted in R?
-The coefficients of the regression model can be extracted using the 'coef' function or by using the dollar sign ($) followed by the attribute name 'mod$coefficients'.
What command is used in R to add a regression line to a plot?
-The 'abline' command is used in R to add a regression line to a plot, with options to customize color and line width.
How can confidence intervals for the model coefficients be produced in R?
-Confidence intervals for the model coefficients can be produced using the 'confint' command, with the 'level' argument specifying the confidence level.
What does the 'anova' command in R generate for a linear regression model?
-The 'anova' command in R generates an ANOVA (Analysis of Variance) table for the linear regression model, which includes the F-test and associated p-value.
How are regression diagnostic plots mentioned in the video related to the assumptions of regression?
-Regression diagnostic plots, such as residual plots and QQ plots, are used to examine the assumptions of regression, such as linearity, homoscedasticity, and normality of residuals.
What will be the focus of the next video in the series according to the transcript?
-The next video in the series will discuss how to produce regression diagnostic plots to examine the regression assumptions.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenant5.0 / 5 (0 votes)