matematika kelas 9 | Refleksi/Pencerminan terhadap sumbu-Y
Summary
TLDRIn this video, the concept of reflection over the y-axis is explained. The host demonstrates how to reflect a point, using coordinates such as (3,1) and (-2,2), across the y-axis. By visualizing these points on a graph, viewers learn that the x-coordinate changes sign while the y-coordinate remains the same. The video emphasizes the importance of symmetry in reflections, ensuring that the distance from the point to the y-axis is equal to the distance from the reflected point to the y-axis. The host encourages viewers to subscribe for more educational content.
Takeaways
- 😀 Reflection across the y-axis involves mirroring a point across the vertical line at x = 0, known as the y-axis.
- 😀 The distance from the original point to the y-axis is the same as the distance from the reflected point to the y-axis.
- 😀 The reflection of a point (x, y) across the y-axis results in a new point (-x, y).
- 😀 In the example with point A (3, 1), its reflection across the y-axis is at (-3, 1).
- 😀 For the point B (-2, 2), after reflection across the y-axis, the new point is (2, 2).
- 😀 The rule for reflection is simple: the y-coordinate stays the same, while the x-coordinate changes sign.
- 😀 A reflection creates a mirror image, meaning both the original and reflected points are equidistant from the y-axis.
- 😀 The formula for reflecting a point (x, y) across the y-axis is A' = (-x, y).
- 😀 The y-axis acts like a mirror, flipping points to the opposite side of the axis.
- 😀 The concept of reflection across the y-axis is foundational in geometry and often used to understand symmetry.
Q & A
What is the main topic of this video?
-The video primarily discusses reflection, specifically reflection over the Y-axis.
What is the significance of reflecting over the Y-axis?
-Reflecting over the Y-axis involves mirroring a point across the Y-axis, where the X-coordinate of the point changes its sign, while the Y-coordinate remains the same.
What are the coordinates of point A before the reflection?
-Point A has coordinates (3, 1) before the reflection.
How do you reflect point A over the Y-axis?
-To reflect point A (3, 1) over the Y-axis, you negate the X-coordinate. The reflection of point A will be at (-3, 1).
What is the formula for reflecting a point over the Y-axis?
-The formula for reflecting a point (x, y) over the Y-axis is (-x, y), where the X-coordinate changes sign and the Y-coordinate remains the same.
How does the distance to the Y-axis affect the reflection?
-The distance from the original point to the Y-axis must be the same as the distance from the reflection to the Y-axis. This ensures that the reflection is symmetrical.
What are the coordinates of point B before the reflection?
-Point B has coordinates (-2, 2) before the reflection.
What happens to the coordinates of point B after the reflection over the Y-axis?
-After reflecting point B (-2, 2) over the Y-axis, the X-coordinate changes sign, and the reflection of point B will be at (2, 2).
What is the rule for reflecting any point (x, y) over the Y-axis?
-The rule for reflecting a point (x, y) over the Y-axis is to negate the X-coordinate while keeping the Y-coordinate unchanged, resulting in the point (-x, y).
What should viewers do if they haven't subscribed to the channel?
-Viewers are encouraged to subscribe to the channel to receive more content like this.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

TRANSFORMASI PART 1 : REFLEKSI DAN TRANSLASI : MATEMATIKA KELAS 9

matematika kelas 9 | Transformasi - Refleksi atau pencerminan terhadap sumbu X
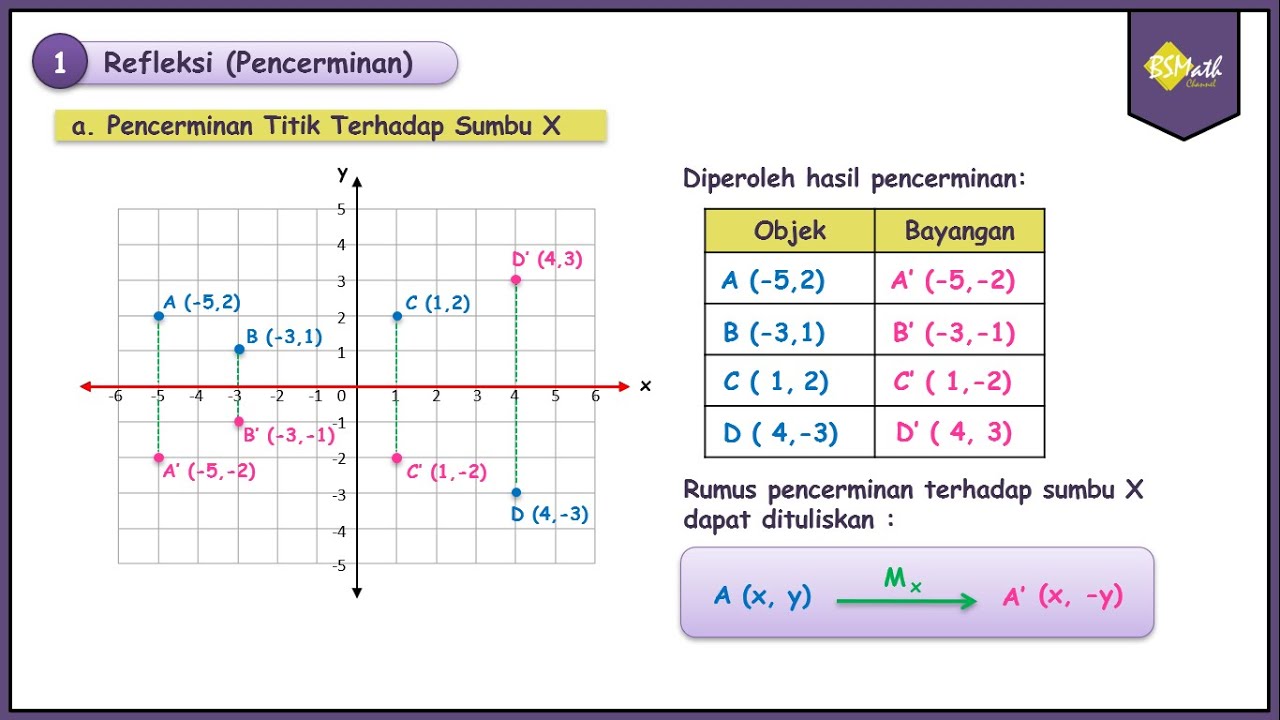
Transformasi Refleksi, Pencerminan Titik Terhadap Sumbu X - Matematika Tingkat Lanjut Kelas XI

Pencerminan/Refleksi 1| Transformasi Geometri
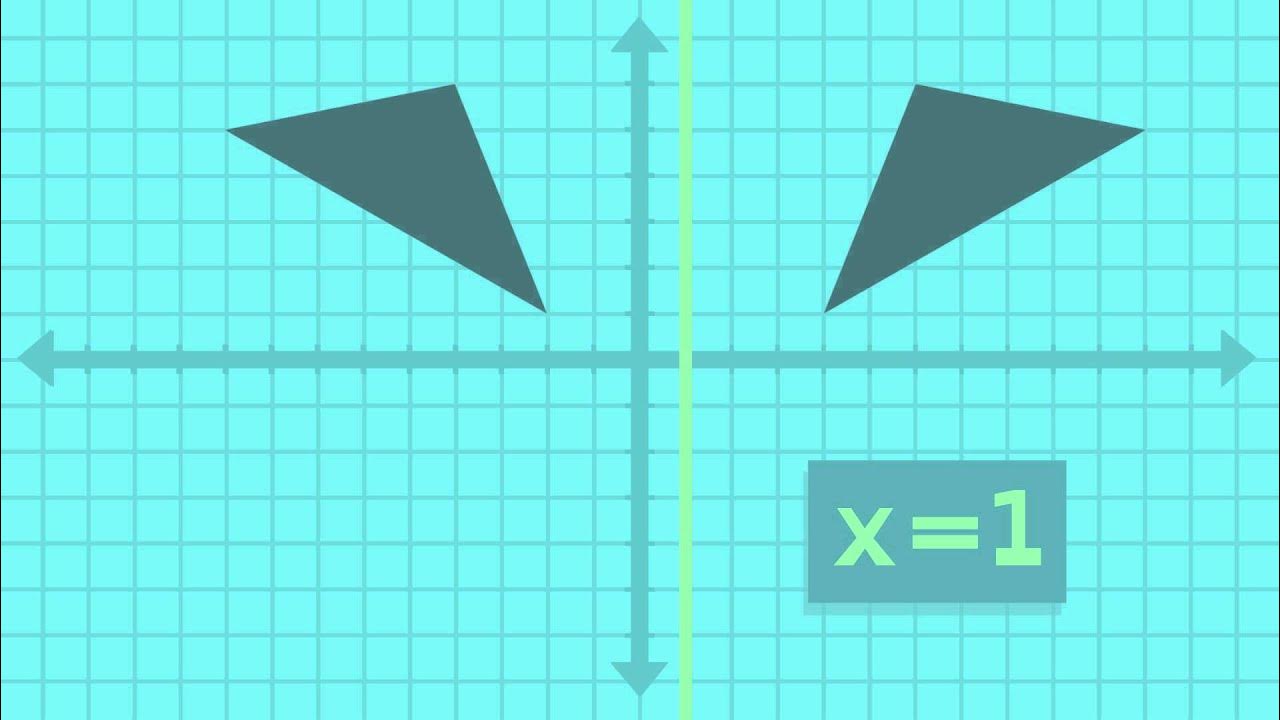
Math Shorts Episode 4 - Reflection

TRANSFORMASI GEOMETRI || Matematika Kelas XI SMA
5.0 / 5 (0 votes)
