Kernel 1
Summary
TLDRThis video script focuses on the concept of homomorphisms in group theory, specifically discussing how to determine the kernel of a homomorphism. The lecturer explains the definitions, examples, and theorems associated with homomorphisms and kernels, such as the kernel being the set of elements mapped to the identity of the codomain. Several examples are provided, including the kernel of a homomorphism between rational numbers with addition and real numbers with multiplication. The script also covers theorems related to homomorphisms, such as the identity element and inverse mapping properties.
Takeaways
- 😀 The topic of the lecture focuses on the kernel of a group homomorphism.
- 😀 The kernel of a homomorphism is defined as the set of elements in the domain that map to the identity element of the codomain.
- 😀 The kernel can contain multiple elements, including the possibility of being a singleton or even finite, depending on the homomorphism.
- 😀 An example demonstrates the kernel of a homomorphism where the set of rational numbers with addition is mapped to itself. The kernel in this case is {0}.
- 😀 The identity element for the codomain must be determined before finding the kernel, and it plays a critical role in the definition of the kernel.
- 😀 A second example involves a homomorphism from the group of positive real numbers under multiplication to the group of real numbers under addition, with the mapping defined by taking the logarithm of x.
- 😀 In the second example, the kernel of the homomorphism is {1}, as the only real number whose logarithm equals 0 is 1.
- 😀 The lecture also covers two important theorems related to homomorphisms: one about the identity element and one about the inverse of elements.
- 😀 The first theorem proves that a homomorphism maps the identity element of the domain to the identity element of the codomain.
- 😀 The second theorem asserts that the homomorphism maps the inverse of an element in the domain to the inverse of its image in the codomain.
Q & A
What is the definition of a kernel in group theory?
-In group theory, the kernel of a homomorphism is the set of all elements in the domain of the homomorphism that map to the identity element of the codomain. It is denoted as 'ker(f)' and is defined as the set {x ∈ G | f(x) = e}, where 'e' is the identity element in the codomain.
How can the kernel of a homomorphism be more than one element?
-The kernel of a homomorphism can consist of multiple elements if there are multiple elements in the domain that map to the identity element of the codomain. The kernel can also be finite, infinite, or even the trivial group containing only the identity element.
What is the identity element of the codomain in the given example with rational numbers?
-In the given example with rational numbers, the codomain is the group of rational numbers under addition, and the identity element is 0 because adding 0 to any rational number does not change the value of the number.
Why is the kernel of the homomorphism from the rational numbers to itself in the given example {0}?
-In this example, the homomorphism maps each rational number 'x' to 'x/2'. The kernel consists of elements 'x' such that 'x/2 = 0', which leads to 'x = 0'. Therefore, the kernel is the set containing only the element {0}.
What is the identity element in the codomain of the second example with positive real numbers?
-In the second example, the codomain is the group of real numbers under addition. The identity element in this group is 0, because adding 0 to any real number does not change the value of the number.
What is the kernel of the homomorphism from positive real numbers to real numbers in the second example?
-In the second example, the homomorphism maps each positive real number 'x' to its logarithm (log(x)). The kernel consists of elements 'x' such that 'log(x) = 0'. The only value of 'x' that satisfies this condition is 1, since log(1) = 0. Thus, the kernel is {1}.
What does the first theorem in the script state about homomorphisms?
-The first theorem states that if 'f' is a homomorphism from a group 'G' to a group 'G'' and 'e' is the identity element of 'G', then the image of the identity element of 'G' under the homomorphism must be the identity element of 'G''.
How does the proof of the first theorem establish the relationship between identity elements?
-In the proof, the homomorphism maps the identity element 'e' of 'G' to an element 'f(e)' in 'G'' which must satisfy 'f(e) = e'' because homomorphisms preserve the structure of groups. This shows that the identity element in the domain maps to the identity element in the codomain.
What does the second theorem in the script state about inverses under homomorphisms?
-The second theorem states that if 'f' is a homomorphism from a group 'G' to a group 'G'', then the image of the inverse of an element 'x' in 'G' under the homomorphism is the inverse of the image of 'x' in 'G''.
How does the proof of the second theorem demonstrate the relationship between inverses?
-In the proof, it is shown that if 'f' is a homomorphism and 'x' has an inverse 'x⁻¹' in 'G', then 'f(x⁻¹) = f(x)⁻¹'. This is because the homomorphism preserves the group operation, so the inverse in the codomain corresponds to the inverse of the image of 'x'.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
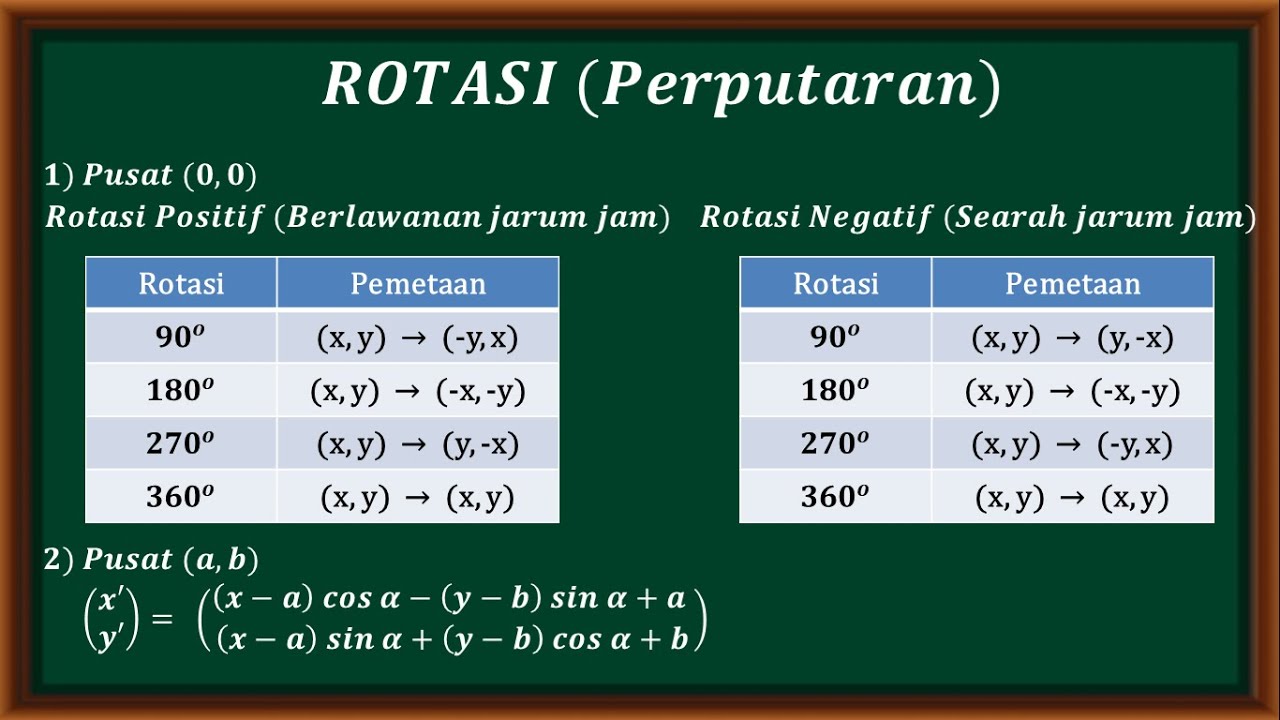
ROTASI (Perputaran) - Cara menentukan bayangan titik di pusat (0,0) dan (a,b)

PEMBANGUNAN DAN PERTUMBUHAN WILAYAH (GEOGRAFI XII)

Matematika Kelas 8 Bab 6 Statisika - Modus - Hal. 259 - 263 - Kurikulum Merdeka

Microteaching (Materi Keanekaragaman Hayati SMA Kelas X)
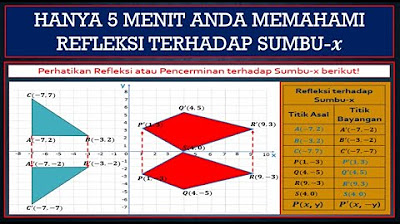
Hanya 5 menit anda paham Refleksi terhadap sumbu-𝒙

Transformasi gabungan
5.0 / 5 (0 votes)
