Point Slope Form - Basic Introduction - Algebra
Summary
TLDRIn this video, the concept of point-slope form of linear equations is explored, detailing its formula and applications. The presenter explains how to identify the slope and a point from an equation in point-slope form, along with step-by-step examples. Viewers learn how to write linear equations using given slopes and points, as well as how to graph them effectively. The video concludes with a derivation of the point-slope formula from the standard slope formula, enhancing understanding of its origins and utility in graphing linear functions.
Takeaways
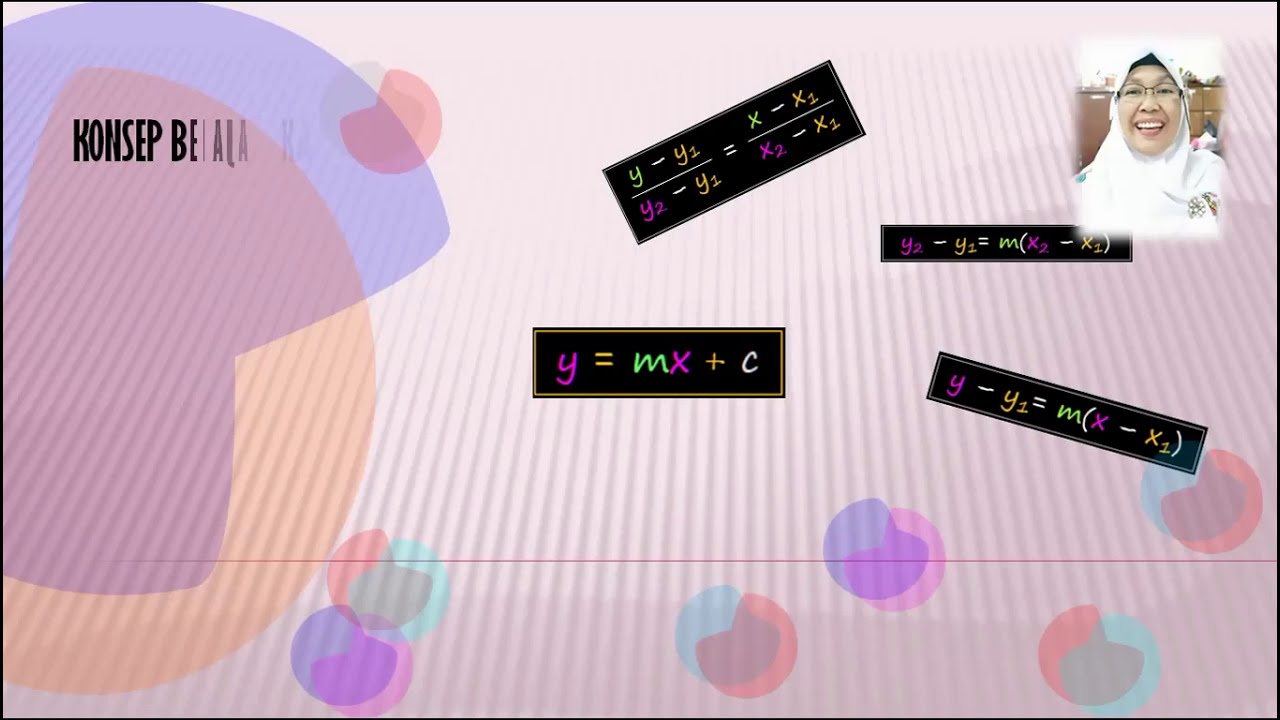
- 😀 The point-slope form of a linear equation is expressed as y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line.
- 😀 Linear equations can be represented in different forms, including standard form, slope-intercept form, and point-slope form.
- 😀 To write a linear equation in point-slope form, you need the slope and a point on the line.
- 😀 When extracting the slope and point from an equation in point-slope form, the slope is the coefficient in front of the parentheses.
- 😀 The signs of x1 and y1 in the point-slope equation are reversed compared to their appearance in the equation.
- 😀 To find the slope between two points, use the formula m = (y2 - y1) / (x2 - x1).
- 😀 When given a linear equation in point-slope form, you can identify the slope and convert the equation to standard or slope-intercept form if needed.
- 😀 Graphing a linear equation in point-slope form involves plotting a point and using the slope to find another point.
- 😀 The derivation of the point-slope formula stems from the slope formula and involves algebraic manipulation to arrive at the equation.
- 😀 Understanding point-slope form is essential for graphing linear equations and for connecting various forms of linear representation.
Q & A
What is the point-slope form of a linear equation?
-The point-slope form of a linear equation is expressed as y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line.
How do you identify the slope from a point-slope equation?
-The slope can be identified as the coefficient of x in the equation. For example, in the equation y - 2 = 3(x - 4), the slope is m = 3.
How can you find the point (x1, y1) from a point-slope equation?
-The point (x1, y1) can be determined by taking the opposite of the values next to x and y in the equation. For example, in y - 2 = 3(x - 4), x1 = 4 and y1 = 2.
What do you do if the equation includes negative signs?
-If the equation includes negative signs, you reverse the signs to find x1 and y1. For instance, in y - 2 = -4(x + 5), x1 becomes -5 and y1 becomes 2.
How do you write a point-slope equation from a given slope and point?
-To write a point-slope equation, substitute the slope (m) and the point (x1, y1) into the formula. For example, if the slope is -2 and the point is (-4, 5), the equation is y - 5 = -2(x + 4).
What formula do you use to find the slope when given two points?
-The slope can be found using the formula m = (y2 - y1) / (x2 - x1).
What steps should be taken to graph a linear equation in point-slope form?
-To graph a linear equation in point-slope form, first identify the slope and point, plot the point on the graph, use the slope to find a second point, and finally draw a line connecting the two points.
What is the significance of the point-slope formula in algebra?
-The point-slope formula is significant because it provides a method for writing and manipulating linear equations, making it easier to understand relationships between variables.
Can you derive the point-slope formula from the slope formula?
-Yes, the point-slope formula can be derived from the slope formula by rearranging the slope equation (m = (y2 - y1) / (x2 - x1)) into the form y - y1 = m(x - x1).
What is an example of using two points to write a point-slope equation?
-For points (2, 6) and (5, -3), calculate the slope as m = (-3 - 6) / (5 - 2) = -3. Using point (2, 6), the point-slope equation is y - 6 = -3(x - 2).
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

2. PENGERTIAN GRADIEN - GRADIEN MELALUI 1 TITIK, 2 TITIK DAN DARI PERSAMAAN GARIS - PGL-KELAS 8 SMP

Standard Form - Lesson 6.3
MATEMATIKA VIII Bab 4 Bagian 2 PERSAMAAN GARIS dengan KEMIRINGAN

Linear Functions

Grade 8 Lesson 2.2: Point Slope Form Short Video
Writing Equations of Parallel and Perpendicular Lines
5.0 / 5 (0 votes)
