Test for Goodness of Fit - Problem 1 - Chi Square Test - Engineering Mathematics 4
Summary
TLDRIn this informative video, the instructor explores the chi-square test for goodness of fit, illustrating how to assess whether breakdowns in a factory are uniformly distributed over the days of the week. The video details the formulation of null and alternative hypotheses, the calculation of expected frequencies, and the application of the chi-square formula to derive results. Ultimately, it concludes that the breakdowns are uniformly distributed, providing viewers with a clear understanding of the chi-square test process and its practical applications.
Takeaways
- 😀 The chi-square test is used to determine goodness of fit and independence of attributes.
- 😀 There are two types of chi-square tests: goodness of fit and test for independence.
- 😀 The problem presented involves analyzing breakdowns in a factory over a week.
- 😀 The null hypothesis assumes that the breakdowns are uniformly distributed across the week.
- 😀 The alternate hypothesis states that the breakdowns are not uniformly distributed.
- 😀 The level of significance (LOH) can be assumed to be 5% if not explicitly mentioned.
- 😀 Degrees of freedom for the goodness of fit test is calculated as n - 1, where n is the number of categories (days).
- 😀 The expected frequency for uniformly distributed breakdowns is calculated as the average of observed values.
- 😀 The formula for chi-square involves summing the squared differences between observed and expected frequencies divided by the expected frequencies.
- 😀 The final chi-square value is compared to chi-square alpha to accept or reject the null hypothesis.
Q & A
What is the primary purpose of the chi-square test discussed in the video?
-The primary purpose of the chi-square test discussed in the video is to determine if the breakdowns in a factory are uniformly distributed across different days of the week.
What are the two types of chi-square tests mentioned?
-The two types of chi-square tests mentioned are the test for goodness of fit and the test for independence of attributes.
How is the null hypothesis defined in this example?
-In this example, the null hypothesis states that the breakdowns are uniformly distributed across the days of the week.
What is the alternative hypothesis in this context?
-The alternative hypothesis states that the breakdowns are not uniformly distributed across the days of the week.
How is the level of significance determined in this video?
-The level of significance is assumed to be 5%, as it is not explicitly mentioned in the problem.
What formula is used to calculate the degrees of freedom in this test?
-The formula for degrees of freedom in this test is n - 1, where n is the number of categories or days of the week.
What are the observed frequencies given in the example?
-The observed frequencies given in the example are 14, 22, 16, 18, 12, 19, and 11 for Monday through Sunday, respectively.
How is the expected frequency calculated in this scenario?
-The expected frequency is calculated by taking the average of the observed breakdowns, which is determined to be 16 in this case, assuming uniform distribution.
What is the formula used to calculate the chi-square statistic?
-The formula for calculating the chi-square statistic is the summation of (observed - expected) squared divided by expected, represented as Σ((O - E)² / E).
What conclusion is drawn from the chi-square test in this example?
-The conclusion drawn is that the breakdowns are uniformly distributed, as the calculated chi-square value is less than the critical value from the chi-square table.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
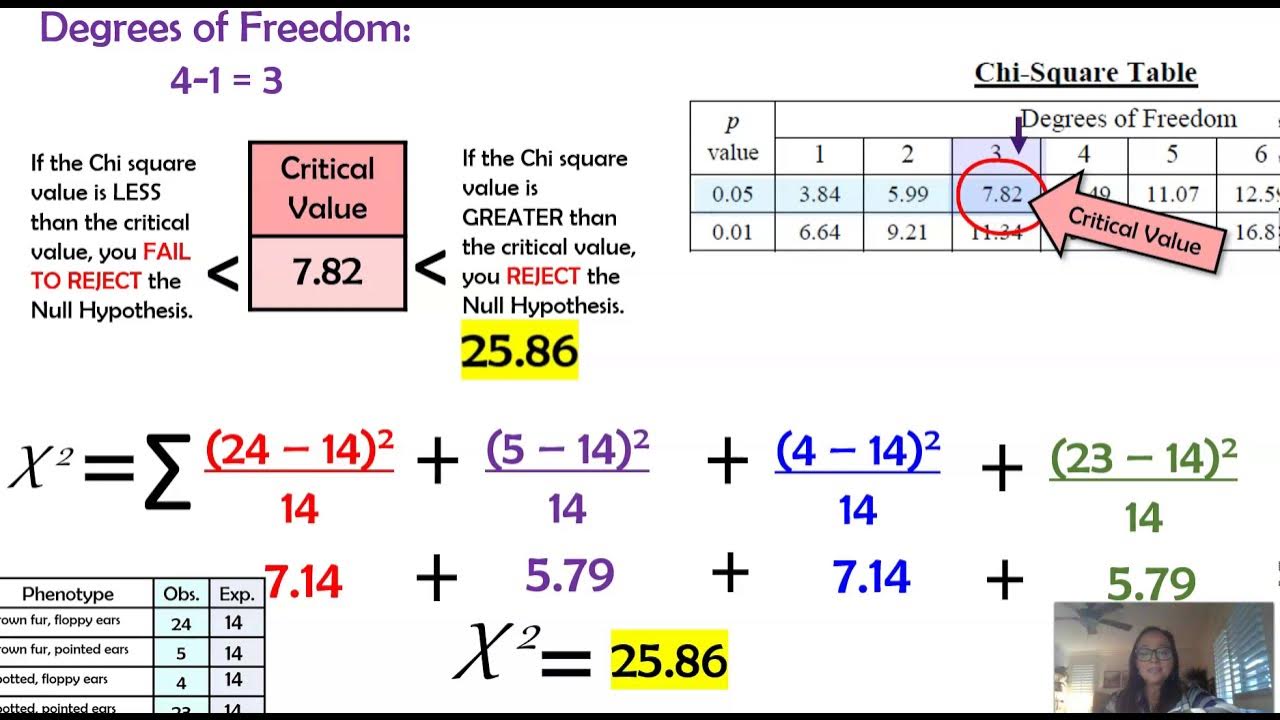
Chi Square in Genetics & Examples (AP Biology)
Chi-Square Tests: Crash Course Statistics #29
video 14.2. chi-square test of independence

*M* Uji Kecocokan: Frekuensi yang Diduga Sama dan yang Tidak Sama dengan Microsoft Excel dan SPSS

Uji Chi Square (Part 1) | Genetika Tanaman
#4 Uji Normalitas Chi Square dengan Excel - Statistika Pendidikan
5.0 / 5 (0 votes)
