Composition of Function by Ma'am Ella Barrun

Summary

This video script introduces the concept of function composition, explaining it as substituting one function into another to create a new function. It demonstrates this with several examples, showing step-by-step calculations for composite functions such as F(G(x)) and G(F(x)). The script covers linear and quadratic functions, illustrating the process of finding composite functions and their simplified forms, including evaluating composite functions at specific points.

Takeaways

• 📚 The concept of function composition is introduced, where one function is substituted into another to create a new function.
• 🔢 The notation for function composition is explained, using f(g(x)) to represent the composition of function f with function g.
• 📝 An example is provided to illustrate the process of function composition, starting with simple linear functions and moving to more complex polynomials.
• 📉 The first example demonstrates the composition of two linear functions, resulting in a new linear function.
• 📚 The second example involves composing a linear function with a quadratic function, resulting in a new quadratic function.
• 🔑 The third example shows the composition of a quadratic function with another quadratic function, leading to a new quadratic expression.
• 📌 The fourth example introduces the concept of evaluating a composite function at a specific value, in this case, G(-4).
• 🧩 The process of substituting the result of one function into another is shown step by step, with simplification of the resulting expression.
• 🔍 An example of finding the square root of a composite function's result is given, highlighting the application of real-world operations on function compositions.
• 📈 The final example demonstrates the composition of a quadratic function with a linear function, resulting in a new quadratic expression when evaluated at a specific point.
• 🤔 The script concludes with an invitation for questions, indicating an interactive component to the lesson on function composition.

Q & A

What is the definition of function composition?

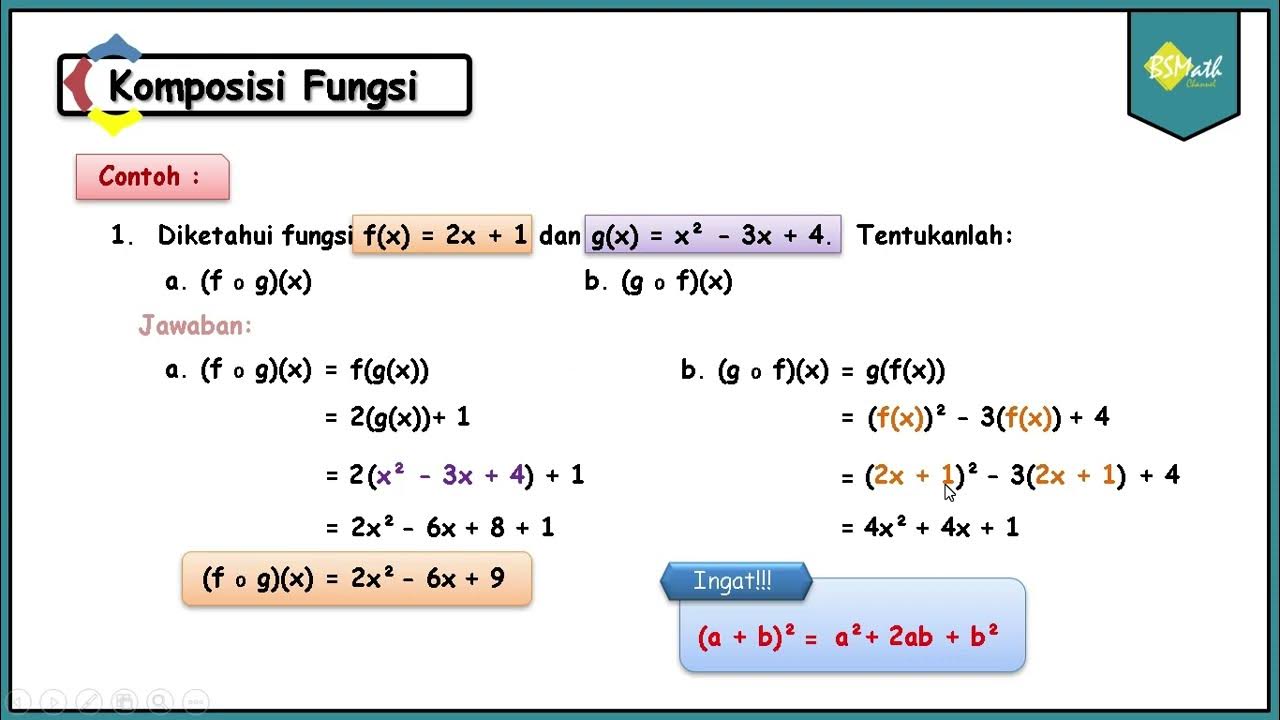
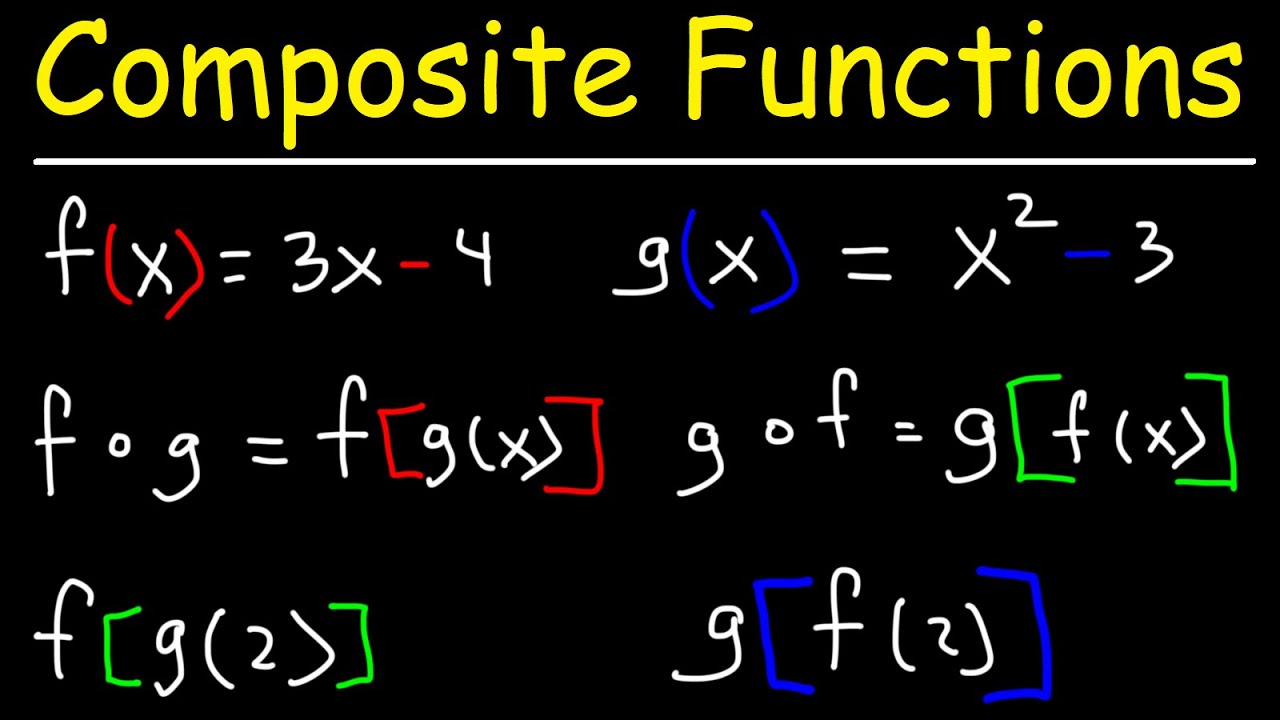
Function composition is the process of substituting one function into another, creating a new function. If a function 'f' is substituted into all variables of another function 'g', it is denoted as 'f composite with g' or 'f(g(x))'.

How is the composition of functions denoted mathematically?

The composition of functions is denoted as 'f ∘ g' or 'f(g(x))', where 'f' and 'g' are functions and 'x' is the variable.

What is the first example of function composition given in the script?

The first example given is G(x) = 5x + 3 and F(x) = 2x + 1. The composition of F with G, denoted as F(G(x)), is calculated as 2*(5x + 3) + 1, which simplifies to 10x + 7.

In the second example, what are the functions F(x) and G(x), and what is their composition?

In the second example, G(x) is given as 5x^2 - x + 4 and F(x) as 2x - 1. The composition F(G(x)) simplifies to 10x^2 - 2x + 7.

What is the third example of function composition in the script?

In the third example, F(x) = 2x - 15, G(x) = x^2 + 19x + 90. The composition G(F(x)) simplifies to 4x^2 - 22x + 30.

What is the purpose of the fourth example in the script?

The fourth example demonstrates how to find the composition of the function H with G at a specific value, x = -4. It shows the process of substituting the result of G(-4) into H(x) and finding the final value.

What is the result of the composition H(G(-4)) in the fourth example?

The result of the composition H(G(-4)) is 6, after simplifying the expressions and evaluating the square root of the final value.

What is the function F(x) in the last example of the script?

In the last example, the function F(x) is given as x^2 + 5x + 6.

How is the composition F(G(4)) calculated in the last example?

In the last example, after finding G(4) which equals 6, this value is substituted into F(x), resulting in F(6) which simplifies to 72.

What is the main takeaway from the script regarding function composition?

The main takeaway is understanding how to perform function composition by substituting one function into another, and simplifying the resulting expression to obtain a new function or a specific value.