Complex Numbers Add, Subtract, Multiply, Divide
Summary
TLDRThis video script introduces complex numbers, which consist of a real part and an imaginary part, denoted as 'a + bi'. It explains the concept of 'i' as the square root of negative one, and how to perform basic arithmetic operations with complex numbers, such as addition, subtraction, multiplication, and division. The script also covers the importance of using the complex conjugate to eliminate 'i' from the denominator during division, and emphasizes the need to express the result in the standard 'a + bi' form. The explanation includes step-by-step examples to illustrate the process clearly.
Takeaways
- 🔢 A complex number is composed of a real part and an imaginary part, represented in the standard form as 'a + bi'.
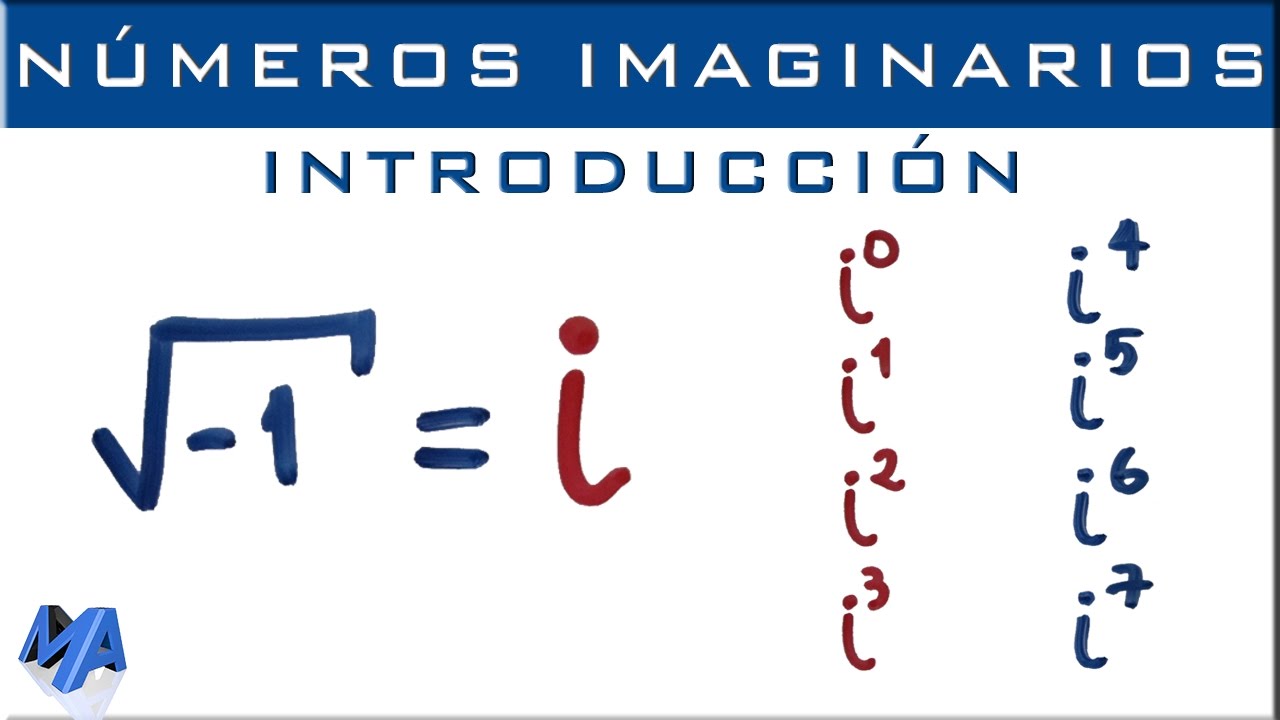
- 📐 The imaginary unit 'i' is defined as the square root of -1, which is denoted by 'i^2 = -1'.
- 🤹 Adding complex numbers involves adding the real parts together and the imaginary parts separately, resulting in a new complex number in 'a + bi' form.
- 🔄 Subtracting complex numbers is similar to addition, but involves subtracting the real and imaginary parts of the second number from the first.
- 🤝 Multiplication of complex numbers uses the FOIL method (First, Outer, Inner, Last), and the result is simplified by recognizing 'i^2 = -1'.
- 🚫 Division by a complex number is not allowed with 'i' in the denominator. It must be rationalized by multiplying by the complex conjugate.
- 🔄 The complex conjugate is used to eliminate 'i' from the denominator by changing the sign of the imaginary part of the denominator.
- 📉 When dividing, both the numerator and the denominator are multiplied by the complex conjugate of the denominator to simplify the expression.
- 📈 After division, the result should be expressed in the standard 'a + bi' form, separating the real and imaginary parts.
- 🔍 An example is given where dividing by a monomial with 'i' in the denominator is simplified by multiplying by 'i/i', resulting in a real number times 'i'.
- 📚 The script provides a comprehensive overview of arithmetic operations with complex numbers, including addition, subtraction, multiplication, and division.
Q & A
What is a complex number?
-A complex number is a number that consists of a real part and an imaginary part, typically written in the form a + bi, where 'i' represents the square root of -1.
What is the significance of 'i' in complex numbers?
-'i' is used to denote the square root of -1. It is the imaginary unit and is fundamental in forming complex numbers.
How do you represent the square root of a negative number?
-The square root of a negative number is represented using 'i'. For example, the square root of -16 is 4i.
What is the result of i squared (i^2)?
-When you square 'i' (i^2), the result is -1, since 'i' is the square root of -1.
How do you add two complex numbers?
-To add two complex numbers, you add their real parts together and their imaginary parts together separately. For example, adding (3 + 2i) and (4 - 5i) results in (7 - 3i).
What is the process for subtracting complex numbers?
-Subtracting complex numbers involves subtracting the real parts and the imaginary parts separately. For instance, (3 + 2i) minus (4 - 5i) equals (-1 + 7i).
How do you multiply complex numbers using the FOIL method?
-When multiplying complex numbers, you use the FOIL method (First, Outer, Inner, Last), multiplying each term in the first binomial by each term in the second, and then combining like terms. For example, (3 + 2i) times (4 - 5i) results in 12 - 15i + 8i - 10i^2, which simplifies to 22 - 7i after considering i^2 = -1.
Why is it necessary to eliminate the imaginary unit from the denominator when dividing complex numbers?
-Having an imaginary unit in the denominator is considered improper. To eliminate it, you multiply both the numerator and the denominator by the complex conjugate, which helps to rationalize the denominator.
What is the complex conjugate and how is it used in division of complex numbers?
-The complex conjugate of a complex number is formed by changing the sign of the imaginary part. It is used to multiply the numerator and denominator to eliminate the imaginary part from the denominator, making it a real number.
How do you simplify the result of dividing two complex numbers?
-After multiplying by the complex conjugate, you simplify the result by performing the multiplication in the numerator and denominator, and then separating the real and imaginary parts into their respective terms, ensuring the result is in the form a + bi.
Can you provide an example of dividing a complex number by a monomial in the denominator?
-Sure, for example, dividing 3 by 2i involves multiplying by i over i, which results in -3/2 times i, or -3/2i. This is because multiplying by i over i is equivalent to multiplying by -1, which simplifies the expression.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados
5.0 / 5 (0 votes)