tripel pythagoras kelas 8 part 2 (mudah) dan menentukan jenis segitiga - Abi Muis Math

Summary

In this educational video, the instructor explains the Pythagorean Theorem, focusing on Pythagorean Triples and how to determine the type of triangle based on known side lengths. The video delves into the concept of Pythagorean Triples—sets of three positive integers that satisfy the theorem—and demonstrates how to calculate and verify these triples. It also covers the classification of triangles into right, acute, and obtuse types based on side length relationships, providing clear examples and problem-solving techniques. The lesson encourages viewers to practice finding Pythagorean Triples and categorizing triangles, with an emphasis on hands-on learning.

Takeaways

• 😀 The Pythagorean theorem relates the sides of a right triangle, where the square of the hypotenuse equals the sum of the squares of the other two sides.
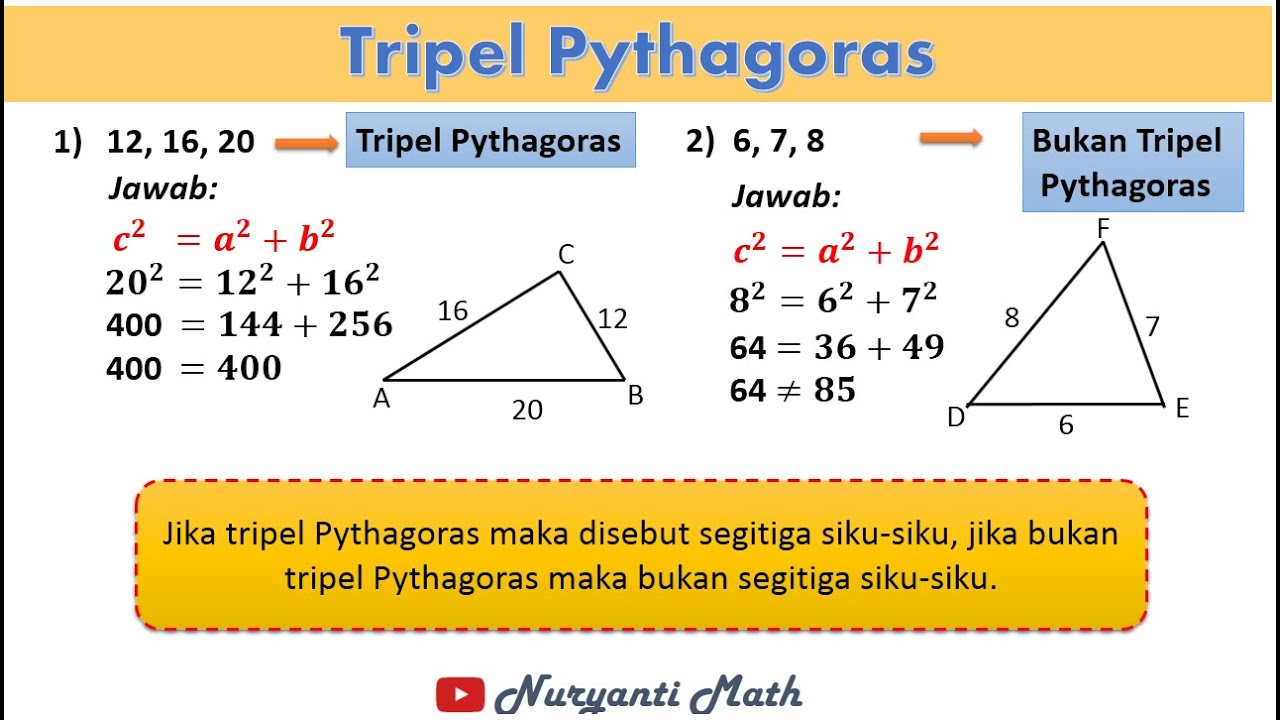
• 😀 Pythagorean triples are sets of three positive integers that satisfy the Pythagorean theorem equation (a^2 + b^2 = c^2).
• 😀 An example of a Pythagorean triple is (3, 4, 5), where 3^2 + 4^2 = 5^2.
• 😀 To find Pythagorean triples, the formula 2pq, p^2 - q^2, and p^2 + q^2 can be used, where p and q are positive integers with p > q.
• 😀 If a set of numbers is a Pythagorean triple, the numbers can be multiples of each other, making them scaled versions of the original triple.
• 😀 An example problem asks whether the set of numbers (9, 12, 15) forms a Pythagorean triple. It does, as 9^2 + 12^2 = 15^2.
• 😀 If a set of numbers does not satisfy the Pythagorean theorem, like (6, 10, 12), it is not a Pythagorean triple.
• 😀 The video explains how to determine the type of triangle (right, acute, or obtuse) based on the lengths of its sides.
• 😀 A triangle is classified as a right triangle if a^2 + b^2 = c^2, where c is the longest side.
• 😀 If a^2 + b^2 < c^2, the triangle is obtuse, while if a^2 + b^2 > c^2, the triangle is acute.
• 😀 The video encourages practice with Pythagorean triples and classifying triangles, and reminds viewers to like, comment, and share the content.

Q & A

What is a Pythagorean Triple?

A Pythagorean Triple is a set of three positive integers (a, b, c) that satisfy the equation a² + b² = c². These integers represent the sides of a right-angled triangle, where 'c' is the hypotenuse (the longest side).

How do we find a Pythagorean Triple using integers p and q?

To find a Pythagorean Triple using two integers p and q, where p > q, you can use the following formulas: a = p² - q², b = 2pq, and c = p² + q². This will give you a Pythagorean Triple.

What does the Pythagorean Theorem state?

The Pythagorean Theorem states that in a right-angled triangle, the square of the length of the hypotenuse (c) is equal to the sum of the squares of the lengths of the other two sides (a and b). The formula is: a² + b² = c².

What does it mean if a set of numbers is a multiple of a Pythagorean Triple?

If a set of numbers is a multiple of a Pythagorean Triple, it means that all the numbers in the set are the result of multiplying the original Pythagorean Triple by a constant factor. These multiples also form a valid Pythagorean Triple.

Can you provide an example of how to determine if a set of numbers forms a Pythagorean Triple?

To determine if a set of numbers forms a Pythagorean Triple, check if the square of the largest number (the hypotenuse) is equal to the sum of the squares of the other two numbers. For example, for the set {9, 12, 15}, check if 9² + 12² equals 15². It does, so {9, 12, 15} is a Pythagorean Triple.

What is the method for determining the type of triangle based on its side lengths?

To determine the type of triangle based on its side lengths, use the Pythagorean Theorem. If c² = a² + b², it is a right triangle. If c² < a² + b², it is an acute triangle, and if c² > a² + b², it is an obtuse triangle.

How do we classify a triangle as acute, right, or obtuse?

A triangle can be classified based on the relationship between the squares of its sides: - If c² = a² + b², it is a right triangle. - If c² < a² + b², it is an acute triangle. - If c² > a² + b², it is an obtuse triangle.

What does it mean if a triangle has an obtuse angle?

A triangle is classified as obtuse if the square of the longest side (c) is greater than the sum of the squares of the other two sides (a² + b²). This means that the angle opposite the longest side is greater than 90°.

What is an example of a triangle with sides 12, 10, and 16? How do we classify it?

For a triangle with sides 12, 10, and 16, calculate the squares of the sides: 16² = 256, 12² + 10² = 144 + 100 = 244. Since 256 > 244, the triangle is classified as obtuse.

What can we conclude if the sum of the squares of the two shorter sides of a triangle is equal to the square of the longest side?

If the sum of the squares of the two shorter sides is equal to the square of the longest side, we can conclude that the triangle is a right triangle.