Pembuktian Teorema Pythagoras 2

Summary

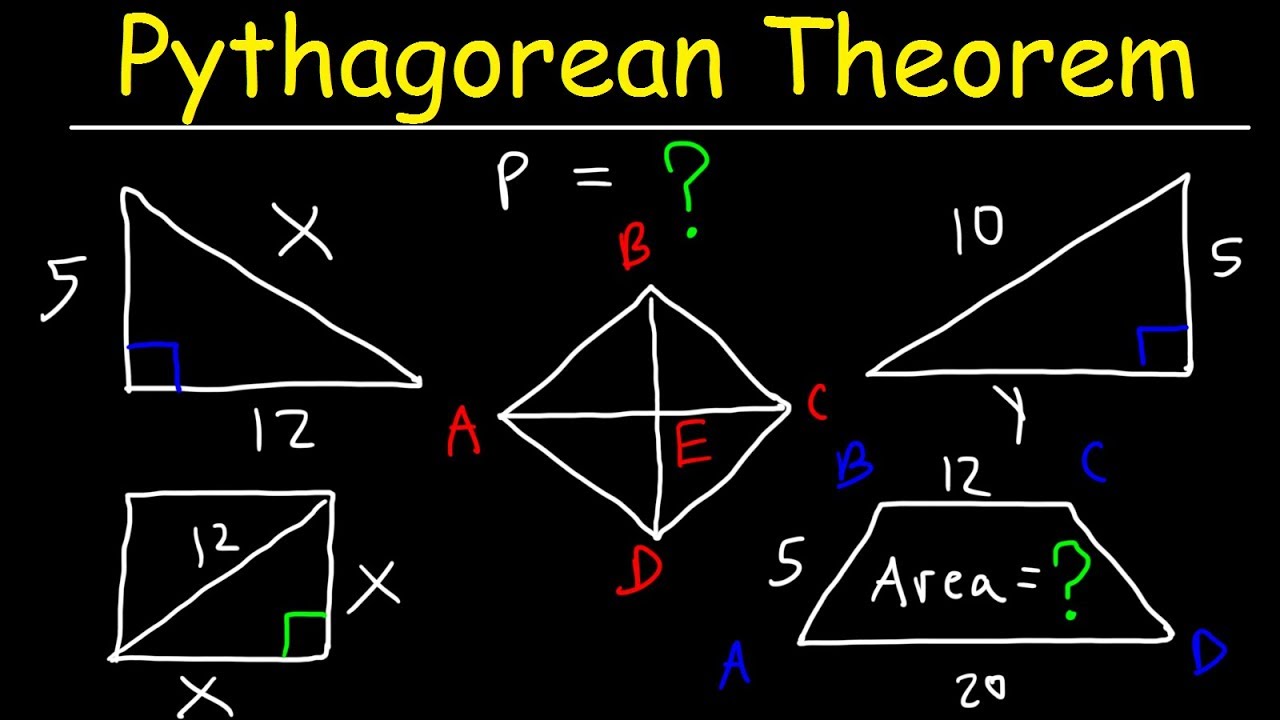
This educational script demonstrates the Pythagorean Theorem using a geometric approach. It begins with constructing a right-angled triangle with specific dimensions (12 cm base, 16 cm height), and uses eight identical triangles to build a visual proof. The process involves creating two symmetrical squares around the triangles, and through careful arrangement and calculation of empty areas, the relationship between the sides of the squares and the triangles is explored. Ultimately, the proof confirms the Pythagorean Theorem (a² + b² = c²), illustrating the geometric properties that lead to this fundamental mathematical result.

Takeaways

• 😀 Start by constructing a right triangle with a base of 12 cm, height of 16 cm, and a hypotenuse of 20 cm.
• 😀 Repeat the process to create 8 identical right triangles.
• 😀 Create two symmetrical squares to act as frames for the triangles, with each square having a side length of 28 cm.
• 😀 Attach the triangles to the first square, positioning four of them along the inner sides.
• 😀 For the second square, place the triangles differently so that they form a rectangle.
• 😀 Label the sides of the right triangle as follows: 'a' for the base, 'b' for the height, and 'c' for the hypotenuse.
• 😀 Prove that the side length of the square is the sum of 'a' and 'b'.
• 😀 Identify empty spaces in the two squares not covered by the triangles.
• 😀 In the first square, the empty space forms a smaller square, labeled as 'C' with a side length of 'c'.
• 😀 In the second square, the empty spaces form two smaller squares, one with a side length of 'a' and the other with 'b'.
• 😀 Conclude that the area of square 'C' is equal to the sum of the areas of squares 'a' and 'b', leading to the proof of the Pythagorean theorem: c^2 = a^2 + b^2.

Q & A

What is the first step in the process described in the script?

The first step is to create a right triangle with a base of 12 cm and a height of 16 cm. The hypotenuse of this triangle will naturally measure 20 cm.

How many right triangles are created in total?

Eight right triangles are created in total, following the same process described in the script.

What is the purpose of the squares mentioned in the second step?

The purpose of the squares is to act as frames for the right triangles. Two symmetric squares are made, and the size of each square is chosen based on the size of the right triangles.

What is the side length of each square?

Each square has a side length of 28 cm.

How are the right triangles placed on the squares?

Four right triangles are placed on the inside of the first square, while the second square has the right triangles placed in a different arrangement, forming a rectangle.

What are the different labels used for the sides of the right triangles?

The sides of the right triangles are labeled as follows: the base is 'a', the height is 'b', and the hypotenuse is 'c'.

How is the side length of the square calculated?

The side length of the square is calculated as the sum of the base and height of the right triangle, i.e., 'a + b'.

What is the shape of the empty spaces left inside the squares?

The empty spaces inside the squares are squares themselves. In the first square, the empty space forms a square labeled 'C', and in the second square, the empty spaces form two smaller squares labeled 'a' and 'b'.

What is the area of the square 'C'?

The area of square 'C' is 'c^2', where 'c' is the hypotenuse of the right triangle.

How does the script prove the Pythagorean Theorem?

The script proves the Pythagorean Theorem by showing that the area of square 'C' is equal to the sum of the areas of squares 'a' and 'b'. This gives the equation 'c^2 = a^2 + b^2', which is the Pythagorean Theorem.