matematika kelas 9 | Transformasi - Refleksi atau pencerminan terhadap sumbu X

Summary

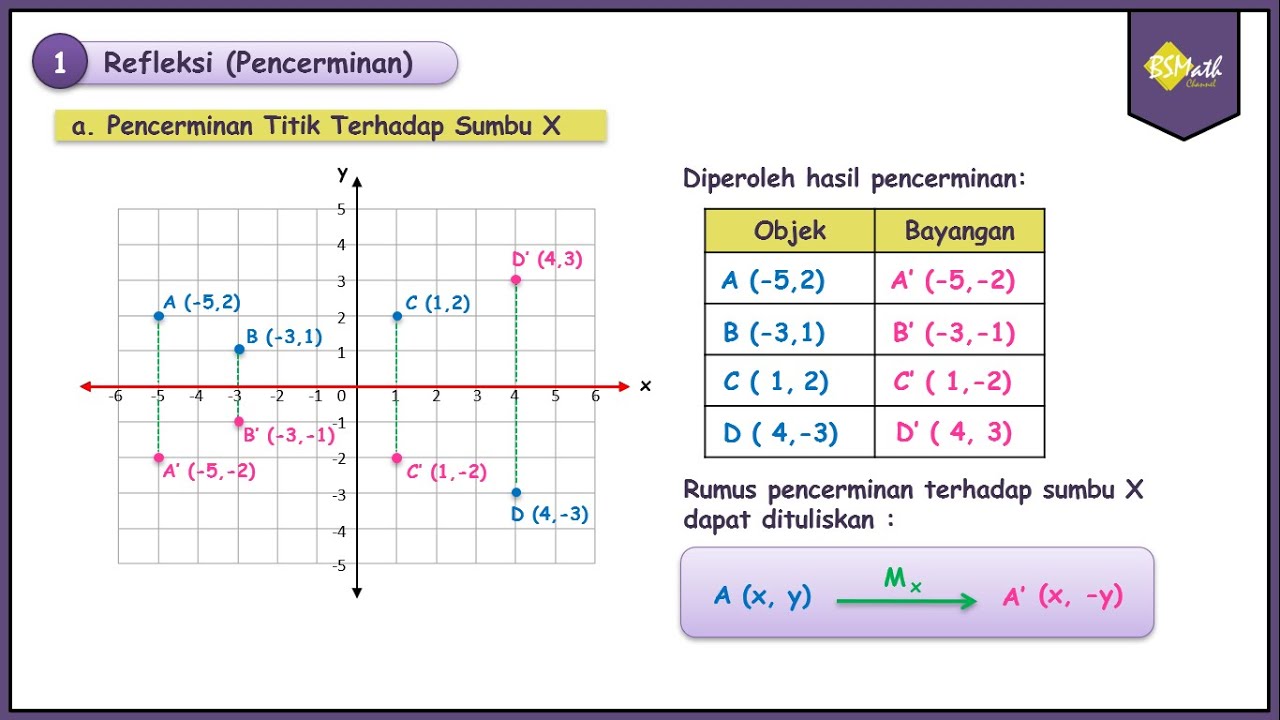
In this video, the concept of reflection in geometry is explored, specifically reflections over the x-axis. The presenter explains how points on the coordinate plane are reflected across the x-axis, using examples to demonstrate the process. For instance, a point A with coordinates (4, 2) is reflected over the x-axis, resulting in its reflection at (4, -2). The video also provides a formula for reflecting points, where the x-coordinate remains the same, and the y-coordinate is negated. With clear visual aids and examples, viewers are encouraged to understand and apply the concept of reflection in their own studies.

Takeaways

• 😀 Reflection is the process of flipping a point over the x-axis in coordinate geometry.
• 😀 When reflecting a point over the x-axis, the x-coordinate remains unchanged, while the y-coordinate changes its sign.
• 😀 The distance between the original point and the x-axis is equal to the distance between the reflected point and the x-axis.
• 😀 The reflection of point A (4, 2) over the x-axis results in point A' (4, -2).
• 😀 The reflection of point B (3, 1) over the x-axis results in point B' (3, -1).
• 😀 The reflection rule can be generalized: for any point (x, y), its reflection over the x-axis will be (x, -y).
• 😀 The instructor suggests visualizing reflections by drawing points on a graph to better understand the concept.
• 😀 To reflect a point, simply invert the sign of the y-coordinate while keeping the x-coordinate the same.
• 😀 The reflection concept applies universally in coordinate geometry for points above and below the x-axis.
• 😀 The video encourages students to subscribe, like, and share the video for further learning and engagement.

Q & A

What is the main topic of this video?

The video focuses on the concept of reflection, specifically reflecting points across the x-axis in a coordinate system.

What does 'refleksi' mean in this context?

'Refleksi' means 'reflection' or 'mirroring' in this context, referring to the transformation of a point's position in relation to the x-axis.

How is a point reflected across the x-axis?

When a point is reflected across the x-axis, the x-coordinate remains the same, while the y-coordinate is negated (multiplied by -1).

What is the reflection of point A (4, 2) across the x-axis?

The reflection of point A (4, 2) across the x-axis is point A' (4, -2). The x-coordinate stays the same, and the y-coordinate becomes negative.

What is the general rule for reflecting a point (x, y) across the x-axis?

The general rule for reflecting a point (x, y) across the x-axis is that the reflected point will be (x, -y).

What is the significance of the distance from a point to the x-axis in this process?

The distance from a point to the x-axis remains the same after the reflection. The original point and its reflected image will be equidistant from the x-axis.

How would the point B (3, 1) be reflected across the x-axis?

Point B (3, 1) would be reflected across the x-axis to point B' (3, -1), with the x-coordinate unchanged and the y-coordinate negated.

What happens to the x-coordinate when reflecting a point across the x-axis?

The x-coordinate remains unchanged when reflecting a point across the x-axis.

Why is the distance between a point and its reflection the same?

The distance is the same because reflection across the x-axis creates a mirror image, where both the original point and its reflection are equally spaced from the axis.

What should you do if you want to reflect a point but are unsure about the coordinates?

You can plot the original point on a coordinate grid, then reflect it by changing the sign of the y-coordinate, ensuring the x-coordinate remains unchanged.