Angular Velocity Introduction
Summary
TLDRIn this educational video, the concept of average velocity is introduced, with a focus on both linear and angular velocities. The formula for average linear velocity is explained as the change in position divided by the change in time. The discussion then shifts to average angular velocity, represented by the Greek letter Omega (ω), and its formula as the change in angular position divided by time. The video also explores common units like radians per second and revolutions per minute (RPM). The instructor emphasizes the importance of understanding angular velocity first without direction, with the right-hand rule to be introduced later.
Takeaways
- 😀 Average velocity is calculated as the change in position divided by the change in time.
- 😀 Average angular velocity is calculated as the change in angular position (theta) divided by the change in time.
- 😀 The symbol for angular velocity is the Greek letter omega (ω), which looks like a curly 'w'.
- 😀 Common units for angular velocity include radians per second (rad/s) and revolutions per minute (RPM).
- 😀 Radians per second are commonly used in physics because radians are dimensionless and easier for calculations.
- 😀 Revolutions per minute (RPM) are typically used in the real world for objects like bike tires, record players, and car engines.
- 😀 Direction is not immediately discussed when introducing angular quantities, but it will be covered later using the right-hand rule.
- 😀 The right-hand rule will replace clockwise and counterclockwise directions, as they are observer-dependent.
- 😀 Examples of objects with angular velocity include bike tires, record players, power drills, and ceiling fans.
- 😀 The equation for average velocity is universally applicable, whether for linear or angular motion, with the primary difference being the type of position (linear vs. angular).
- 😀 Patience is encouraged while learning angular quantities; the direction aspects will be introduced once the core concepts are understood.
Q & A
What is the equation for average velocity?
-The equation for average velocity is the change in position divided by the change in time.
What is the equation for average angular velocity?
-The equation for average angular velocity is the change in angular position (θ) divided by the change in time.
What is the symbol used for angular velocity?
-The symbol for angular velocity is the lowercase Greek letter Omega (Ω).
What units are commonly used for angular velocity?
-The most common units for angular velocity are radians per second (rad/s) and revolutions per minute (RPM).
Why are radians commonly used in physics when measuring angular velocity?
-Radians are preferred in physics because they are dimensionless and serve as a placeholder, making calculations simpler and more standardized.
What real-world objects can have angular velocity?
-Real-world objects that can have angular velocity include a bike tire, a record player, a power drill, a ceiling fan, a car engine, and many others.
What does the right-hand rule refer to in angular motion?
-The right-hand rule is a method used to determine the direction of angular quantities like angular displacement and angular velocity, though it is not yet introduced in this part of the lesson.
What is the main difference between revolutions per minute (RPM) and radians per second?
-Revolutions per minute (RPM) is typically used in the real world to measure the speed of rotating objects, whereas radians per second (rad/s) is the preferred unit in physics because it is more standardized and easier to use in mathematical equations.
Why is the direction of angular quantities not discussed initially in the lesson?
-The direction of angular quantities like angular displacement and angular velocity is not discussed initially to help students first understand the basic concepts without the complexity of direction. Direction will be introduced later using the right-hand rule.
What is the purpose of using the right-hand rule instead of clockwise or counterclockwise directions?
-The right-hand rule is used because clockwise and counterclockwise directions are observer-dependent, and the right-hand rule provides a consistent method for determining direction regardless of the observer's viewpoint.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

Movimento Circular Uniforme (MCU) - Cinemática Escalar - Aula 16 - Prof. Marcelo Boaro
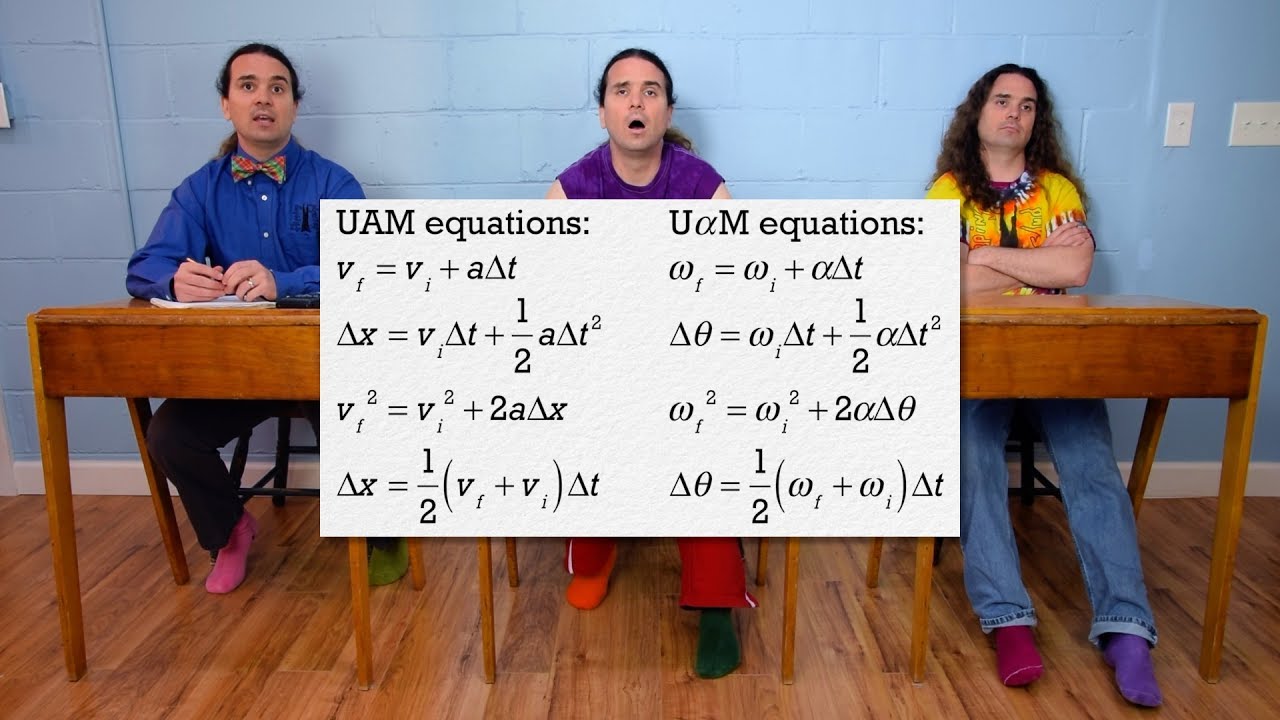
Uniformly Angularly Accelerated Motion Introduction

Kinematika: Menentukan Kecepatan pada Mekanisme 4 Batang dengan Menggunakan Metode Kecepatan Relatif

Movimento Circular Uniforme - Aula 01

L18 - Dyanamics - Lesson 18 - Rigid Body Kinematics
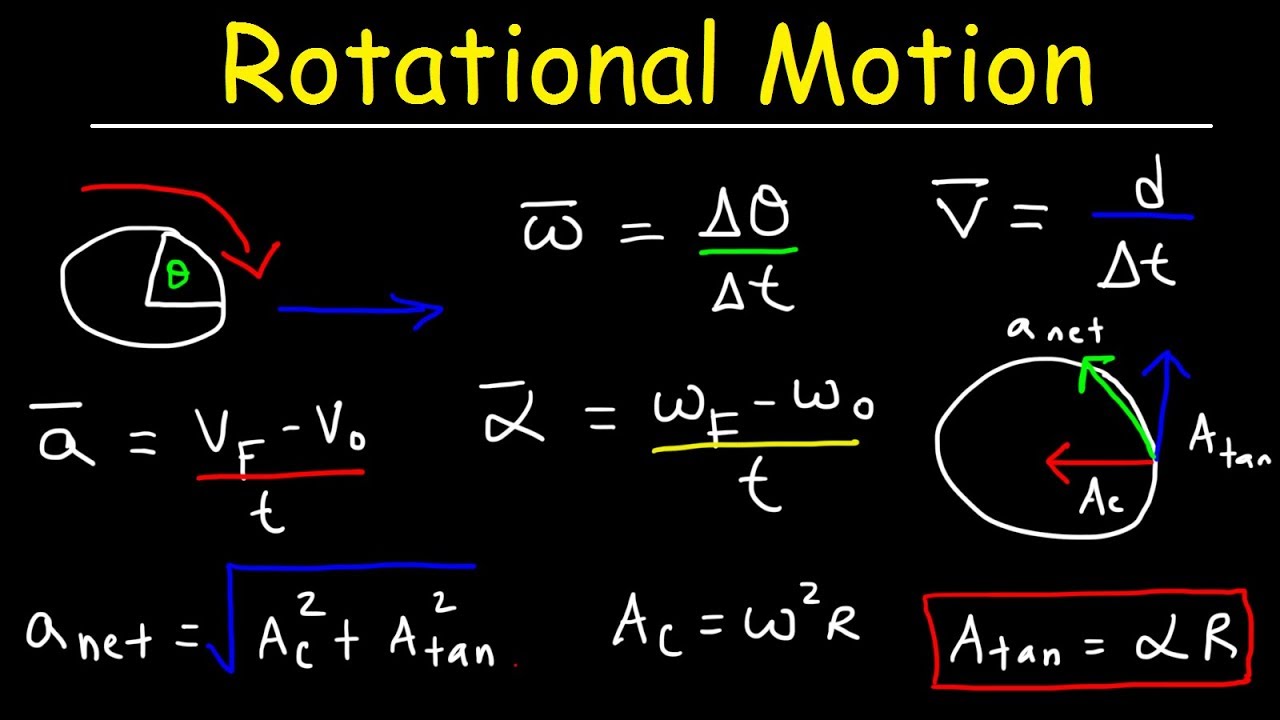
Rotational Motion Physics, Basic Introduction, Angular Velocity & Tangential Acceleration
5.0 / 5 (0 votes)
